Fiber reinforced composites have been used in many engineering areas especially in aerospace industry. A main drawback in the use of composite materials is that their mechanical properties due to the influence of anisotropy have to be determined by difficult or expensive experiments. Micromechanics is a theory to approach the effective properties of a composite from its constituent information, which significantly reduces the demands on the experiments.
Numerious existing miromechanics models[1-3] are mainly developed for the prediction of a composite stiffness. Failure and strength behavior of the composite is hardly predictable only based on the original constituent data with a reasonable accuracy, according to Ref.[4]. By "original", it means that the properties are either measured from monolithic material, for instance, matrix, specimens or ones documented in a recognized material database. Very recently, we have found that the homogenized stresses in the matrix of a composite determined by a micromechanics theory must be converted into true values before a failure assessment can be made against the original constituent properties[5-11]. The conversion is achieved by multiplying the homogenized quantities with corresponding stress concentration factors (SCFs) of a matrix in the composite. Such an SCF cannot be defined following a classical approach. Explicit formulae for the SCFs under various loads have been obtained[5-11], making a reasonable prediction for a composite strength achievable only by using the original constituent properties.
A critical assessment for the predictability of 14 well-known micromechanics models for the stiffness and more importantly for the strength of a UD composite is made in this paper. Previous comparisons, such as by Refs. [12-14], were made only for predicting stiffness by different models. Few has been found for the strength prediction. The models included in this paper are Eshelby's method[15], Bridging Model16], Mori-Tanaka method[17-18], rule of mixture method[19], Chamis model[20], modified rule of mixture method[19], Halpin-Tsai formulae[21], Hill-Hashin-Christensen-Lo model[22], self-consistent method[15], generalized self-consistent method[15, 23], double inclusion method[3], generalized method of cells (GMC)[24], generalized finite volume method (GFVM)[25-26], and finite element method (FEM)[27]. The measured stiffness and strength data of all the 9 independent UD composites in three WWFEs were used as benchmark to judge the prediction accuracy of each model. An accuracy ranking was made based on the overall correlation error between the predictions and the experiments. On the whole, Bridging Model exhibited the highest prediction accuracy in both stiffness and strength for the composites.
As solution of a numerical micromechanics method, GMC, GFVM, and FEM, depend on an RVE geometry to be discretized, different fiber arrangement patterns in the RVE have been chosen for the FEM solution. Many people deemed that a sufficient number of fibers arranged in a random pattern should be taken for an RVE[28-30], but this is not supported by our study. Among the four patterns, i.e. the square, hexagonal, square-diagonal, and the random fiber arrays, the smallest fiber volume or the square fiber array in the RVE resulted in the highest simulation accuracy. The conclusion is, in fact, easy to understand. By definition, the volume of an RVE, on which a homogenization is made, should be infinitesimal. Though in reality an RVE has to be finite, the smallest volume should lead to the best approximation, providing that all of the other conditions remain unchanged.
Finally, consistency is an issue that should be taken into account especially when a numerical micromechanics model is applied to simulate a composite property. Any model results in two sets of formulae, i.e. 2D and 3D formulae, for the internal stresses in the fiber and matrix. When the composite is subjected to a plane load, either the 2D or the 3D formulae can be applied to calculate the internal stresses. If the stress components by the 2D and the 3D formulae are exactly the same, the model is said to be consistent in the internal stress calculation. Among the 14 theories considered, only Bridging Model is consistent in this respect, implying that the full 3D approach of any other model investigated in this paper should be used to achieve the highest accuracy.
2 Internal Stress DeterminationA composite is heterogeneous by nature. Any stress (as well as strain) should be defined based on averaged quantities with respect to its RVE of a volume V' through
| $ {\sigma _i} = \left( {\int\limits_{V'} {{{\tilde \sigma }_i}{\rm{d}}V} } \right)/V' = {V_f}\sigma _i^f + {V_m}\sigma _i^m $ | (1) |
It must be noted that by definition V' is infinitesimal. A resulting stress, with "~" on top, represents a point-wise quantity. If V' is finite, the corresponding stress is called a homogenized one. In Eq. (1), V is a volume fraction with Vf+Vm=1. A super-/sub-script f or m refers to the fiber or matrix, whereas a quantity without any suffix stands for the composite.
Using a bridging equation, {σim}= [Aij]{σjf}, the internal stresses in the fiber and matrix together with the compliance tensor of the composite are found to be[16]
| $ \left\{ {\sigma _i^f} \right\} = {\left( {{V_f}\left[ I \right] + {V_m}\left[ {{A_{ij}}} \right]} \right)^{ - 1}}\left\{ {{\sigma _j}} \right\} $ | (2) |
| $ \left\{ {\sigma _i^m} \right\} = \left[ {{A_{ij}}} \right]{\left( {{V_f}\left[ I \right] + {V_m}\left[ {{A_{ij}}} \right]} \right)^{ - 1}}\left\{ {{\sigma _j}} \right\} $ | (3) |
| $ \begin{array}{l} \left[ {{S_{ij}}} \right] = \left( {{V_f}\left[ {S_{ij}^f} \right] + {V_m}\left[ {S_{ij}^m} \right]\left[ {{A_{ij}}} \right]} \right)\left( {{V_f}\left[ I \right] + } \right.\\ \;\;\;\;\;\;\;\;\;\;{\left. {{V_m}\left[ {{A_{ij}}} \right]} \right)^{ - 1}} \end{array} $ | (4) |
where [Sijf] and [Sijm] are the compliance tensors of the fiber and matrix, respectively, and [I] is a unit tensor. From Eq.(4), the bridging tensor is solved as
| $ \left[ {{A_{ij}}} \right] = {V_f}{\left( {\left[ {{S_{ij}}} \right] - \left[ {S_{ij}^m} \right]} \right)^{ - 1}}\left( {\left[ {S_{ij}^f} \right] - \left[ {{S_{ij}}} \right]} \right)/{V_m} $ | (5) |
Thus, the prediction of the elastic moduli is equivalent to the calculation of the internal stresses in the fiber and matrix of the same composite.
3 Summary on Micromechanics ModelsFor the purpose of completeness, all the micromechanics models considered in this paper, except for the double inclusion method which has been incorporated into Digimat[31], are briefly summarized as follows.
3.1 Eshelby's MethodThe stiffness tensor of the composite by this method is given by[15]
| $ \begin{array}{l} \left[ {{K_{ij}}} \right] = {\left[ {{S_{ij}}} \right]^{ - 1}} = \left[ {K_{ij}^m} \right] + {V_f}\left( {\left[ {K_{ij}^f} \right] - } \right.\\ \;\;\;\;\;\;\;\;\;\;\;\left. {\left[ {K_{ij}^m} \right]} \right)\left\{ {\left[ I \right] + \left[ {{L_{ij}}} \right]\left[ {S_{ij}^m} \right]} \right.\left( {\left[ {K_{ij}^f} \right] - } \right.\\ \;\;\;\;\;\;\;\;\;\;\;{\left. {\left. {\left[ {K_{ij}^m} \right]} \right)} \right\}^{ - 1}} \end{array} $ | (6) |
where [Kijf] and [Kijm] are the stiffness tensors of the fiber and matrix, and [Lij] is Eshelby's tensor which reads[16]
| $ \left[ {{L_{ij}}} \right] = \left[ {\begin{array}{*{20}{c}} 0&0&0&0&0&0\\ {{L_{2211}}}&{{L_{2222}}}&{{L_{2233}}}&0&0&0\\ {{L_{3311}}}&{{L_{3322}}}&{{L_{3333}}}&0&0&0\\ 0&0&0&{2{L_{2323}}}&0&0\\ 0&0&0&0&{2{L_{1313}}}&0\\ 0&0&0&0&0&{2{L_{1212}}} \end{array}} \right] $ | (7.1) |
| $ {L_{2211}} = {L_{3311}} = \frac{{{\nu ^m}}}{{2\left( {1 - {\nu ^m}} \right)}} $ | (7.2.1) |
| $ {L_{2222}} = {L_{3333}} = \frac{1}{{2\left( {1 - {\nu ^m}} \right)}}\left[ {\frac{3}{4} + \frac{{1 - 2{\nu ^m}}}{2}} \right] $ | (7.2.2) |
| $ {L_{1212}} = {L_{1313}} = 1/4 $ | (7.2.3) |
| $ {L_{2233}} = {L_{3322}} = \frac{1}{{2\left( {1 - {\nu ^m}} \right)}}\left[ {\frac{1}{4} - \frac{{1 - 2{\nu ^m}}}{2}} \right] $ | (7.2.4) |
| $ {L_{2323}} = \frac{1}{{2\left( {1 - {\nu ^m}} \right)}}\left[ {\frac{1}{4} + \frac{{1 - 2{\nu ^m}}}{2}} \right] $ | (7.2.5) |
where νm is Poisson's ratio of the matrix.
3.2 Bridging ModelThe homogenized internal stresses by Bridging Model are expressed as[16]
| $ \sigma _{11}^f = \frac{{\sigma _{11}^0}}{{{V_f} + {V_m}{a_{11}}}} - \frac{{{V_m}{a_{12}}\left( {\sigma _{22}^0 + \sigma _{33}^0} \right)}}{{\left( {{V_f} + {V_m}{a_{11}}} \right)\left( {{V_f} + {V_m}{a_{22}}} \right)}} $ | (8.1) |
| $ \sigma _{11}^m = \frac{{{a_{11}}\sigma _{11}^0}}{{{V_f} + {V_m}{a_{11}}}} + \frac{{{V_f}{a_{12}}\left( {\sigma _{22}^0 + \sigma _{33}^0} \right)}}{{\left( {{V_f} + {V_m}{a_{11}}} \right)\left( {{V_f} + {V_m}{a_{22}}} \right)}} $ | (8.2) |
| $ \begin{array}{l} \sigma _{ij}^f = \frac{{\sigma _{ij}^0}}{{{V_f} + {V_m}{a_{22}}}},\sigma _{ij}^m = \frac{{{a_{22}}\sigma _{ij}^0}}{{{V_f} + {V_m}{a_{22}}}},\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;ij = 22,33,{\rm{and}}\;{\rm{23}} \end{array} $ | (8.3, 8.4) |
| $ \begin{array}{l} \sigma _{ij}^f = \frac{{\sigma _{ij}^0}}{{{V_f} + {V_m}{a_{66}}}},\sigma _{ij}^m = \frac{{{a_{66}}\sigma _{ij}^0}}{{{V_f} + {V_m}{a_{66}}}},\\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;ij = 12\;{\rm{and}}\;{\rm{13}} \end{array} $ | (8.5, 8.6) |
| $ {a_{11}} = {E^m}/E_{11}^f $ | (9.1) |
| $ {a_{12}} = {a_{13}} = \frac{{{\nu ^m}E_{11}^f - {E^m}\nu _{12}^f}}{{{E^m} - E_{11}^f}}\left( {{a_{11}} - {a_{22}}} \right) $ | (9.2) |
| $ {a_{22}} = {a_{33}} = {a_{44}} = 0.3 + 0.7\frac{{{E^m}}}{{E_{22}^f}} $ | (9.3) |
| $ {a_{55}} = {a_{66}} = 0.3 + 0.7\frac{{{G^m}}}{{G_{12}^f}} $ | (9.4) |
where E11f, E22f and G12f are longitudinal, transverse, and in-plane shear modulus of the fiber, respectively. ν12f is its longitudinal Poisson's ratio. Em and Gm are Young's and shear moduli of the matrix. {σ110, σ220, σ330, σ230, σ130, σ120} are arbitrary loads applied on the composite.
3.3 Mori-Tanaka MethodNon-zero bridging tensor elements of Mori-Tanaka method are given below[32]:
| $ {A_{11}} = \frac{{{E^m}}}{{E_{11}^f}}\left( {1 + \frac{{{\nu ^m}\left( {{\nu ^m} - \nu _{12}^f} \right)}}{{\left( {1 + {\nu ^m}} \right)\left( {1 - {\nu ^m}} \right)}}} \right) $ | (10.1) |
| $ \begin{array}{l} {A_{12}} = \frac{{{E^m}}}{{E_{22}^f}}\frac{{{\nu ^m}\left( {1 - \nu _{23}^f} \right)}}{{2\left( {1 + {\nu ^m}} \right)\left( {1 - {\nu ^m}} \right)}} - \\ \;\;\;\;\;\;\;\;\frac{{{E^m}}}{{E_{11}^f}}\frac{{\nu _{12}^f}}{{\left( {1 + {\nu ^m}} \right)\left( {1 - {\nu ^m}} \right)}} + \\ \;\;\;\;\;\;\;\;\;\;\;\;\frac{{{\nu ^m}}}{{2\left( {1 - {\nu ^m}} \right)}} = {A_{13}} \end{array} $ | (10.2) |
| $ {A_{21}} = \frac{{{E^m}}}{{E_{11}^f}}\frac{{{\nu ^m} - \nu _{12}^f}}{{2\left( {1 + {\nu ^m}} \right)\left( {1 - {\nu ^m}} \right)}} = {A_{31}} $ | (10.3) |
| $ \begin{array}{l} {A_{22}} = \frac{{{E^m}}}{{E_{22}^f}}\frac{{\left( {\nu _{23}^f - 3} \right)}}{{8\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} + \\ \;\;\;\;\;\;\;\;\frac{{{E^m}}}{{E_{11}^f}}\frac{{\nu _{12}^f{\nu ^m}}}{{2\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} + \\ \;\;\;\;\;\;\;\;\frac{{\left( {{\nu ^m} + 1} \right)\left( {4{\nu ^m} - 5} \right)}}{{8\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} = {A_{33}} \end{array} $ | (10.4) |
| $ \begin{array}{l} {A_{32}} = \frac{{{E^m}}}{{E_{22}^f}}\frac{{\left( {3\nu _{23}^f - 1} \right)}}{{8\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} + \\ \;\;\;\;\;\;\;\;\frac{{{E^m}}}{{E_{11}^f}}\frac{{\nu _{12}^f{\nu ^m}}}{{2\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} + \\ \;\;\;\;\;\;\;\;\frac{{\left( {{\nu ^m} + 1} \right)\left( {1 - 4{\nu ^m}} \right)}}{{8\left( {{\nu ^m} - 1} \right)\left( {{\nu ^m} + 1} \right)}} = {A_{23}} \end{array} $ | (10.5) |
| $ {A_{44}} = \frac{{{G^m}}}{{G_{23}^f}}\frac{1}{{4\left( {1 - {\nu ^m}} \right)}} + \frac{{\left( {3 - 4{\nu ^m}} \right)}}{{4\left( {1 - {\nu ^m}} \right)}} $ | (10.6) |
| $ {A_{55}} = \frac{{{G^m} + G_{12}^f}}{{2G_{12}^f}} = {A_{66}} $ | (10.7) |
where G23f is the fiber transverse shear modulus.
3.4 Rule of Mixture ModelFive elastic moduli of the composite defined by the rule of mixture model are[19]
| $ {E_{11}} = VE_{11}^f + {V_m}{E^m} $ | (11.1) |
| $ {\nu _{12}} = {V_f}\nu _{12}^f + {V_m}{\nu ^m} $ | (11.2) |
| $ {E_{22}} = \frac{{{E^m}}}{{1 - {V_f}\left( {1 - \frac{{{E^m}}}{{E_{22}^f}}} \right)}} $ | (11.3) |
| $ {G_{12}} = {G_{13}} = \frac{{{G^m}}}{{1 - {V_f}\left( {1 - \frac{{{G^m}}}{{G_{12}^f}}} \right)}} $ | (11.4) |
| $ {G_{23}} = \frac{{{G^m}}}{{1 - {V_f}\left( {1 - \frac{{{G^m}}}{{G_{23}^f}}} \right)}} $ | (11.5) |
| $ {E_{11}} = VE_{11}^f + {V_m}{E^m} $ | (12.1) |
| $ {\nu _{12}} = {V_f}\nu _{12}^f + {V_m}{\nu ^m} $ | (12.2) |
| $ {E_{22}} = {E_{33}} = \frac{{{E^m}}}{{1 - \sqrt {{V_f}} \left( {1 - \frac{{{E^m}}}{{E_{22}^f}}} \right)}} $ | (12.3) |
| $ {G_{12}} = {G_{13}} = \frac{{{G^m}}}{{1 - \sqrt {{V_f}} \left( {1 - \frac{{{G^m}}}{{G_{12}^f}}} \right)}} $ | (12.4) |
| $ {G_{23}} = \frac{{{G^m}}}{{1 - \sqrt {{V_f}} \left( {1 - \frac{{{G^m}}}{{G_{12}^f}}} \right)}} $ | (12.5) |
| $ {E_{11}} = {V_f}E_{11}^f + {V_m}{E^m} $ | (13.1) |
| $ {\nu _{12}} = {V_f}\nu _{12}^f + {V_m}{\nu ^m} $ | (13.2) |
| $ \begin{array}{l} {E_{22}} = \frac{{4{\eta _{22}}{G_{23}}}}{{{\eta _{22}} + m{G_{23}}}},m = 1 + \frac{{4{\eta _{22}}\nu _{12}^2}}{{{E_{11}}}},\\ \frac{1}{{{\eta _{22}}}} = \frac{1}{{{V_f} + {\eta _k}{V_m}}}\left( {\frac{{{V_f}}}{{\Lambda _{22}^f}} + \frac{{{V_m}{\eta _k}}}{{{\Lambda ^m}}}} \right) \end{array} $ | (13.3) |
| $ \begin{array}{*{20}{c}} {\frac{1}{{{G_{12}}}} = \frac{1}{{{V_f} + {\eta _{12}}{V_m}}}\left( {\frac{{{V_f}}}{{G_{22}^f}} + \frac{{{V_m}{\eta _k}}}{{{G^m}}}} \right),}\\ {{\eta _{12}} = \frac{1}{2}\left( {1 + \frac{{{G^m}}}{{G_{12}^f}}} \right)} \end{array} $ | (13.4) |
| $ \begin{array}{l} \frac{1}{{{G_{23}}}} = \frac{1}{{{V_f} + {\eta _{23}}{V_m}}}\left( {\frac{{{V_f}}}{{G_{23}^f}} + \frac{{{V_m}{\eta _{23}}}}{{{G^m}}}} \right),\\ {\eta _{23}} = \frac{1}{{4\left( {1 - {\nu ^m}} \right)}}\left( {3 - 4{\nu ^m} + \frac{{{G^m}}}{{G_{23}^f}}} \right) \end{array} $ | (13.5) |
| $ {\eta _k} = \frac{1}{{2\left( {1 - {\nu ^m}} \right)}}\left( {1 + \frac{{{\Lambda ^m}}}{{\Lambda _{22}^f}}} \right) $ | (13.6) |
| $ \begin{array}{*{20}{c}} {\Lambda _{22}^f = 0.5\left( {K_{22}^f + K_{23}^f} \right),}\\ {{\Lambda ^m} = 0.5\left( {K_{22}^m + K_{23}^m} \right)} \end{array} $ | (13.7) |
where Kijf and Kijm are the stiffness elements of the fiber and matrix.
3.7 Halpin-Tsai's Formulae[21]| $ {E_{11}} = {V_f}E_{11}^f + {V_m}{E^m} $ | (14.1) |
| $ {\nu _{12}} = {V_f}\nu _{12}^f + {V_m}{\nu ^m} $ | (14.2) |
| $ \begin{array}{*{20}{c}} {{E_{22}} = \frac{{4{\Lambda _L}{G_{23}}}}{{{\Lambda _L} + m{G_{23}}}},m = 1 + \frac{{4{\Lambda _L}\nu _{12}^2}}{{{E_{11}}}},}\\ {\frac{{{\Lambda _L}}}{{{\Lambda ^m}}} = \frac{{1 + \left( {1 - 2{\nu ^m}} \right)\eta {V_f}}}{{1 - \eta {V_f}}}} \end{array} $ | (14.3) |
| $ \begin{array}{*{20}{c}} {\frac{1}{{{G_{12}}}} = \frac{1}{{{V_f} + {\eta _{12}}{V_m}}}\left( {\frac{{{V_f}}}{{G_{22}^f}} + \frac{{{V_m}{\eta _12}}}{{{G^m}}}} \right),}\\ {{\eta _{12}} = \frac{1}{2}\left( {1 + \frac{{{G^m}}}{{G_{12}^f}}} \right)} \end{array} $ | (14.4) |
| $ \begin{array}{l} \frac{1}{{{G_{23}}}} = \frac{1}{{{V_f} + {\eta _{23}}{V_m}}}\left( {\frac{{{V_f}}}{{G_{23}^f}} + \frac{{{V_m}{\eta _{23}}}}{{{G^m}}}} \right),\\ {\eta _{23}} = \frac{1}{{4\left( {1 - {\nu ^m}} \right)}}\left( {3 - 4{\nu ^m} + \frac{{{G^m}}}{{G_{23}^f}}} \right) \end{array} $ | (14.5) |
| $ \eta = \frac{{\Lambda _{22}^f/{\Lambda ^m} - 1}}{{\Lambda _{22}^f/{\Lambda ^m} + 1 - 2{\nu ^m}}} $ | (14.6) |
| $ \begin{array}{l} {E_{11}} = {V_f}E_{11}^f + {V_m}{E^m} + \\ \;\;\;\;\;\;\;\;\frac{{4{{\left( {\nu _{12}^f - {\nu ^m}} \right)}^2}{V_f}\left( {1 - {V_f}} \right)}}{{\frac{{{V_f}}}{{{\Lambda ^m}}} + \frac{{1 - {V_f}}}{{\Lambda _{22}^f}} + \frac{1}{{{G^m}}}}} \end{array} $ | (15.1) |
| $ \begin{array}{l} {\nu _{12}} = {V_f}\nu _{12}^f + {V_m}{\nu ^m} + \\ \;\;\;\;\;\;\;\;\;\;\frac{{{{\left( {\nu _{12}^f - {\nu ^m}} \right)}^2}{V_f}\left( {1 - {V_f}} \right)}}{{\frac{{{V_f}}}{{{\Lambda ^m}}} + \frac{{1 - {V_f}}}{{\Lambda _{22}^f}} + \frac{1}{{{G^m}}}}}\left( {\frac{1}{{{\Lambda ^m}}} - \frac{1}{{\Lambda _{22}^f}}} \right) \end{array} $ | (15.2) |
| $ {E_{22}} = \frac{2}{{0.5/{K_T} + 0.5/{G_{23}} + 2\nu _{12}^2/{E_{11}}}} $ | (15.3) |
| $ {G_{12}} = {G^m}\frac{{\left( {G_{12}^f + {G^m}} \right) + {V_f}\left( {G_{12}^f - {G^m}} \right)}}{{\left( {G_{12}^f + {G^m}} \right) - {V_f}\left( {G_{12}^f - {G^m}} \right)}} $ | (15.4) |
| $ {K_T} = {K^m} + \frac{{{V_f}}}{{\frac{1}{{\Lambda _{22}^f - {\Lambda ^m}}} + \frac{{1 - {V_f}}}{{{\Lambda ^m} + {G^m}}}}} $ | (15.5) |
| $ {A_1}{\left( {\frac{{{G_{23}}}}{{{G^m}}}} \right)^2} + 2{B_1}\left( {\frac{{{G_{23}}}}{{{G^m}}}} \right) + C = 0 $ | (15.6) |
| $ \begin{array}{l} {A_1} = 3{V_f}V_m^2\left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right)\left( {\frac{{{G_f}}}{{{G^m}}} + {\eta _f}} \right) + \\ \;\;\;\;\;\;\;\left[ {\frac{{{G_f}}}{{{G^m}}}{\eta _m} + {\eta _f}{\eta _m} - \left( {\frac{{{G_f}}}{{{G^m}}}{\eta _m} - {\eta _f}} \right)V_f^3} \right] \times \\ \;\;\;\;\;\;\;\left[ {{V_f}{\eta _m}\left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right) - \left( {\frac{{{G_f}}}{{{G^m}}}{\eta _m} + 1} \right)} \right] \end{array} $ | (15.7) |
| $ \begin{array}{l} {B_1} = - 3{V_f}V_m^2\left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right)\left( {\frac{{{G_f}}}{{{G^m}}} + {\eta _f}} \right) + \\ \;\;\;\;\;\;\;\frac{1}{2}\left[ {\frac{{{G_f}}}{{{G^m}}}{\eta _m} + \left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right){V_f} + 1} \right]\\ \;\;\;\;\;\;\;\left[ {\left( {{\eta _m} - 1} \right)\left( {\frac{{{G_f}}}{{{G^m}}} + {\eta _f}} \right) - } \right.\\ \;\;\;\;\;\;\;\left. {2\left( {\frac{{{G_f}}}{{{G^m}}}{\eta _m} - {\eta _f}} \right)V_f^3} \right] + \frac{{{V_f}}}{2}\left( {{\eta _m} + 1} \right)\left( {\frac{{{G_f}}}{{{G^m}}} - } \right.\\ \;\;\;\;\;\;\;\left. 1 \right)\left[ {\frac{{{G_f}}}{{{G^m}}} + {\eta _f} + \left( {\frac{{{G_f}}}{{{G^m}}}{\eta _m} - {\eta _f}} \right)V_f^3} \right] \end{array} $ | (15.8) |
| $ \begin{array}{l} C = 3{V_f}V_m^2\left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right)\left( {\frac{{{G_f}}}{{{G^m}}} + {\eta _f}} \right) + \\ \;\;\;\;\;\;\;\;\left[ {\frac{{{G_f}}}{{{G^m}}}{\eta _m} + {V_f}\left( {\frac{{{G_f}}}{{{G^m}}} - 1} \right) + 1} \right] \times \\ \;\;\;\;\;\;\;\;\left[ {\frac{{{G_f}}}{{{G^m}}} + {\eta _f} + \left( {\frac{{{G_f}}}{{{G^m}}}{\eta _m} - {\eta _f}} \right)V_f^3} \right] \end{array} $ | (15.9) |
| $ {\eta _f} = 3 - 4\nu _{23}^f,{\eta _m} = 3 - 4{\nu _m} $ | (15.10) |
It is noted that Eq. (15.6) is applicable only to composites with isotropic fiber reinforcement.
3.9 Self-Consistent MethodThe self-consistent formulae are represented as follows[15]:
| $ \left[ {{K_{ij}}} \right] = \left[ {K_{ij}^m} \right] + {V_f}\left( {\left[ {K_{ij}^f} \right] - \left[ {K_{ij}^m} \right]} \right)\left[ {M_{ij}^f} \right] $ | (16.1) |
| $ \left[ {M_{ij}^f} \right] = {\left\{ {\left[ I \right] + \left[ {{{\tilde L}_{ij}}} \right]\left[ {{S_{ij}}} \right]\left( {\left[ {K_{ij}^f} \right] - \left[ {{K_{ij}}} \right]} \right)} \right\}^{ - 1}} $ | (16.2) |
| $ \begin{array}{l} \left[ {{{\tilde L}_{ij}}} \right] = \left( {\left[ {{S_{ij}}} \right]\left[ {{{\tilde A}_{ij}}} \right]\left[ {K_{ij}^f} \right] - \left[ I \right]} \right)\\ \;\;\;\;\;\;\;\;\;\;\;{\left( {\left[ {K_{ij}^f} \right] - \left[ {{K_{ij}}} \right]} \right)^{ - 1}}\left[ {{K_{ij}}} \right] \end{array} $ | (16.3) |
where [
| $ {{\tilde A}_{11}} = \frac{{{E_{11}}\left( {{E_{11}} - {E_{22}}\nu _{12}^f{\nu _{12}}} \right)}}{{E_{11}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} $ | (17.1) |
| $ \begin{array}{l} {{\tilde A}_{12}} = {{\tilde A}_{13}} = \left\{ {{E_{11}}\left\{ {E_{11}^f{E_{22}}\left( {1 - \nu _{23}^f} \right){\nu _{12}} + } \right.} \right.\\ \;\;\;\;\;\;\;\;\;\left. {\left. {E_{22}^f\left[ { - 2{E_{11}}\nu _{12}^f + E_{11}^f{\nu _{12}}\left( {1 + {\nu _{23}}} \right)} \right]} \right\}} \right\}/\\ \;\;\;\;\;\;\;\;\;\left\{ {2E_{11}^fE_{22}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]} \right\} \end{array} $ | (17.2) |
| $ {{\tilde A}_{21}} = {{\tilde A}_{31}} = \frac{{{E_{11}}{E_{12}}\left( {{\nu _{12}} - \nu _{12}^f} \right)}}{{2E_{11}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} $ | (17.3) |
| $ \begin{array}{l} {{\tilde A}_{22}} = {{\tilde A}_{33}} = \frac{{{E_{11}}\left[ {E_{22}^f\left( {5 + {\nu _{23}}} \right) + {E_{22}}\left( {3 - \nu _{23}^f} \right)} \right]}}{{8E_{22}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} - \\ \;\;\;\;\;\;\;\;\frac{{{E_{22}}{\nu _{12}}\left( {{E_{11}}\nu _{12}^f + E_{11}^f{\nu _{12}}} \right)}}{{2E_{11}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} \end{array} $ | (17.4) |
| $ \begin{array}{l} {{\tilde A}_{23}} = {{\tilde A}_{32}} = \frac{{{E_{11}}\left[ {{E_{22}}\left( {1 - 3\nu _{23}^f} \right) + E_{22}^f\left( {3{\nu _{23}} - 1} \right)} \right]}}{{8E_{22}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} + \\ \;\;\;\;\;\;\;\;\frac{{{E_{22}}{\nu _{12}}\left( {E_{11}^f{\nu _{12}} - {E_{11}}\nu _{12}^f} \right)}}{{2E_{11}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]}} \end{array} $ | (17.5) |
| $ \begin{array}{*{20}{c}} {{{\tilde A}_{44}} = \left\{ {\left( {1 + \nu _{23}^f} \right)\left\{ { - 8{E_{22}}G_{23}^f{{\left( {{\nu _{12}}} \right)}^2} + {E_{11}}\left[ {{E_{22}} + } \right.} \right.} \right.}\\ {\left. {\left. {2G_{23}^f\left( {3 - {\nu _{23}}} \right)} \right]} \right\}/\left\{ {4E_{22}^f\left[ {{E_{11}} - {E_{22}}{{\left( {{\nu _{12}}} \right)}^2}} \right]} \right\}} \end{array} $ | (17.6) |
| $ {{\tilde A}_{55}} = {{\tilde A}_{66}} = \frac{{G_{12}^f + {G_{12}}}}{{2G_{12}^f}} $ | (17.7) |
Different from the preceding 8 explicit models, the self-consistent model is implicit. An iteration has to be carried out to determine the five effective elastic moduli of the composite, E11, E22, G12, ν12, and ν23.
3.10 Generalized Self-Consistent MethodBasic equations of the generalized self-consistent method are the same as Eqs. (16.1)-(16.3), except that the bridging tensor in Eq. (16.3), [
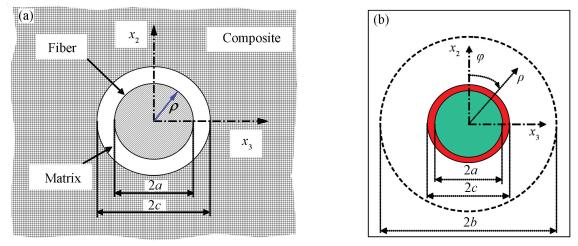
|
Fig.1 (a) Schematic for generalized self-consistent method, (b) Three-phase CCA model (b→∞) for the generalized self-consistent method |
As pointed out in Ref. [34], a three-phase CCA model (Fig. 1) can be sufficiently well approximated with two-step two-phase CCA ones. In the first step, the fiber and matrix constitute a UD composite, whose effective elastic moduli, E11eq, E22eq, ν12eq, G12eq, and ν23eq, can be obtained from Eq. (4) in which the bridging tensor [Aij] is defined by Eqs. (10.1)-(10.7). Then, this UD composite is regarded as an equivalent fiber to be embedded into the composite. The resulting bridging tensor [Aijeq] correlating the stresses of the composite with those of the equivalent fiber is given by Eqs. (17.1)-(17.7), providing that E11f, E22f, ν12f, G12f, and ν23f are replaced by E11eq, E22eq, ν12eq, G12eq, and ν23eq, respectively.
From {σi}=[Aijeq]{σjeq} and {σif}=(Vf[I]+Vm[Aij])-1{σjeq}, it is obtained that
| $ \left[ {{{\tilde A}_{ij}}} \right] \approx \left[ {A_{ij}^{eq}} \right]\left( {{V_f}\left[ I \right] + {V_m}\left[ {{A_{ij}}} \right]} \right) $ | (18) |
The remaining three methods, GMC, GFVM, and FEM, belong to the approach of computational micromechanics, in which discretization on an RVE is necessary. Various selections of the RVE geometry have been used by different researchers. In this paper, the discretization scheme described in Ref.[35] is used. An RVE with a square fiber arrangement is applied to model a UD composite.
4 Assessment on Stiffness PredictionHinton et al.[4] organized three WWFEs to judge efficiency of the current strength theories for composites. A total number of 9 independent material systems were used. Mechanical properties of the fibers and matrices as well as fiber volume fractions of the 9 UD composites were provided[36-38], and are cited in Table 1. Measured effective properties of the composites from the exercise orginazors[36-38], which are used as a banchmark to assess the predicability of the 14 models, are listed in Table 2. Predictions for the five effective elastic moduli of each of the 9 UD composites by the 14 models were made and summarized in Table 3. Relative error of each prediction result in comparison with the measured counterpart (Table 2) was calculated, and the overall averaged errors by the 14 models are given in Table 4. Based on the overall errors, a ranking for the stiffness prediction accuracy is made and indicated in Table 4. It can be seen that Bridging Model exhibits overall the highest accuracy in the stiffness prediction. The second most accurate result was obtained by GFVM, which has a accuracy difference of 24% compared with Bridging Model. The similar conclusion can also be found in Refs. [12-14].
| Table 1 Mechanical properties of the fibers and matrices of the 9 UD composites used in WWFEs[36-38] |
| Table 3 Predicted elastic moduli of the 9 UD composites by different models |
| Table 4 Overall averaged errors in prediction of the elastic moduli of the 9 UD composites by different micromechanics models |
It is acknowledged in the composite community that the prediction of the elasticity of UD composites is already mature. Thus, a significant improvement in the accuracy of both experimental measurement and micromechanics prediction for the elastic properties of a UD composite is not likely achievable. Considering the deviations occurred in the experimental measurement of the fiber, matrix, and composite properties and in light of Table 4, an attainable overall correlation error between the predicted and measured effective elastic moduli of the composite should be around 10%.
5 Different Fiber Arrays in RVEThe results of the three numerical micromechanics methods, GMC, GFVM, and FEM, were obtained based on a square fiber arrangement pattern. As different fiber arrays result in different solutions. the fiber array most suitable for a numerical micromechanics approach need to be determined. A random arrangement pattern with a sufficient number of fibers, such as 25[39], 30[40], 40[41] or even 120[42], in the RVE is most widely applied in the current literature. In this work, a comparison on different fiber arrays was made only for the FEM approach. The fiber and matrix in the RVE are discretized, respectively, into a number of cube elements with the prescribed boundary conditions given in Ref. [43]. The FEM software package ABAQUS was employed to obtain the solutions.
In addition to the square fiber array (Fig. 2(a)), three other fiber arrangement patterns investigated in this paper are hexagonal array[43](Fig. 2(b)), square-diagonal array[44] (Fig. 2(c)), and random array with 30 fibers[28, 45](Fig. 2(d)) involved. Our sample solutions are the same as those in Ref.[43] for Fig. 2(b), Ref.[44] for Fig. 2(c), and Ref.[45] for Fig. 2(d), respectively.
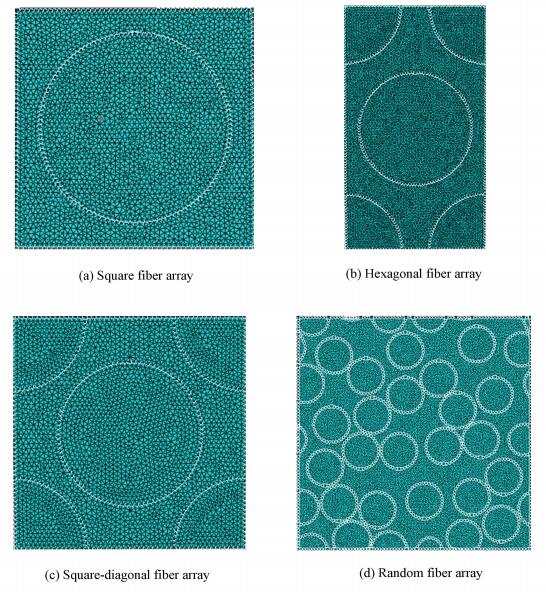
|
Fig.2 Different RVE geometries for a UD composite used in FE approach |
The predicted elastic moduli of the 9 composites using the three other fiber arrays are also shown in Table 3. Note that the solutions by FEM with all of the four fiber arrays, as shown in Figs. 2(a)-(d), are not exactly transversely isotropic, for instance, G13≠G12. However, the departure of the solution on any of the arrays from a transverse isotropy is insignificant. Thus, only the 5 main elastic moduli from the solutions are given in Table 3. The averaged correlation errors between the predictions with the different fiber arrays and the experiments (Table 2) are indicated in Table 4. In the tables, the FE-hexagonal, FE-square diagonal, and FE-random stand for the FEM solutions are based on Figs. 2(b), 2(c), and 2(d), respectively. It is clearly demonstrated that among the four fiber arrays, the square fiber arrangement pattern, which has the smallest RVE volume, has the highest simulation accuracy. Although the FE-random has slightly higher accuracy than the other two fiber arrays, i.e. the FE-hexagonal and FE-square diagonal, it is much less efficient than the FE-square. Hence, the smallest fiber volume in an RVE plays a much more dominant role in achieving the highest simulation accuracy.
6 True Stresses of the Matrix in a Composite 6.1 BackgroundConsider the E-Glass/LY556 UD composite in Table 1 subjected to a transverse tension, σ220. Its ultimate failure is caused by a matrix failure. Using Eqs. (8) and (9), the only non-zero matrix stresses are σ11m=0.134σ220 and σ22m=0.442σ220. Hence, the transverse tensile strength of the composite is given by σ22u, t=Ym/0.422, where Ym is the allowable transverse tensile strength of the matrix. Choosing Ym=σu, tm=80 MPa (Table 2), where σu, tm is the original tensile strength of the matrix, we obtain σ22u, t=181MPa, which is more than 5.2 times greater than 35 MPa, the measured transverse tensile strength of the composite (Table 2).
This is valid almost for any composite, no matter which micromechanics model is used. In other words, the homogenized internal stresses evaluated through Eqs. (2) and (3) must be converted into "true" values before a failure assessment can be made against the original strength data of the constituents. The point-wise stresses in the fiber are uniform[46-47]. Its homogenized and true stresses are the same, while those in the matrix are not. Each of its true stresses is obtained by multiplying the homogenized counterpart with a factor, called an SCF of the matrix in the composite. This is because a plate with a hole subjected to an in-plane tension generates a stress concentration. If the hole is filled with a fiber of different properties, an SCF occurs as well.
6.2 Definition for an SCF of the MatrixThe most significant feature of such an SCF is that it cannot be defined as a point-wise stress of the matrix divided by an overall applied one following a classical approach. Otherwise, the resulting SCF would be infinite if a crack occurs on the fiber and matrix interface, because the matrix stress is singular at the crack tip. The new definition must be made on an averaged stress. An SCF of the matrix subjected to a transverse load is derived through[10]
| $ {K_{22}}\left( \varphi \right) = \frac{1}{{\left| {\mathit{\boldsymbol{\vec R}}_\varphi ^b - \mathit{\boldsymbol{\vec R}}_\mathit{\boldsymbol{\varphi }}^\mathit{\boldsymbol{a}}} \right|}}\int\limits_{\left| {\mathit{\boldsymbol{\vec R}}_\varphi ^a} \right|}^{\left| {\mathit{\boldsymbol{\vec R}}_\varphi ^b} \right|} {\frac{{\tilde \sigma _{22}^m}}{{{{\left( {\sigma _{22}^m} \right)}_{BM}}}}{\rm{d}}\left| {{{\mathit{\boldsymbol{\vec R}}}_\varphi }} \right|} $ | (19) |
where
The transverse tensile, transverse compressive, transverse shear, and longitudinal shear SCFs of the matrix with a perfect fiber and matrix interface bonding have been derived previously. They are summarized below[5, 9, 10]:
| $ \begin{array}{l} K_{22}^t = \left[ {1 + \frac{{\sqrt {{V_f}} }}{2}A + \frac{{\sqrt {{V_f}} }}{2}\left( {3 - {V_f} - \sqrt {{V_f}} } \right)B} \right]\\ \;\;\;\;\;\;\;\;\;\frac{{\left( {{V_f} + 0.3{V_m}} \right)E_{22}^f + 0.7{V_m}{E^m}}}{{0.3E_{22}^f + 0.7{E^m}}} \end{array} $ | (20.1) |
| $ \begin{array}{l} K_{22}^c = \left\{ {1 - \frac{{\sqrt {{V_f}} }}{2}A\frac{{\sigma _{u,c}^m - \sigma _{u,t}^m}}{{2\sigma _{u,c}^m}} + \frac{B}{{2\left( {1 - \sqrt {{V_f}} } \right)}}} \right.\\ \;\;\;\;\;\;\;\;\;\;\;\left[ { - V_f^2\left( {1 - 2{{\left( {\frac{{\sigma _{u,c}^m - \sigma _{u,t}^m}}{{2\sigma _{u,c}^m}}} \right)}^2}} \right) + } \right.\\ \;\;\;\;\;\;\;\;\;\frac{{\left( {\sigma _{u,c}^m + \sigma _{u,t}^m} \right){V_f}}}{{\sigma _{u,c}^m}}\left( {1 + \frac{{\sigma _{u,c}^m - \sigma _{u,t}^m}}{{\sigma _{u,c}^m}}} \right) - \\ \;\;\;\;\;\;\;\;\;\left. {\left. {\sqrt {{V_f}} \left( {\frac{{\sigma _{u,c}^m - \sigma _{u,t}^m}}{{\sigma _{u,c}^m}} + 1 - 2{{\left( {\frac{{\sigma _{u,c}^m - \sigma _{u,t}^m}}{{\sigma _{u,c}^m}}} \right)}^2}} \right)} \right]} \right\} \times \\ \;\;\;\;\;\;\;\;\;\frac{{\left( {{V_f} + 0.3{V_m}} \right)E_{22}^f + 0.7{V_m}{E^m}}}{{0.3E_{22}^f + 0.7{E^m}}} \end{array} $ | (20.2) |
| $ {K_{23}} = 2\sigma _{u,s}^m\sqrt {\frac{{K_{22}^tK_{22}^c}}{{\sigma _{u,t}^m\sigma _{u,c}^m}}} $ | (20.3) |
| $ {K_{12}} = \left[ {1 - {V_f}\frac{{G_{12}^f - {G^m}}}{{G_{12}^f + {G^m}}}\left\{ {W\left( {{V_f}} \right) - \frac{1}{3}} \right\}} \right]\frac{{\left( {{V_f} + {a_{66}}{V_m}} \right)}}{{{a_{66}}}} $ | (20.4) |
| $ W\left( {{V_f}} \right) = {\rm{ \mathsf{ π} }}\sqrt {{V_f}} \left[ {\frac{1}{{4{V_f}}} - \frac{4}{{128}} - \frac{2}{{512}}{V_f} - \frac{5}{{4096}}{V_f}^2} \right] $ | (20.5) |
| $ \begin{array}{l} A = \left\{ {2E_{22}^f{E^m}{{\left( {\nu _{12}^f} \right)}^2} + E_{11}^f\left\{ {{E^m}\left( {\nu _{23}^f - 1} \right) - } \right.} \right.\\ \;\;\;\;\;\left. {\left. {E_{22}^f\left[ {2{{\left( {{\nu ^m}} \right)}^2} + {\nu ^m} - 1} \right]} \right\}} \right\}/\left\{ {E_{11}^f\left[ {E_{22}^f + } \right.} \right.\\ \;\;\;\;\;\left. {\left. {{E^m}\left( {1 - \nu _{23}^f} \right) + E_{22}^f{\nu ^m}} \right] - 2E_{22}^f{E^m}{{\left( {\nu _{12}^f} \right)}^2}} \right\} \end{array} $ | (20.6) |
| $ B = \frac{{{E^m}\left( {1 - \nu _{23}^f} \right) - E_{22}^f\left( {1 + {\nu ^m}} \right)}}{{E_{22}^f\left[ {{\nu ^m} + 4{{\left( {{\nu ^m}} \right)}^2} - 3} \right] - {E^m}\left( {1 + \nu _{23}^f} \right)}} $ | (20.7) |
where σu, tm, σu, cm and σu, sm are the original tensile, compressive and shear strengths of the matrix, respectively. It is noted that under a longitudinal load, the stress field of the matrix is uniform and no stress concentration occurs in such a case.
6.4 True Stresses of the MatrixLet
| $ \left\{ {\sigma _i^m} \right\} = {\left\{ {\sigma _{11}^m,\sigma _{22}^m,\sigma _{33}^m,\sigma _{23}^m,\sigma _{13}^m,\sigma _{12}^m} \right\}^{\rm{T}}} $ |
be the homogenized stresses of the matrix in a UD composite calculated from a micromechanics model with σ22m×σ33m=0. The true stresses of the matrix, {σim}={σ11m, σ22m, σ33m, σ23m, σ13m, σ12m}T, are determined as:
| $ \left\{ \begin{array}{l} \left\{ {\bar \sigma _i^m} \right\} = \\ \;\;\;\;{\left\{ {\sigma _{11}^m,{K_{22}}\sigma _{22}^m,0,{K_{23}}\sigma _{23}^m,{K_{12}}\sigma _{13}^m,{K_{12}}\sigma _{12}^m} \right\}^{\rm{T}}},\\ \;\;\;\;{\rm{if}}\;\sigma _{33}^m = 0\\ \left\{ {\bar \sigma _i^m} \right\} = \\ \;\;\;\;{\left\{ {\sigma _{11}^m,0,{K_{33}}\sigma _{33}^m,{K_{23}}\sigma _{23}^m,{K_{12}}\sigma _{13}^m,{K_{12}}\sigma _{12}^m} \right\}^{\rm{T}}},\\ \;\;\;\;{\rm{if}}\;\sigma _{22}^m = 0 \end{array} \right. $ | (21.1) |
| $ {K_{22}} = \left\{ \begin{array}{l} K_{22}^t,{\rm{if}}\;\sigma _{22}^m > 0\\ K_{22}^c,{\rm{if}}\;\sigma _{22}^m < 0 \end{array} \right. $ | (21.2) |
| $ {K_{33}} = \left\{ \begin{array}{l} K_{22}^t,{\rm{if}}\;\sigma _{33}^m > 0\\ K_{22}^c,{\rm{if}}\;\sigma _{33}^m < 0 \end{array} \right. $ | (21.3) |
Bridging tensor elements of a model for each of the 9 UD composites can be calculated through Eq. (5), using the corresponding elastic moduli given in Table 3. The thus obtained non-zero bridging tensor elements are summarized in Table 5.
| Table 5 Non-zero bridging tensor elements and coefficients λi's for the 9 composites by different models |
Under a uniaxial load, only the internal stress component of a constituent (fiber or matrix) along the loading direction is dominant. The other stress components, if any, are negligibly small, as indicted by Eqs. (8.1)-(8.6). Accordingly, a longitudinal failure of the composite subjected to a longitudinal load is controlled mostly by a fiber failure, whereas all the other failures induced by uniaxial loads result from matrix failures. We only need to determine the following relationships:
| $ \begin{array}{*{20}{c}} {\sigma _{11}^f = {\lambda _1}\sigma _{11}^0,\sigma _{22}^m = {\lambda _2}\sigma _{22}^0,}\\ {\sigma _{23}^m = {\lambda _3}\sigma _{23}^0,\sigma _{12}^m = {\lambda _4}\sigma _{12}^0} \end{array} $ | (22) |
where λi's depend on the bridging tensor. σ110, σ220, σ230, or σ120 is uniaxial load applied individually to the composite once at a time. Consider, for example, Eshelby's method for the E-glass/LY556 composite. From Table 5, the bridging tensor [Aij] is as follows:
| $ \left[ {\begin{array}{*{20}{c}} {0.031}&{0.676}&{0.676}&0&0&0\\ { - 0.016}&{1.775}&{0.179}&0&0&0\\ { - 0.016}&{0.179}&{1.775}&0&0&0\\ 0&0&0&{1.596}&0&0\\ 0&0&0&0&{1.304}&0\\ 0&0&0&0&0&{1.304} \end{array}} \right] $ |
Hence, the tensor [Bij]=(Vf[I]+Vm[Aij])-1 is (noting that Vf=0.62):
| $ \left[ {\begin{array}{*{20}{c}} {1.5771}&{ - 0.3}&{ - 0.3}&0&0&0\\ {0.007}&{0.773}&0&0&0&0\\ {0.007}&{ - 0.04}&{0.77}&0&0&0\\ 0&0&0&{0.815}&0&0\\ 0&0&0&0&{0.896}&0\\ 0&0&0&0&0&{0.896} \end{array}} \right] $ |
Substituting the thus obtained [Aij] and [Bij] into Eqs. (2) and (3) and meanwhile applying {σj}={1, 0, 0, 0, 0, 0}T, {σj}={0, 1, 0, 0, 0, 0}T, {σj}={0, 0, 0, 1, 0, 0}T and {σj}={0, 0, 0, 0, 0, 1}T to Eqs.(2) and (3) once at a time, we determine λ1=1.577, λ2=1.37, λ3=1.301 and λ4=1.169. For each of the 9 composites, λi is calculated by virtue of the 14 models, as shown in Table 5.
From Table 5, the longitudinal tensile and compressive, transverse tensile and compressive, transverse shear and longitudinal shear strengths of a UD composite are calculated through
| $ \begin{array}{*{20}{c}} {\sigma _{11}^{u,t} = \sigma _{u,t}^f/{\lambda _1},\sigma _{11}^{u,c} = \sigma _{u,c}^f/{\lambda _1},}\\ {\sigma _{22}^{u,t} = \sigma _{u,t}^m/\left( {K_{22}^t{\lambda _2}} \right),\sigma _{22}^{u,c} = \sigma _{u,c}^m/\left( {K_{22}^c{\lambda _2}} \right),}\\ {\sigma _{23}^u = \sigma _{u,s}^m/\left( {{K_{23}}{\lambda _3}} \right),\sigma _{12}^u = \sigma _{u,s}^m/\left( {{K_{12}}{\lambda _4}} \right)} \end{array} $ | (23) |
where K22t, K22c, K12 and K23 are the transverse tensile, transverse compressive, longitudinal shear and transverse shear SCFs of the matrix, respectively.
Using the relevant parameters in Tables 1 and 2, the SCFs of the matrix in each of the 9 composites are calculated, as indicated in Table 6. The predicted strengths are compared with the experimental measurements (Table 2), and the averaged relative correlation errors for all of the 14 models are summarized in Table 7. Bridging Model is still overall the most accurate, although the accuracy difference between the Bridging Model's and the other models' predictions for the strengths is less than those for the elastic properties of the composites. As more than double of the constituent data and more other information are required, meaning that more than double the accumulated error may be involved, a reasonable correlation error to be expected in a micromechanical strength prediction would be greater than 10% and up to 20%. Table 7 shows that this is attainable by the current theory.
| Table 6 SCFs of the matrices in the 9 composites |
| Table 7 Overall averaged errors in prediction of the uniaxial strengths of the 9 UD composites by different micromechanics models |
If no SCFs of the matrix are taken into account, i.e. if K22t=K22c=K12=K23≡1 are assigned in Eqs. (23), the overall correlation error by a model other than the rule of mixture and Eshelby methods would be much greater than that shown in Table 7. Consider, for instance, Bridging Model. The error between the predicted and measured transverse tensile, transverse compressive, transverse shear, and longitudinal shear strengths of the 9 composites is 115.3%, 5.22 times greater than that when the SCFs are taken into account. Hence, the most important factor to influence a composite strength prediction is the SCFs of a matrix in the composite.
8 ConsistencyEqs. (1)-(5) are valid for both 2D and 3D stress states. Any micromechanics model can result in two sets of internal stress formulae, i.e. 2D and 3D formulae, respectively. Suppose that the composite is subjected to a planar stress state, {σ110, σ220, σ120}. The resulting internal stresses in the fiber and matrix by the 2D formulae are denoted as {σ11f, 2D, σ22f, 2D, σ33f, 2D, σ23f, 2D, σ13f, 2D, σ12f, 2D} and {σ11m, 2D, σ22m, 2D, σ33m, 2D, σ23m, 2D, σ13m, 2D, σ12m, 2D}, where σ33f, 2D=σ23f, 2D=σ13f, 2D = σ33m, 2D=σ23m, 2D= σ13m, 2D=0. On the other hand, the internal stresses {σ11f, 3D, σ22f, 3D, σ33f, 3D, σ23f, 3D, σ13f, 3D, σ12f, 3D} and {σ11m, 3D, σ22m, 3D, σ33m, 3D, σ23m, 3D, σ13m, 3D, σ12m, 3D} are also given by the 3D formulae when the composite is loaded with {σ110, σ220, 0, 0, 0, σ120}. If σijf, 2D=σijf, 3D and σijm, 2D=σijm, 3D for all i and j, the corresponding micromechanics model is consistent in the internal stress calculation.
A necessary and sufficient condition for a micromechanics model to be consistent is that its bridging tensor is always in an upper triangular form. If, for instance, A32≠0, we will get from Eq. (2) that σ33f=B32σ220≠0 and from Eq. (3) that σ33m= A32σ22f+A33σ33f≠0, where [Bij]=(Vf[I]+Vm[Aij])-1. The bridging tensor of Bridging Model, by definition, is always upper triangular, even when the matrix of a constituent undergoes a plastic deformation[16]. On the other hand, Table 5 shows that all of the other models are not consistent. The non-consistency implies that the homogenized internal stresses should be calculated using the full 3D stress formulae, even though the composite is subjected to a uniaxial load. To apply an analytical model other than Bridging Model to the stress calculation, the 3D compliance tensors of the fiber, matrix and the composite should be used in Eq. (5) to obtain the 3D bridging tensor. If a numerical method is applied to predict a composite property, a 3D rather than 2D RVE geometry should be discretized.
9 ConclusionOnce SCFs of the matrix in a composite are determined and are taken into account, any micromechanics model originally developed for composite stiffness can perform a reasonable prediction for a composite strength as well. An assessment on the predictive ability of 14 models for the stiffness and strength of a UD composite against the benchmark data in WWFEs indicates that Bridging Model operates with the smallest correlation error in both the stiffness and strength predictions. Moreover, it is the only consistent model in the internal stress calculation. A full 3D approach should be applied if any other model is used to simulate a composite's effective property.
AcknowledgementsWe greatly appreciate the help of Dr. Chen at Xi'an Jiaotong University for supplying the data in Table 3 predicted by finite volume method and generalized method of cells.
| [1] |
Bogdanovich A E, Sierakowski R L. Composite materials and structures: Science, technology and applications-A compendium of books, review papers, and other sources of information. Appl. Mech. Rev., 1999, 52(12): 351-366. DOI:10.1115/1.3098921 (  0) 0) |
| [2] |
Pindera M J, Khatam H, Drago A S, et al. Micromechanics of spatially uniform heterogeneous media: A critical review and emerging approaches. Comp. Part B:Engieering, 2009, 40(5): 349-378. DOI:10.1016/j.compositesb.2009.03.007 (  0) 0) |
| [3] |
Nemat-Nasser S, Hori M. Micromechanics: Overall Properties of Heterogeneous Materials. Amsterdam: Elsevier, 2013. (  0) 0) |
| [4] |
Hinton M J, Kaddour A S, Soden P D. Failure Criteria in Fiber Reinforced Polymer Composites. Amsterdam: Elsevier, 2004. (  0) 0) |
| [5] |
Huang Z M, Liu L. Predicting strength of fibrous laminates under triaxial loads only upon independently measured constituent properties. Int. J. Mech. Sci., 2014, 79(1): 105-129. DOI:10.1016/j.ijmecsci.2013.08.010 (  0) 0) |
| [6] |
Yao Z, Huang Z-M. Stress concentration factor in the matrix reinforced with fiber having an interface layer. J. Reinf. Plastics Comp., 2013, 32: 105-123. DOI:10.1177/0731684412453214 (  0) 0) |
| [7] |
Yao Z, Huang Z-M. Stress concentration factors in the matrix with different imperfect interfaces. Int. J. Damage Mechanics., 2014, 23: 745-771. DOI:10.1177/1056789513512345 (  0) 0) |
| [8] |
Liu L, Huang Z-M. Stress concentration factor in matrix of a composite reinforced with transversely isotropic fibers. J. Comp. Mater., 2014, 48: 81-98. DOI:10.1177/0021998312469237 (  0) 0) |
| [9] |
Huang Z M, Xin L M. Stress concentration factor in matrix of a composite subjected to transverse compression. Int. J. Appl. Mech., 2016, 8(3): 1650034-1. DOI:10.1142/S1758825116500344 (  0) 0) |
| [10] |
Huang Z M, Xin L M. In situ strengths of matrix in a composite. Acta Mech. Sinica, 2017, 33(1): 120-131. DOI:10.1007/s10409-016-0611-1 (  0) 0) |
| [11] |
Zhou Y, Huang Z M, Liu L. Prediction of interfacial debonding in fiber-reinforced composite laminates. Poly. Comp. (in press).
(  0) 0) |
| [12] |
Ryan S, Wicklein M, Mouritz A, et al. Theoretical prediction of dynamic composite material properties for hypervelocity impact simulations. Int. J. Impact Eng., 2009, 36(7): 899-912. DOI:10.1016/j.ijimpeng.2008.12.012 (  0) 0) |
| [13] |
Younes R, Hallal A, Fardoun F, et al. Comparative review study on elastic properties modeling for unidirectional composite materials. In: Hu, N. (ed.) Composites and Their Properties, 2012. 391-408.DOI: 10.5772/50362.
(  0) 0) |
| [14] |
Medikonda S T A, Hamm R. A comparative study of the effect of representative volume cell (RVC) boundary conditions on the elastic properties of a micromechanics based unidirectional composite material model. Int. J. Comp. Mater., 2017, 7(2): 51-71. DOI:10.5923/j.cmaterials.20170702.03 (  0) 0) |
| [15] |
Qu J, Cherkaoui M. Fundamentals of Micromechanics of Solids. New Jersey: John Wiley & Sons, 2006.
(  0) 0) |
| [16] |
Huang Z M, Zhou Y X. Strength of Fibrous Composites. Zhejiang University Press & Springer, 2011.
(  0) 0) |
| [17] |
Mori T, Tanaka K. Average stress in matrix and average energy of materials with misfitting inclusion. Acta Metall., 1973, 21(5): 571-574. DOI:10.1016/0001-6160(73)90064-3 (  0) 0) |
| [18] |
Benveniste Y. A new approach to the application of Mori-Tanaka's theory in composite materials. Mech. Mater., 1987, 6(2): 147-157. DOI:10.1016/0167-6636(87)90005-6 (  0) 0) |
| [19] |
Tsai S W, Hahn H T. Introduction to Composite Materials. CRC Press, 1980.
(  0) 0) |
| [20] |
Chamis CC. Mechanics of composite materials: Past, present and future. J. Comp. Tech. Res., 1989, 11(1): 3-14. DOI:10.1016/0010-4361(90)90294-7 (  0) 0) |
| [21] |
Halpin Affdl J C, Kardos J L. The Halpin-Tsai equations: A review. Poly. Eng. Sci., 1976, 16(5): 344-352. DOI:10.1002/pen.760160512 (  0) 0) |
| [22] |
Swanson S R. Introduction to design and analysis with advanced composite materials. Prentice Hall, 1997.
(  0) 0) |
| [23] |
Christensen R M, Lo K H. Solutions for effective shear properties in three phase sphere and cylinder models. J. Mech. Phys. Solids., 1979, 27(4): 315-330. DOI:10.1016/0022-5096(79)90032-2 (  0) 0) |
| [24] |
Aboudi J, Arnold S M, Bednarcyk B A. Micromechanics of composite materials: A generalized multiscale analysis approach. Butterworth-Heinemann, 2012.
(  0) 0) |
| [25] |
Cavalcante M A A, Pindera M J, Khatam H. Finite-volume micromechanics of periodic materials: Past, present and future. Comp. Part B:Engineering, 2012, 43(6): 2521-43. DOI:10.1016/j.compositesb.2012.02.006 (  0) 0) |
| [26] |
Cavalcante M A A, Pindera M J. Generalized finite-volume theory for elastic stress analysis in solid mechanics—Part Ⅰ: Framework. J. Appl. Mech. ASME, 2012, 79(5): 051006. DOI:10.1115/1.4006805 (  0) 0) |
| [27] |
Barbero E J. Finite element analysis of composite materials using Abaqus. Boca Raton: CRC Press, 2013.
(  0) 0) |
| [28] |
Segurado J, Llorca J. A numerical approximation to the elastic properties of sphere-reinforced composites. J. Mech. Phy. Solids., 2002, 50(10): 2107-2121. DOI:10.1016/S0022-5096(02)00021-2 (  0) 0) |
| [29] |
Melro A R, Camanho P P, Pinho S T. Generation of random distribution of fibres in long-fibre reinforced composites. Comp. Sci. Tech., 2008, 68(9): 2092-2102. DOI:10.1016/j.compscitech.2008.03.013 (  0) 0) |
| [30] |
Chateau C, Gelebart L, Bornert M, et al. Micromechanical modeling of the elastic behavior of unidirectional CVI SiC/SiC composites. Int. J. Solids Struct., 2015, 58: 322-334. DOI:10.1016/j.ijsolstr.2014.11.020 (  0) 0) |
| [31] |
MSC Software Corporation.DIGIMAT user manual (Release 5.1.2, 2014). Newport Beach: MSC Software Corporation.2014.
(  0) 0) |
| [32] |
Liu L, Huang Z-M. A note on Mori-Tanaka's method. ACTA Mech. Solida Sinica., 2014, 27(3): 234-244. DOI:10.1016/S0894-9166(14)60033-1 (  0) 0) |
| [33] |
Zhang C C, Wang Y C, Huang Z M. Effective elastic moduli of a transversely isotropic matrix based composite. Appl. Math. Mech. (in press)
(  0) 0) |
| [34] |
Wang Y C, Huang Z M. Bridging tensor with an imperfect interface. Eur. J. Mech. - A/Solids, 2016, 56: 73-91. DOI:10.1016/j.euromechsol.2015.10.006 (  0) 0) |
| [35] |
Chen Q, Tu W Q, Liu R N, et al. Parametric multiphysics finite-volume theory for periodic composites with thermo-electro-elastic phases. J. Intell. Mater. Sys. Struct., 2017, 29(4): 1045389X17711789. DOI:10.1177/1045389X17711789 (  0) 0) |
| [36] |
Soden D, Hinton M J, Kaddour A S. Lamina properties, lay-up configurations and loading conditions for a range of fiber-reinforced composite laminates. Comp. Sci. Tech., 1998, 58: 1011-1022. DOI:10.1016/S0266-3538(98)00078-5 (  0) 0) |
| [37] |
Kaddour A S, Hinton J M. Input data for test cases used in benchmarking triaxial failure theories of composites. J. Comp. Mater., 2012, 46(19-20): 2295-2312. DOI:10.1177/0021998312449886 (  0) 0) |
| [38] |
Kaddour A S, Hinton M J, Smith P A, et al. Mechanical properties and details of composite laminates for test cases used in the third world-wide failure exercise. J. Comp. Mater., 2013, 47(20-21): 2427-2442. DOI:10.1177/0021998313499477 (  0) 0) |
| [39] |
Heinrich C, Aldridge M, Wineman A S, et al. The influence of the representative volume element (RVE) size on the homogenized response of cured fiber composites. Modell. Simul. Mater. Sci. Eng., 2012, 20(7): 075007. DOI:10.1088/0965-0393/20/7/075007 (  0) 0) |
| [40] |
Gonzalez C, Llorca J. Mechanical behavior of unidirectional fiber-reinforced polymers under transverse compression: Microscopic mechanisms and modeling. Comp. Sci. Tech., 2007, 67(13): 2795-2806. DOI:10.1016/j.compscitech.2007.02.001 (  0) 0) |
| [41] |
Jimenez F L, Pellegrino S. Constitutive modeling of fiber composites with a soft hyperelastic matrix. Int. J. Solids Struct., 2012, 49(3-4): 635-647. DOI:10.1016/j.ijsolstr.2011.11.006 (  0) 0) |
| [42] |
Huang Y, Jin K K, Ha S K. Effects of fiber arrangement on mechanical behavior of unidirectional composites. J. Comp. Mater., 2008, 42(18): 1851-1871. DOI:10.1177/0021998308093910 (  0) 0) |
| [43] |
Sertse H M, Goodsell J, Ritchey A J, et al. Challenge problems for the benchmarking of micromechanics analysis: Level I initial results. J. Comp. Mater., 2018, 52(1): 61-80. DOI:10.1177/0021998317702437 (  0) 0) |
| [44] |
Tsai J L, Chi Y K. Investigating thermal residual stress effect on mechanical behaviors of fiber composites with different fiber arrays. Comp. Part B:Engineering, 2008, 39(4): 714-721. DOI:10.1016/j.compositesb.2007.05.005 (  0) 0) |
| [45] |
Gonzalez C, Llorca J. Mechanical behavior of unidirectional fiber-reinforced polymers under transverse compression: Microscopic mechanisms and modeling. Comp. Sci. Tech., 2007, 67(13): 2795-2806. DOI:10.1016/j.compscitech.2007.02.001 (  0) 0) |
| [46] |
Eshelby J D. The determination of the elastic field of an ellipsoidal inclusion and related problems. Proc. Royal Soc., 1957, 241(1226): 367-396. DOI:10.1098/rspa.1957.0133 (  0) 0) |
| [47] |
Chen T, Dvorak G J, Benveniste Y. Stress fields in composites reinforced by coated cylindrically orthotropic fibers. Mech. Mater., 1990, 9(1): 17-32. DOI:10.1016/0167-6636(90)90027-D (  0) 0) |
 2018, Vol. 25
2018, Vol. 25


