2. School of Energy and Power Engineering, Dalian University of Technology, Dalian 116024, China
Flow and heat transport through porous media is widespread in natural system and engineering practice[1-3]. Two types of models for the energy equation were commonly described in various heat transfer process, involving local thermal equilibrium (LTE) or non-equilibrium (LTNE) model[4-6]. Using LTE and LTNE models respectively, Lee and Vafai[7] obtained and compared the exact temperature solutions of thermal transfer in a porous media channel. The thermal bifurcation phenomenon in a forced convection porous medium channel by employing LTNE model was analyzed by Yang and Vafai[8]. Considering the Darcy-Binkman-Forchheimer flow, Jiang and Ren[9] used LTNE model to discuss forced convection process in a porous media channel. A metal foam microchannel heat sink was constructed by Li et al.[10], and the heat transfer characteristics were numerically investigated using Brinkman extended Darcy and LTNE model. Based on fully developed flow and LTNE model, Xu[11]theoretically investigated the flow inertia, velocity jumps, and thermal slips in a microchannel partially filled with micro-porous media. Using LTNE model, Shen et al.[12]numerically studied the heat exchange characteristics of copper metal foam with different shapes in micro channels. Based on the Brinkman-Darcy momentum equation, considering viscous dissipation effect, Chen and Tso[13] numerically simulated the forced convection process using LTNE model in a porous media channel, and gained the fluid-solid temperature distributions with invariant heat flux.
Most initial research is based on Newtonian fluid. In numerous practical engineering applications, many fluids belong to the category of non-Newtonian fluid, such as blood and cell fluid in human body, slurry, pigment, paint, and industrial grease in chemical production, milk, and beverage in food industry, showing rheological properties of non-Newtonian fluid[14-15]. In the study of Thayalan and Huang[16], the momentum boundary layer integral method was used to solve the velocity distribution and the temperature distribution using LTNE model. The effects of Da, n, Bi, and k on convection heat transfer were analyzed. However, the inertial effect and viscous dissipation effect of non-Darcy flow were not considered. Using Brinkman-Forchheimer extended Darcy flow model, Tian et al.[17]compared and analyzed three different viscous dissipation terms on forced convection thermal characteristic of power-law fluid in a porous media channel, but did not take into consideration fluid-solid temperature difference.
To strengthen heat transfer of tube-fin heat exchangers[18] or heat dissipation of microelectronic equipment, microchannel cooling technology using nanofluid is an effective solution. For example, for the convection heat transfer process of magnetic nanofluid[19-20] in porous microchannels, the non-Newtonian characteristics of nanofluids[21-22], LTNE effects, and other micro scale effects[23] (such as viscous dissipation effect of nanofluids, nonlinear drag inertia effect, thermal dispersion effect, etc.) may need to be considered concurrently.
In summary, for the rheological characteristics of power-law fluid, there are few reports on the thermal transport in porous media including fluid-solid temperature difference, Darcy-Brinkman-Forchheimer flow, and viscous dissipation effect at the same time. In this paper, a convection model of power-law fluid in porous media channel is established. The effects of dimensionless parameters, such as Bi, k, Br, Da, F, and n on temperature distribution and heat transfer characteristics are analyzed numerically.
1 Physical Mathematical ModelIn this study, as shown in Fig. 1, an infinite parallel channel of height 2H was investigated. Considering the fully developed steady state of two-dimensional laminar flow heat transfer, the z-direction flow heat transfer is not considered (the width of the model is much larger than the height, and the conditions in the width direction are uniform and symmetrical). Assume the fluid is a single-phase, incompressible power-law fluid, the porous skeletons are rigid and isotropic, and the fluid and skeletons are in local thermal non-equilibrium. Except for the fluid viscosity, the themo-physical properties of the fluid and solid phases are considered constant. The velocity gradient along flow direction (in x-direction)is infinitesimal, and the chemical reaction, radiation heat, and the axial conduction are ignored.

|
Fig.1 Model of the considered problem |
The Forchheimer-Brinkman extended Darcy's law by modifying the momentum equation for power-law fluid[24] is expressed as
| $ \frac{\mu}{\varepsilon^{n}} \frac{\mathrm{d}}{\mathrm{d} y}\left[\left|\frac{\mathrm{d} u}{\mathrm{~d} y}\right|^{n-1} \frac{\mathrm{d} u}{\mathrm{~d} y}\right]-\frac{\mu}{K^{*}} u^{n}-\rho b u^{2}+\frac{\mathrm{d} p}{\mathrm{~d} x}=0 $ | (1) |
Dimensionless parameters are defined as follows: n represents the power-law index, transverse coordinate Y =y/H; centerline velocity uc=(K*dp/(μdx))1/n; characteristic velocity based on centerline velocity U=u/uc; inertial parameter F= ρbK*(uc)2-n/μ; Da number Da=K*2/(n+1)/H2/ε2n/(n+1). Then Eq. (1) with its boundary conditions is non-dimensionalized as
| $ \frac{\mathrm{d}}{\mathrm{d} Y}\left[\left|\frac{\mathrm{d} U}{\mathrm{~d} Y}\right|^{n-1} \frac{\mathrm{d} U}{\mathrm{~d} Y}\right]=\frac{U^{n}+F U^{2}-1}{D a^{(1+n) / 2}} $ | (2) |
The corresponding energy equations are written as
| $ k_{\mathrm{f}, \text { eff }} \frac{\partial^{2} T_{\mathrm{f}}}{\partial y^{2}}+h_{\mathrm{sf}} a_{\mathrm{sf}}\left(T_{\mathrm{s}}-T_{\mathrm{f}}\right)+\varOmega=\rho c_{P} u \frac{\partial T_{\mathrm{f}}}{\partial x} $ | (3) |
| $ \varOmega=\frac{\mu u^{n+1}}{K^{*}}+\frac{\mu}{\varepsilon^{n}}\left|\frac{\mathrm{d} u}{\mathrm{~d} y}\right|^{n-1}\left(\frac{\mathrm{d} u}{\mathrm{~d} y}\right)^{2}+\rho b u^{3} $ | (4) |
| $ k_{\mathrm{s}, \text { eff }} \frac{\partial^{2} T_{\mathrm{s}}}{\partial y^{2}}-h_{\mathrm{sf}} a_{\mathrm{sf}}\left(T_{\mathrm{s}}-T_{\mathrm{f}}\right)=0 $ | (5) |
where Tf, Ts, ks, eff, kf, eff are fluid-solid temperature and thermal conductivity, respectively; Ω is representative of different viscous dissipation, involving Darcy term (internal heating), Al-Hadhrami term (frictional heating), and Forchheimer term (nonlinear drag power); hsf and asf are interface heat transfer coefficient and specific surface area, respectively.
According to the given conditions, the temperature gradient
| $ \frac{\mathrm{d} T_{\mathrm{m}}}{\mathrm{d} x}=\frac{q_{\mathrm{w}}+\int_{0}^{H} \varOmega}{\rho c_{p} H u_{\mathrm{m}}} $ | (6) |
| $ q_{\mathrm{w}}=\left.k_{\mathrm{f}, \text { eff }} \frac{\partial T_{\mathrm{f}}}{\partial y}\right|_{y=0}+\left.k_{\mathrm{s}, \text { eff }} \frac{\partial T_{\mathrm{s}}}{\partial y}\right|_{y=0} $ | (7) |
Other dimensionless parameters are defined as follows:
Substituting Eqs. (4), (6), and (7) into Eq. (3), combining with Eq. (5), and integrating along the channel cross section, the following energy equation is obtained:
| $ \begin{aligned} k &\frac{\partial^{2} \theta_{\mathrm{f}}}{\partial Y^{2}}+\frac{\partial^{2} \theta_{s}}{\partial Y^{2}}=U^{\prime}\left(1+B r \int_{0}^{1}\left(U^{\prime}\right)^{n+1} \mathrm{~d} Y+\right. \\ &\left.B r^{\prime} \int_{0}^{1}\left|\frac{\mathrm{d} U^{\prime}}{\mathrm{d} Y}\right|^{n-1}\left(\frac{\mathrm{d} U^{\prime}}{\mathrm{d} Y}\right) \mathrm{d} Y+B r F^{\prime} \int_{0}^{1}\left(U^{\prime}\right)^{3} \mathrm{~d} Y\right)- \\ &B r\left(U^{\prime}\right)^{n+1}-B r^{\prime}\left|\frac{\mathrm{d} U^{\prime}}{\mathrm{d} Y}\right|^{n-1}\left(\frac{\mathrm{d} U^{\prime}}{\mathrm{d} Y^{\prime}}\right)^{2}-B r F^{\prime}\left(U^{\prime}\right)^{3} \end{aligned} $ | (8) |
When the channel wall has finite thickness and the thermal conductivity is relatively high, under the fully developed steady state of two-dimensional flow heat transfer, θf=θs=θw, and considering axisymmetric boundary conditions, the corresponding dimensionless boundary conditions can be justified by
| $ Y=0, U=U^{\prime}=0, \theta_{\mathrm{f}}=\theta_{\mathrm{s}}=0 $ | (9a) |
| $ Y=1, \mathrm{~d} U / \mathrm{d} Y=\mathrm{d} U^{\prime} / \mathrm{d} Y=0, \partial \theta_{\mathrm{f}} / \mathrm{d} Y=\partial \theta_{\mathrm{s}} / \mathrm{d} Y=0 $ | (9b) |
It is noteworthy that Br characterizes the intensity of the viscous dissipation (Br=0, which indicates no viscous dissipation). It can be seen from the above expression that the viscous dissipation effect is closely related to Br, Da, F, and n. The Nusselt number[25] can be evaluated as
| $ N u=\frac{2 H q_{\mathrm{w}}}{k_{\mathrm{f}, \mathrm{eff}}\left(T_{\mathrm{w}}-T_{\mathrm{m}}\right)}=-\frac{2}{k \theta_{\mathrm{fb}}} $ | (10) |
where dimensionless average temperature of fluid phase
For LTE model, θf=θs. According to Eqs. (8) and (9), the dimensionless Nusselt number can be rewritten as follows:
| $ N u_{\mathrm{LTE}}=\frac{2 H q_{\mathrm{w}}}{k_{\mathrm{f}, \mathrm{eff}}^{\prime}\left(T_{\mathrm{w}}-T_{\mathrm{m}}\right)}=-\frac{2}{k \theta_{\mathrm{b}}}=-\frac{2(k+1)}{k \theta_{\mathrm{fb}}} $ | (11) |
The difference between LTE and LTNE models can be evaluated as E=NuLTE-Nu
2 Numerical Methods and SolversFrom the expressions of momentum equation and energy equation, it can be deduced that when the inertia flow and viscous dissipation effect are considered, there is a strong nonlinear effect of non-Darcy flow in porous media, which must be solved by numerical method. To do this, Eqs. (2) and (8) are first recombined and reduced to first order differential Eqs. (12). Obviously, this is a boundary value problem of ordinary differential equations. Then, assuming the initial value and a reasonable step size (0.01), the classical Runge Kutta method is used to carry out variable alternating numerical iteration from 0 to 1 until the iteration accuracy (10-5) and the boundary condition Eqs. (13) are satisfied. The parameters defined are as follows:
| $ y_{1}=U(Y), y_{2}=(\mathrm{d} U / \mathrm{d} Y)^{n} $ |
| $ y_{3}=\int\limits_{0}^{Y} U \mathrm{d} Y, y_{4}=\int\limits_{0}^{Y} U^{n+1} \mathrm{d} Y $ |
| $ y_{5}=\int\limits_{0}^{Y} U^{3} \mathrm{d} Y, y_{6}=\int\limits_{0}^{Y}(\mathrm{d} U / \mathrm{d} Y)^{n+1} \mathrm{d} Y $ |
| $ y_{7}=U_{\mathrm{m}}, y_{8}=\left(U_{\mathrm{m}}\right)^{n+1} $ |
| $ y_{9}=\left(U_{\mathrm{m}}\right)^{3}, y_{10}=\int\limits_{0}^{1} U^{n+1} \mathrm{d} Y $ |
| $ y_{11}=\int\limits_{0}^{1} U^{3} \mathrm{d} Y, y_{12}=\int\limits_{0}^{1}(\mathrm{d} U / \mathrm{d} Y)^{n+1} \mathrm{d} Y $ |
| $ y_{13(\mathrm{f})}=\theta_{(\mathrm{f})}(Y), y_{13(\mathrm{s})}=\theta_{(\mathrm{s})}(Y) $ |
| $ y_{14(\mathrm{f})}=\mathrm{d} \theta_{(\mathrm{f})} / \mathrm{d} Y, y_{14(\mathrm{s})}=\mathrm{d} \theta_{(\mathrm{s})} / \mathrm{d} Y $ |
and
| $ \begin{array}{l} \begin{array}{l} y_{1}^{\prime}=\left(y_{2}\right)^{\frac{1}{n}}, y_{2}^{\prime}=\left(\left(y_{1}\right)^{n}+F\left(y_{1}\right)^{2}-1\right) / D a^{\frac{n+1}{2}}\\ y_{3}^{\prime}=y_{1}, y_{4}^{\prime}=\left(y_{1}\right)^{n+1}, y_{5}^{\prime}=\left(y_{1}\right)^{3}, y_{6}^{\prime}=\left(y_{2}\right)^{\frac{n+1}{n}}\\ y_{7}^{\prime}=y_{8}^{\prime}=y_{9}^{\prime}=y_{10}^{\prime}=y_{11}^{\prime}=y_{12}^{\prime}=0, y_{13}^{\prime}=y_{14} \end{array}\\ \ \ \ \begin{aligned} k y&_{14(\mathrm{f})}^{\prime}+B i\left(y_{13(\mathrm{~s})}-y_{13(\mathrm{f})}\right)= \\ &\frac{y_{1}}{y_{7}}\left(1+B r \frac{y_{10}}{y_{8}}+B r^{\prime} \frac{y_{12}}{y_{8}}+F^{\prime} B r \frac{y_{11}}{y_{9}}\right)- \\ &B r \frac{\left(y_{1}\right)^{n+1}}{y_{8}}-B r^{\prime} \frac{\left(y_{2}\right)^{(n+1) / n}}{y_{8}}-F^{\prime} B r \frac{\left(y_{1}\right)^{3}}{y_{9}} \cdot \\ &y_{14(\mathrm{~s})}^{\prime}=B i\left(y_{13(\mathrm{~s})}-y_{13(\mathrm{f})}\right) \end{aligned} \end{array} $ | (12) |
| $ \begin{array}{l} y_{1}(0)=y_{2}(1)=y_{3}(0)=y_{4}(0)=y_{5}(0)= \\ \ \ \ \ y_{6}(0)=y_{13}(0)=y_{14}(1)=0 \\ \ \ \ \ \ \ y_{7}(0)=y_{3}(1), y_{8}(0)=\left(y_{3}(1)\right)^{n+1} \\ \ \ \ \ \ \ \ \ y_{9}(0)=\left(y_{3}(1)\right)^{3}, y_{10}(0)=y_{4}(1) \\ \ \ \ \ \ \ \ \ \ \ y_{11}(0)=y_{5}(1), y_{12}(0)=y_{6}(1) \end{array} $ | (13) |
Then the expression of the Nu number can be obtained by the Eqs. (10), (11), and (12):
| $ N u=-\frac{2 y_{7}}{k \int_{0}^{1} y_{1} y_{13(\mathrm{f})} \mathrm{d} Y} $ | (14) |
| $ N u_{\mathrm{LTE}}=-\frac{2(k+1) y_{7}}{k \int_{0}^{1} y_{1} y_{13(\mathrm{f})} \mathrm{d} Y} $ | (15) |
To prove the validation of the numerical program, the present solution with indirect decoupling analytical solution by Wang et al.[1] is compared in Fig. 2, which illustrates dimensionless fluid-solid temperature profile with varying Biot number and k number along the cross section when Da=0.1, Br=0, and F=0. It can be seen that the present numerical predictions and the work by Wang et al.[1] are in good agreement.

|
Fig.2 Validation and comparison of present solution with indirect analytical solution (Da=0.1, Br=0, F=0) |
In order to further verify numerical solution, suppose Br=F=0, θ(f)=θ(s)(assuming LTE model). When n=1 (Newton fluid), Da≤0.001, the value of Nu is 5.92, which is in line with the conclusion that when Darcy is a small number (i.e., piston flow, Nu is close to 6); when Da≥1000, the simulation result, Nu=4.121, is also consistent with the conclusion that when Darcy is very large (i.e., parallel plate Poiseuille flow, Nu tends to be 70/17), which is consistent with the research of Nield et al.[26] When n≠1 (non-Newtonian fluid), Da≥100, the simulation result of non-Newton fluid (n = 0.5, and n=1.5) are 4.380 and 4.010, respectively, which are in line with the conclusion of Chen and Tso[27].
3 Results and AnalysisTo characterize the effects of dimensionless parameters on convection heat transfer characteristics, such as Bi, k, Br, Da, F, n, and so on, there are many variables of velocity field and temperature field that can be solved. The velocity field is mainly affected by dimensionless Darcy number and Forchheimer number, and the dimensionless velocity profile of fluids for different power-law indexes with varying Da and F number had been characterized and analyzed in Ref. [17]. The relationship of temperature distribution and the Nusselt number of power-law fluid of shear thinning are focused on here.
3.1 Impact of BiFig. 3 describes the temperature profile of fluid and solid with change of Biot number and power-law indexes along the cross section in porous media. As depicted in Fig. 3, with the increase of Bi, the temperature difference of fluid and solid decreases gradually. When Bi =100, the change of temperature difference is not obvious. It can be inferred that with a lager Bi (>1000), the temperature of the two phases tends to be the same. This shows that a larger Bi reduces fluid-solid thermal resistance and enhances convection heat transfer rate. Meanwhile, it is found that the direction of heat flux near wall is opposite, and the phenomenon of heat flux divergence[28]occurs. With the decrease of Bi, the phenomenon of heat flux divergence is more obvious. It can also be seen that with the decrease of n, the fluid-solid temperature difference increases gradually, indicating the fluid-solid heat exchange is gradually weakened. It can be inferred that with the decrease of n, the rheological property of pseudoplasticity is enhanced, the ability of intermolecular entanglement is strong, and the mesh structure formed between the molecules has larger resistance to the flow, which affects the velocity distribution. Meanwhile, the skeleton shear makes the viscosity dissipation between molecules further enhanced, reducing local convection heat transfer rate.
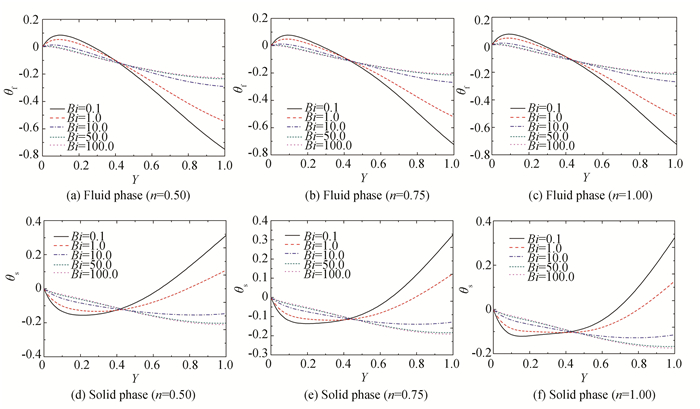
|
Fig.3 Temperature profile for varied Bi(k=1, Br=1, Da=0.1, F=0.1) |
3.2 Impact of k
The temperature profile with variable of k number and power-law indexes in porous media along the cross section is described in Fig. 4. With the increase of k, both fluid and solid temperatures increase, but fluid temperature rises faster than that of solid, which makes the fluid-solid temperature difference slightly reduce. As shown in Fig. 4, it is found that with the decrease of k (especially when k < 0.1), similar to Bi, bifurcation phenomenon appears at the temperature gradient near the wall, which indicates that the heat flow divergence will occur under certain parameter combination. It can also be seen that with the decrease of n, the fluid-solid temperature difference increases gradually, indicating that convection heat transfer rate decreases.
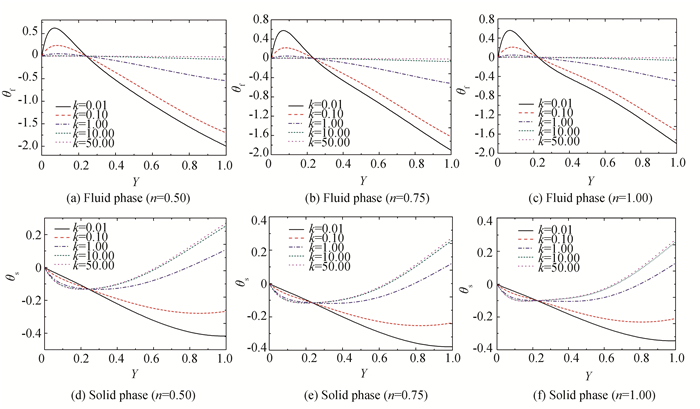
|
Fig.4 Temperature profile for varied k (Bi=1, Br=1, Da=0.1, F=0.1) |
3.3 Impact of Br
Fig. 5 describes the temperature profile with the change of Br and different power-law indexes in porous media along the cross section. It is shown that without the viscous dissipation terms (Br = 0), the temperature distribution of the two phases corresponding to three different fluids is almost equal, and the fluid-solid temperature difference is also small. When considering viscous dissipation effect (Br≠0), the fluid-solid temperature difference changes obviously with the increase of Br, which can be explained that when numbers of Br, Da, and F are not zero, the viscous dissipation effect generated by Darcy term, Alhdharami term, and Forchheimer term will overlap with each other. With the increase of Br, the friction heat dissipation effect corresponding to Alhdharami term is more intense in the vicinity of the wall and decreases towards the channel center. The fluid temperature increases by heating effects[29], and the temperature gradient bifurcation phenomenon also appears near the wall. On the contrary, the internal heat dissipation generated by Darcy term plays a major role near the channel center, which reduces fluid temperature and increases solid temperature. It should be noted that there is a critical value of Br, where the sign of the dimensionless temperature changes. It can also be seen that with the decrease of n, the rheological property of pseudoplasticity is enhanced, and the temperature difference increases gradually, which shows that the convection heat transfer between two phases decreases somewhat, and the effects of Br on the pseudoplastic fluid is more obvious.
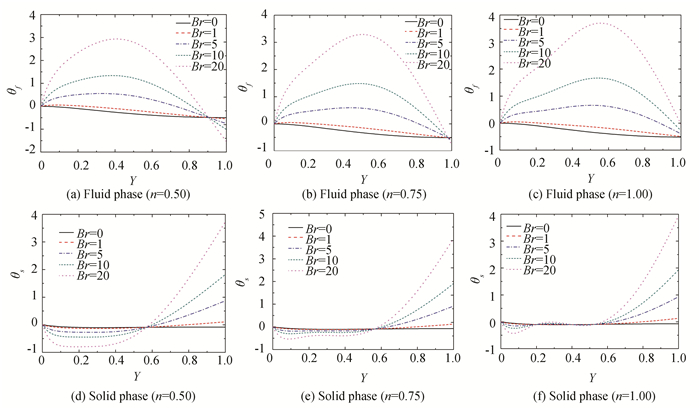
|
Fig.5 Temperature profile for varied Br (Bi=1, k=1, Da=0.1, F=0.1) |
3.4 Impact of Da
Fig. 6 plots the temperature profile with change of Darcy number and different power-law indexes along the cross section. It is observed that the fluid-solid temperature difference increases obviously with the increase of Da. It can be explained that the larger Da makes the flow deviate from Darcy flow, and the non-Darcy flow area increases gradually. The velocity profile tends to be a parabola shape, the contact surface area of heat transfer decreases for the increased porosity, leading to the decrease of convection heat transfer rate, and the fluid-solid temperature difference gradually increases. Meanwhile, it is presented that when Da is small, the temperature difference increases with decrease of n, indicating the convection heat transfer is weakened. On the contrary, with a large Da, a smaller fluid-solid temperature difference is obtained with the decrease of n, and the convection heat transfer is enhanced. The above results show that the range of influence of Da on pseudoplastic fluid is small, which is consistent with Ref. [17].
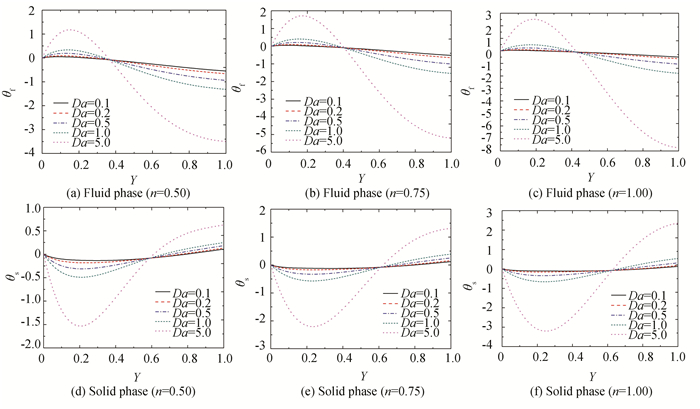
|
Fig.6 Temperature profile for varied Da(Bi=1, k=1, Br=1, F=0.1) |
3.5 Impact of F
The temperature profile along the cross section with the change of F number and different power-law indexes is depicted in Fig. 7. It is observed that increasing F induces a slight increase of fluid and solid temperatures, and the phenomenon of heat flux divergence occurs near the wall. The flow speed increases with a larger F, and the flow resistance of solid matrix to the fluid increases. However, it can produce a larger velocity gradient near the wall, and the velocity field will be modified by Drag forces dissipation effect. The influence of the change of F on shear thinning fluid is also more obvious.
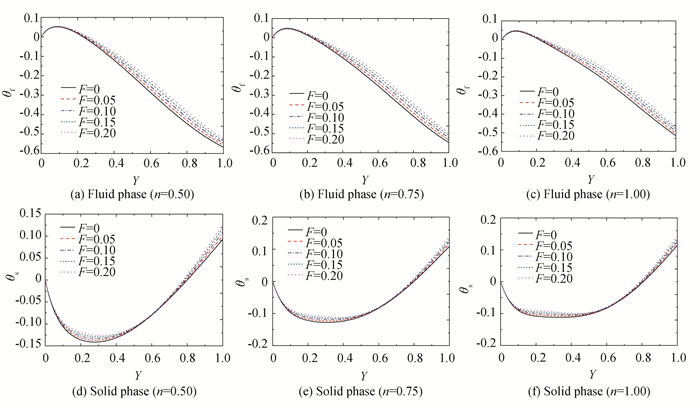
|
Fig.7 Temperature profile for varied F(Bi=1, k=1, Br=1, Da=0.1) |
3.6 Impact of Parameters on Nu
The convection heat transfer characteristics with the change of related parameters (Bi, k, Br, Da, F, and n) is described in Fig. 8. The difference between LTE model and LTNE model is also evaluated. As described in Fig. 8(a), with the increase of Bi and n, Nu increases gradually, while the difference of E decreases gradually with the increase of Bi and the decrease of n. Contrary to Fig. 8(a), with the increase of k, Nu decreases quickly as depicted in Fig. 8(b), while the difference of E decreases with the increase of k and the decrease of n. Fig. 8(a) and Fig. 8(b) show that the local thermal equilibrium is approaching with a larger number of Bi and k.

|
Fig.8 Effects of related quantities on Nu |
As illustrated in Fig. 8(c), because of the reverse of fluid temperature, Nu has negative value, which shows that for a certain combination of other parameters, there is a maximum value of Nu when an appropriate Br number is selected. The general trend of the difference decreases with the increase of Br. Fig. 8(d) shows that with the increase of Da, the number of Nu and E decrease gradually, but the decrease is becoming slower for pseudoplastic fluid. As depicted in Fig. 8(e), with the increase of F and n, the number of Nu and E increases gradually. It can be explained that the larger velocity makes the fluid flow deviate from the Darcy region, and LTNE effect is more significant.
4 Conclusions1) The general model of power-law fluid forced convection considering LTNE and viscous dissipation effects in a porous media channel was established. The calculation expressions of dimensionless fluid-solid temperature profiles and Nusselt number were derived with a clear relationship between physical parameters. The classical fourth-order Runge Kutta method was applied to numerically solve self-programmed solution under a constant heat flux boundary condition.
2) With a bigger Bi and k, the temperature profile of solid was close to that of fluid, which means a local thermal equilibrium was reached. While with the decrease of Bi, k, Br, Da, and the increase of F, the difference of temperature profile between fluid and solid increased gradually, intensifying the thermal non-equilibrium effect, and the fluid with different power-law indexes also had a non-negligible impact on the convection heat transfer characteristics.
| [1] |
Wang K Y, Wang D Z, Li P C. Two solutions to the heat transfer model of porous media plate channel. Applied Mathematics and Mechanics, 2015, 36(5): 494-504. (in Chinese) (  0) 0) |
| [2] |
Sharma K, Deepu P, Kumar S. Convective heat transfer in a tube filled with homogeneous and inhomogeneous porous medium. International Communications in Heat and Mass Transfer, 2020, 117: 104791. DOI:10.1016/j.icheatmasstransfer.2020.104791 (  0) 0) |
| [3] |
Ijeje J J, Gan Q, Cai J. Influence of permeability anisotropy on heat transfer and permeability evolution in geothermal reservoir. Advances in Geo-Energy Research, 2019, 3(1): 43-51. DOI:10.26804/ager.2019.01.03 (  0) 0) |
| [4] |
Alomar O R. Analysis of variable porosity, thermal dispersion, and local thermal non-equilibrium on two-phase flow inside porous media. Applied Thermal Engineering, 2019, 154: 263-283. DOI:10.1016/j.applthermaleng.2019.03.069 (  0) 0) |
| [5] |
Buonomo B, Manca O, Lauriat G. Forced convection in micro-channels filled with porous media in local thermal non-equilibrium conditions. International Journal of Thermal Sciences, 2014, 77: 206-222. DOI:10.1016/j.ijthermalsci.2013.11.003 (  0) 0) |
| [6] |
Lu L, Xin C Y, L iu, Z X. Review of local thermal non-equilibrium model of porous media. Journal of Engineering for Thermal Energy and Power, 2019, 34(7): 1-8. (  0) 0) |
| [7] |
Lee D Y, Vafai, K. Analytical characterization and conceptual assessment of solid and fluid temperature differentials in porous media. International Journal of Heat and Mass Transfer, 1999, 42(3): 423-435. DOI:10.1016/S0017-9310(98)00185-9 (  0) 0) |
| [8] |
Yang K, Vafai K. Analysis of temperature gradient bifurcation in porous media-An exact solution. International Journal of Heat and Mass Transfer, 2010, 53: 4316-4325. DOI:10.1016/j.ijheatmasstransfer.2010.05.060 (  0) 0) |
| [9] |
Jiang P X, Ren Z P. Numerical investigation of forced convection heat transfer in porous media using a thermal non-equilibrium model. International Journal of Heat and Fluid Flow, 2001, 22: 102-110. DOI:10.1016/S0142-727X(00)00066-7 (  0) 0) |
| [10] |
Li Y T, Gong L, Xu M H, et al. Analysis of flow and heat transfer characteristics of metal foam composite microchannel heat sink. Chinese Science Bulletin, 2019, 64(2): 215-222. DOI:10.1360/N972018-00191 (  0) 0) |
| [11] |
Xu H J. Thermal transport in microchannels partially filled with micro-porous media involving flow inertia, flow/thermal slips, thermal non-equilibrium and thermal asymmetry. International Communications in Heat and Mass Transfer, 2020, 110: 104404. DOI:10.1016/j.icheatmasstransfer.2019.104404 (  0) 0) |
| [12] |
Shen B B, Yan H B, Sunden B, et al. Forced convection and heat transfer of water-cooled microchannel heat sinks with various structured metal foams. International Journal of Heat and Mass Transfer, 2017, 113: 1043-1053. DOI:10.1016/j.ijheatmasstransfer.2017.06.004 (  0) 0) |
| [13] |
Chen G M, Tso C P. Forced convection with viscous dissipation using a two equation model in a channel filled by a porous medium. International Journal of Heat and Mass Transfer, 2011, 54(9-10): 1791-1804. DOI:10.1016/j.ijheatmasstransfer.2010.12.037 (  0) 0) |
| [14] |
Tian X W, Wang P, Xu S M. Flow resistance characteristics of power law fluid flow through granular porous medium. Journal of Harbin Institute of Technology, 2017, 49(1): 126-132. DOI:10.11918/j.issn.0367-6234.2017.01.018 (  0) 0) |
| [15] |
Tian X W, Xu S M, Sun Z H, et al. Experimental study on flow and heat transfer of power law fluid in structured packed porous media of particles. Experimental Thermal and Fluid Science, 2018, 90: 37-47. DOI:10.1016/j.expthermflusci.2017.08.029 (  0) 0) |
| [16] |
Thayalan N, Hung Y M. Momentum integral method for forced convection in thermal non-equilibrium power-law fluid saturated porous media. Chemical Engineering Communications, 2013, 200(2): 269-288. DOI:10.1080/00986445.2012.707710 (  0) 0) |
| [17] |
Tian X W, Wang P, Xu S M, et al. Comparison study of different viscous dissipation effects on forced convection heat transfer in a power-law fluid saturated porous medium. Journal of Porous Media, 2016, 19(10): 885-900. DOI:10.1615/JPorMedia.v19.i10.40 (  0) 0) |
| [18] |
Wang Y, Xia X T, Wang Y, et al. Using proper orthogonal decomposition to solve heat transfer process in a flat tube bank fin heat exchanger. Advances in Geo-Energy Research, 2017, 1(3): 158-170. DOI:10.26804/ager.2017.03.03 (  0) 0) |
| [19] |
Chamkha A J. Flow of two-immiscible fluids in porous and nonporous channels. Journal of Fluids Engineering, 2000, 122(1): 117-124. DOI:10.1115/1.483233 (  0) 0) |
| [20] |
Seyedi S H, Saray B N, Chamkha A J. Heat and mass transfer investigation of MHD Eyring-Powell flow in a stretching channel with chemical reactions. Physica A: Statistical Mechanics and its Applications, 2020, 544(15): 124109. DOI:10.1016/j.physa.2019.124109 (  0) 0) |
| [21] |
Anvari A R, Javaherdeh K, Emami-Meibodi M. Investigation of heat transfer and pressure drop of non-Newtonian nanofluid performance through micro channels heat exchanger (MCHE) in cross-flow configuration. Journal of Nanofluids, 2019, 8(3): 631-639. DOI:10.1166/jon.2019.1600 (  0) 0) |
| [22] |
Toghraie D, Mashayekhi R, Arasteh H, et al. Two-phase investigation of water-Al2O3 nanofluid in a micro concentric annulus under non-uniform heat flux boundary conditions. International Journal of Numerical Methods for Heat and Fluid Flow, 2019, 30(4): 1795-1814. DOI:10.1108/HFF-11-2018-0628 (  0) 0) |
| [23] |
Tian X W, Hang K, Zhang D G, et al. Review on convection heat transfer characteristics of nano-magnetic fluid coupled metal foam. Modern Chemical Industry, 2020, 40(9): 51-55. DOI:10.16606/j.cnki.issn0253-4320.2020.09.011 (  0) 0) |
| [24] |
Nakayama A, Shenoy A V. Non-Darcy forced convective heat transfer in a channel embedded in a non-Newtonian inelastic fluid-saturated porous medium. Canadian Journal of Chemical Engineering, 1993, 71(1): 168-173. DOI:10.1002/cjce.5450710122 (  0) 0) |
| [25] |
Lu Y H, Duan G L. Analytical solution of velocity distribution and temperature distribution of porous media plate channel and air channel. Journal of Engineering Thermophysics, 2018, 39(3): 633-639. (in Chinese) (  0) 0) |
| [26] |
Nield D A, Kuznetsov A V, Xiong M. Effects of viscous dissipation and flow work on forced convection in a channel filled by a saturated porous medium. Transport in Porous Media, 2004, 56(3): 351-367. DOI:10.1023/B:TIPM.0000026087.77213.c8 (  0) 0) |
| [27] |
Chen G M, Tso C P. Effects of viscous dissipation on forced convective heat transfer in a channel embedded in a power-law fluid saturated porous medium. International Communications in Heat and Mass Transfer, 2011, 38(1): 57-62. DOI:10.1016/j.icheatmasstransfer.2010.10.004 (  0) 0) |
| [28] |
Yang K, Vafai K. Analysis of heat flux bifurcation inside porous media incorporating inertial and dispersion effects-An exact solution. International Journal of Heat & Mass Transfer, 2011, 54(25-26): 5286-5297. DOI:10.1016/j.ijheatmasstransfer.2011.08.014 (  0) 0) |
| [29] |
Hung Y M, Tso C P. Effects of viscous dissipation on fully developed forced convection in porous media. International Journal of Heat and Mass Transfer, 2009, 36(6): 597-603. (  0) 0) |
 2022, Vol. 29
2022, Vol. 29


