2. School of Mechanical Science and Engineering, Huazhong University of Science and Technology, Wuhan 430074, China
The sound absorption technology which can improve the sound absorption effect through reducing the exhaust flow rate has been studied extensively and deeply in recent years. Munjal[1] analyzed the attenuation characteristic of expansion chamber with external intubation. Isshiki et al.[2] studied the relationship between current speed and acoustic characteristics through the method of numerical simulation. In 2009, Wu et al.[3] raised a new type of counteract and self-cancelling sound absorption structure and simulation and carried out experimental test. The result showed that the new sound absorption structure had a good sound absorption effect. Through the studies on reverse hedging new sound absorption structure by Shao[4], the specific parameters of silencing unit had a great impact on comprehensive ability. The study of Song et al.[5] on aerodynamic performance of split opposed muffler found that the speed of the air in split opposed muffler has decreased greatly after hedging so that the re-created noise decreased greatly. Through the study of Zhang et al.[6] on a kind of cone ring shunt sound absorption structure, it is found that the size of cone ring and cone angle is an important factor which influences the sound absorption ability. Yasuda et al.[7] proposed a simplified model based on the theory of one-dimensional CFD model, and simulated the tailpipe noise of exhaust muffler, and verified the results through experiments. Fan and Ji[8] developed a one-dimensional analysis method based on the transfer matrix method to analyze the sound attenuation characteristics of the three-pass muffler, and verified it through experiments. The results show that the average flow rate increases the transmission loss of the muffler at the pass frequency and reduces the sound attenuation in the intermediate frequency range. Bucciarelli et al.[9] analyzed the parameters of the muffler structure of the multilayer microperforated plate by means of the electro-acoustic equivalent circuit method, studied the relationship between the sound absorption performance of the multilayer microperforated plate and the structural parameters, which were verified by the impedance tube experiment. Carbajo et al.[10] proposed a kind of muffler with inclined hole for multi-layer perforated plate. The acoustic performance of inclined hole multi-layer perforated plate was predicted by fluid equivalence theory, and the predicted results were verified by experiments. Zhou et al.[11] studied the low-frequency sound absorption characteristics of double-layer perforated plate with fixed cavity under the action of biased current, and the results show that the velocity of biased current is the key parameter to determine the sound absorption performance of double-layer perforated plate structure. A fully coupled modal formula was proposed by Bravo[12]. Based on the proposed fully coupled modal formula, the effects of structural resonance on the absorption coefficient and transfer loss of multi-layer perforated plates were predicted and verified by experiments.
It can be seen that the sound absorption method based on shunt and offset has been widely studied in recent years. In this paper, a sound absorption method is proposed to reduce the airflow velocity and improve the sound absorption effect by offsetting the airflow in the perforated pipe. The flow velocity of the airflow after the offset was studied, and various factors affecting the transmission loss were analyzed, and the transmission loss of the whole structure was calculated by MATLAB. Finally, the important parameters affecting the transmission loss and the change of the transmission loss when the airflow velocity increases were verified by experiments.
1 Theoretical ModelFig. 1 shows the overall structure. The main components are cone-shaped splitter unit and perforated pipe. The diameter and length of the straight pipe from the entrance are d1 and L1, respectively. The length of the cone-shaped splitter unit is L2, and its cone angle is α. The length of the perforated pipe is L3, and its diameter is d2, The diameter of the external cavity is d3. The diameter of the straight pipe from the exit is d4. The length of both ends of the perforation segment is L5 and L6, respectively. The sound pressure of perforated pipe*s the external cavity is p1a, its particle vibration velocity is u1a. The sound pressure of perforated pipe*s the inner cavity is p1b, and its particle vibration velocity is u1b.
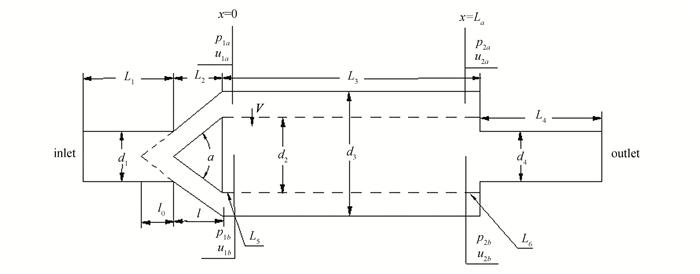
|
Fig.1 Diagram of overall structure |
1.1 Principle of Interference Muffling
The principle of noise attenuation is shown in Fig 2. When the sound wave enters the muffler from the inlet, the sound wave travels along the X-axis. When the sound wave reaches the hole in the perforated tube, the sound wave travels through the hole to the inside of the perforated tube. At this point, the sound wave begins to travel along the Y-axis. Since the structure of the muffler is axisymmetric, when the sound wave propagates along the Y direction, it has the same frequency with the sound wave of the opposite hole, and the phase difference is 1800. At this time, the two sound waves are interfered and silenced. After the interference and silencing, the sound energy is converted into heat energy, so as to achieve the effect of silencing.
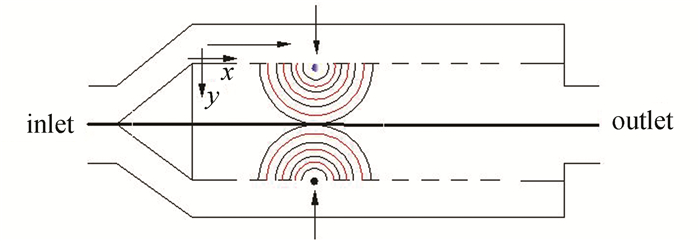
|
Fig.2 Schematic chart of Interference muffler |
1.2 Division of Anechoic Sub Units
As shown in Fig. 3, 1-2 is straight tube unit, 2-3 is conical shunt sub unit, 3-4 is perforated pipe, 4 is shrinking sub unit, 4-5 is straight tube unit. According to the propagation path of sound wave, the anechoic sub units among 1-5 are series connection. It comes to a conclusion that the transfer matrix of the whole sound absorption structure is as follows:
| $ \boldsymbol{T}=\boldsymbol{T}_{12} \cdot \boldsymbol{T}_{23} \cdot \boldsymbol{T}_{34} \cdot \boldsymbol{T}_{4} \cdot \boldsymbol{T}_{45} $ | (1) |
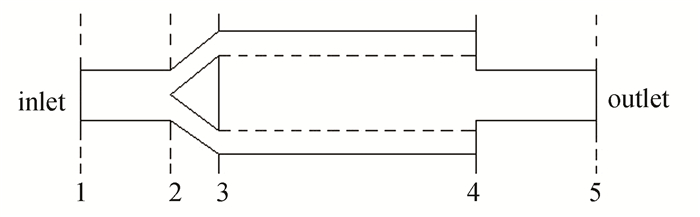
|
Fig.3 Diagram of unit division |
In the formula, T12 is 1-2 part of the transfer matrix, T23 is 2-3 part of the transfer matrix, T34 is 3-4 part of the transfer matrix. T4 is 4 part of the transfer matrix, and T45 is 4-5 part of the transfer matrix.
1.3 Calculation of Transfer Matrix of Anechoic Sub UnitsThe transfer matrix of the inlet straight pipe anechoic sub-unit is[13]
| $ \boldsymbol{T}_{12}=\left[\begin{array}{cc} \cos \left(\frac{k L_{1}}{1-M^{2}}\right) & \mathrm{jsin} \left(\frac{k L_{1}}{1-M^{2}}\right) \\ \mathrm{jsin}\left(\frac{k L_{1}}{1-M^{2}}\right) & \cos \left(\frac{k L_{1}}{1-M^{2}}\right) \end{array}\right] $ | (2) |
where k is the wave number and M is the Mach number.
The transfer matrix of the conical shunt sub unit is as follows[14]
| $ \boldsymbol{T}_{23}=\left[\begin{array}{ll} A_{1} & B_{1} \\ C_{1} & D_{1} \end{array}\right] $ | (3a) |
| $ A_{1}=\frac{\sqrt{l_{0}+2 l}}{l_{0}}(\cos \beta-\mu \sin \beta) $ | (3b) |
| $ B_{1}=\frac{\mu \omega \rho \sqrt{\left(l_{0}+2 l\right)^{3}}}{\sqrt{l_{0}}} \sin \beta $ | (3c) |
| $ \begin{gathered} C_{1}=\frac{\sqrt{l_{0}+2 l}}{\mathrm{j} \omega \rho \sqrt{l_{0}^{3}}}\left(\left(1-\mu \sqrt{1+k^{2} l_{0}^{2}}\right) \cos \beta-(\mu+\right. \\ \left.\left.\sqrt{1+k^{2} l_{0}^{2}}\right) \sin \beta\right) \end{gathered} $ | (3d) |
| $ D_{1}= \frac{\mu \sqrt{\left(l_{0}+2 l\right)^{3}}}{\mathrm{j} \sqrt{l_{0}^{3}}}\left(\sin \beta+\sqrt{1+k^{2} l_{0}^{2}} \cos \beta\right) $ | (3e) |
| $ \beta=l \sqrt{k^{2}+\left(l_{0}+2 l\right)^{-2}} $ | (3f) |
| $ \mu=\frac{\left(l_{0}+2 l\right) \sqrt{k^{2}\left(l_{0}+2 l\right)^{2}+1}}{k^{2}\left(l_{0}+2 l\right)^{3}+l_{0}} $ | (3g) |
where l is the length of the conical ring in the conical shunt sub unit, and l0 is the distance between the entrance of the conical ring in the conical shunt sub unit and the vertex of the reverse extension line of the conical line.
The application of transfer matrix method is based on plane wave theory, so it is assumed that sound waves are plane waves and the propagation process is adiabatic. According to the law of conservation of mass and the law of conservation of momentum in fluid mechanics, the continuity equation and motion equation of the external cavity can be derived as follows[15]:
| $ \rho_{0} \frac{\partial u_{1 a}}{\partial x}+\frac{4 \rho_{0} v}{d_{2}}+\frac{\partial \rho_{1 a}}{\partial t}=0 $ | (4) |
| $ \rho_{0} \frac{\partial u_{1 a}}{\partial t}+\frac{\partial p_{1 a}}{\partial x}=0 $ | (5) |
Inside the perforated pipe, there are
| $ \rho_{0} \frac{\partial u_{1 b}}{\partial x}+\frac{4 \rho_{0} v d_{3}}{d_{3}^{2}-d_{2}^{2}}+\frac{\partial \rho_{1 b}}{\partial t}=0 $ | (6) |
| $ \rho_{0} \frac{\partial u_{1 b}}{\partial t}+\frac{\partial p_{1 b}}{\partial x}=0 $ | (7) |
where v is the velocity flowing into the inner cavity of the perforated pipe, t is time, ρ is the density of gas in the process of sonic wave propagation, and ρ0 is the density of gas of static state.
Because the process of the sonic wave propagation is an adiabatic process, it can be inferred that[13]
| $ p(x)=\rho(x) c_{0}^{2} $ | (8) |
where c0 is sound velocity.
The relative acoustic impedance rate of the perforated pipe is[13]
| $ Z=\frac{p_{1 a}(x)-p_{1 b}(x)}{\rho_{0} c_{0} v(x)} $ | (9) |
Let p(x, t)=p(x)ejωt. It can be concluded that
| $ v(x, t)=\frac{p_{1 a}(x)-p_{1 b}(x)}{\rho_{0} c_{0} Z} \mathrm{e}^{\mathrm{j} \omega t} $ | (10) |
According to the empirical formula obtained by measuring the acoustic impedance of the perforated plate by Lee and Ih[16] under the condition of flow, there is
| $ Z=R_{h}+\mathrm{j} X_{h} $ | (11) |
| $ \begin{aligned} R_{h}=& a_{0}\left(1+a_{1}\left|f-f_{0}\right|\right)\left(1+a_{2} M\right) \cdot \\ &\left(1+a_{3} d_{h}\right)\left(1+a_{4} t_{w}\right) \end{aligned} $ | (12) |
| $ X_{h}=b_{0}\left(1+b_{1} d\right)\left(1+b_{2} t_{w}\right)\left(1+b_{3} M\right)\left(1+b_{4} f\right) $ | (13) |
| $ f_{0}=412(1+104 M) /(1+274 d) $ | (14) |
where a0=3.94×10-4, a1=7.84×10-3, a2=14.9, a3=296, a4=-127, b0=-6×10-3, b1=194, b2=432, b3=-17.2, b4=-6.62×10-3, Rh is the acoustic resistance, Xh is the acoustic reactance, d is the diameter of the perforated pipe, and tw is the thickness of the perforated pipe.
According to mass conservation equation, momentum equation and motion equation, it can be deduced that the acoustics equation of the perforated pipe is[17]
| $ \begin{aligned} \left[\begin{array}{c} p^{\prime}{}_{1 a} \\ \rho_{0} c_{0} u_{1 a}^{\prime} \\ p^{\prime}{}_{2 a} \\ \rho_{0} c_{0} u_{2 a}^{\prime} \end{array}\right]&=\left[\begin{array}{cccc} 0 & -\mathrm{j} k & 0 & 0 \\ -\mathrm{j} a_{1} / k & 0 & -\mathrm{j} a_{2} / k & 0 \\ 0 & 0 & 0 & -\mathrm{j} k \\ -\mathrm{j} a_{3} / k & 0 & -\mathrm{j} a_{4} / k & 0 \end{array}\right]\cdot \\ &{\left[\begin{array}{c} p_{1 a} \\ \rho_{0} c_{0} u_{1 a} \\ p_{2 a} \\ \rho_{0} c_{0} u_{2 a} \end{array}\right]} \end{aligned} $ | (15) |
where
| $ a_{1}=k^{2}-\frac{4 \mathrm{j} k}{d_{2} Z}, a_{2}=\frac{4 \mathrm{j} k}{d_{2} Z} $ | (16a) |
| $ a_{3}=\frac{4 \mathrm{j} k d_{2}}{\left(d_{3}^{2}-d_{2}^{2}\right) Z}, a_{4}=k^{2}-a_{3} $ | (16b) |
| $ \boldsymbol{A}=\left[\begin{array}{cccc} 0 & -\mathrm{j} k & 0 & 0 \\ -\mathrm{j} a_{1} / k & 0 & -\mathrm{j} a_{2} / k & 0 \\ 0 & 0 & 0 & -\mathrm{j} k \\ -\mathrm{j} a_{3} / k & 0 & -\mathrm{j} a_{4} / k & 0 \end{array}\right] $ | (17) |
The solution of Eq. (15) can be expressed as
| $ \left[\begin{array}{c} p_{1 a} \\ \rho_{0} c_{0} u_{1 a} \\ p_{2 a} \\ \rho_{0} c_{0} u_{2 a} \end{array}\right]=[\boldsymbol{\varOmega}] \cdot\left[\begin{array}{c} C_{1} \mathrm{e}^{\lambda_{1} x} \\ C_{2} \mathrm{e}^{\lambda_{2} x} \\ C_{3} \mathrm{e}^{\lambda_{3} x} \\ C_{4} \mathrm{e}^{\lambda_{4} x} \end{array}\right] $ | (18) |
where [Ω] is a matrix composed of eigenvectors of matrix A, λ is the eigenvalue of matrix A.
From Eq. (18), the relationship between sound pressure and particle vibration velocity at x=0 and x=La can be obtained:
| $ \left[\begin{array}{c} p_{1 a}(0) \\ \rho_{0} c_{0} u_{1 a}(0) \\ p_{2 a}(0) \\ \rho_{0} c_{0} u_{2 a}(0) \end{array}\right]=[\boldsymbol{R}] \cdot\left[\begin{array}{c} p_{1 a}\left(L_{a}\right) \\ \rho_{0} c_{0} u_{1 a}\left(L_{a}\right) \\ p_{2 a}\left(L_{a}\right) \\ \rho_{0} c_{0} u_{2 a}\left(L_{a}\right) \end{array}\right] $ | (19) |
where [R]=[Ω][E][Ω]-1
| $ \boldsymbol{E}=\left[\begin{array}{cccc} \mathrm{e}^{-\lambda_{1} L_{a}} & 0 & 0 & 0 \\ 0 & \mathrm{e}^{-\lambda_{2} L_{a}} & 0 & 0 \\ 0 & 0 & \mathrm{e}^{-\lambda_{3} L_{a}} & 0 \\ 0 & 0 & 0 & \mathrm{e}^{-\lambda_{4} L_{a}} \end{array}\right] $ | (20) |
In x=0 and and in the perforated pipe at a random position x=La place, it has a boundary condition[18]:
| $ \left\{\begin{array}{l} \rho_{0} c_{0} u_{1 b}(0)=-\mathrm{jtan}\left(k L_{a}\right) p_{1 b}(0) \\ \rho_{0} c_{0} u_{1 b}\left(L_{5}\right)=\operatorname{jtan}\left(k L_{6}\right) p_{1 b}\left(L_{3}\right) \end{array}\right. $ | (21) |
It can be inferred that
| $ \begin{aligned} p_{1 a}(0)=& R_{11} p_{1 a}\left(L_{a}\right)+R_{12} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{13}+\operatorname{jtan}\left(k L_{6}\right) R_{14}\right) p_{1 b}\left(L_{a}\right) \end{aligned} $ | (22a) |
| $ \begin{aligned} \rho_{0} c_{0} u_{1 a} &(0)=R_{21} p_{1 a}\left(L_{a}\right)+R_{22} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{23}+\operatorname{jtan}\left(k L_{6}\right) R_{24}\right) P_{1 b}\left(L_{a}\right) \end{aligned} $ | (22b) |
| $ \begin{aligned} p_{1 b}(0)=& R_{31} p_{1 a}\left(L_{a}\right)+R_{32} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{33}+\mathrm{jtan} \left(k L_{6}\right) R_{34}\right) p_{1 b}\left(L_{a}\right) \end{aligned} $ | (22c) |
| $ \begin{aligned} \rho_{0} c_{0} &u_{1 b}(0)=R_{41} p_{1 a}\left(L_{a}\right)+R_{42} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{43}+\operatorname{jtan}\left(k L_{6}\right) R_{44}\right) P_{1 b}\left(L_{a}\right) \end{aligned} $ | (22d) |
| $ \begin{aligned} p_{1 a}(0)=& R_{11} p_{1 a}\left(L_{a}\right)+R_{12} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{13}+\mathrm{jtan} \left(k L_{6}\right) R_{14}\right) p_{1 b}\left(L_{a}\right) \end{aligned} $ | (23a) |
| $ \begin{aligned} \rho_{0} c_{0} &u_{1 a}(0)=R_{21} p_{1 a}\left(L_{a}\right)+R_{22} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+ \\ &\left(R_{23}+\operatorname{jtan}\left(k L_{6}\right) R_{24}\right) p_{1 b}\left(L_{a}\right) \end{aligned} $ | (23b) |
| $ \begin{aligned} p_{1 b}(0)=& R_{31} p_{1 a}\left(L_{a}\right)+R_{32} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+\\ &\left(R_{33}+\mathrm{jtan} \left(k L_{6}\right) R_{34}\right) p_{1 b}\left(L_{a}\right) \end{aligned} $ | (23c) |
| $ \begin{array}{l} -\operatorname{jtan}\left(k L_{5}\right) p_{1 b}(0)=R_{41} p_{1 a}\left(L_{a}\right)+R_{42} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right)+ \\ \ \ \ \ \ \ \ \ \ \ \ \left(R_{43}+\operatorname{jtan}\left(k L_{6}\right) R_{44}\right) p_{1 b}\left(L_{a}\right) \end{array} $ | (23d) |
Subtract Eq. (23). There is
| $ \begin{aligned} &\left(R_{41}+\mathrm{j} \tan \left(k L_{5}\right) R_{31}\right)+\left(R_{42}+\mathrm{jtan} \left(k L_{5}\right) R_{32}\right) \\ &\ \ \rho_{0} c_{0} U_{1 a}\left(L_{3}\right)+\left[R_{43}+\mathrm{jtan} \left(k L_{6}\right) R_{44}+\right. \\ &\left.\quad \ \ \mathrm{jtan} \left(k L_{5}\right)\left(R_{33}+\mathrm{jtan} \left(k L_{6}\right) R_{34}\right)\right] P_{1 b}\left(L_{a}\right)=0 \end{aligned} $ | (24) |
Let
| $ \begin{aligned} Q_{1}=& R_{41}+\mathrm{jtan} \left(k L_{5}\right) R_{31} \\ Q_{2}=& R_{42}+\mathrm{jtan} \left(k L_{5}\right) R_{32} \\ Q_{3}=& R_{43}+\mathrm{jtan} \left(k L_{6}\right) R_{44}+\\ & \mathrm{jtan} \left(k L_{5}\right)\left(R_{33}+\mathrm{jtan} \left(k L_{6}\right) R_{34}\right) \end{aligned} $ | (25) |
Eq. (24) can be written as
| $ p_{1 b}\left(L_{a}\right)=-\frac{Q_{1}}{Q_{3}} p_{1 a}\left(L_{a}\right)-\frac{Q_{2}}{Q_{3}} \rho_{0} c_{0} u_{1 a}\left(L_{a}\right) $ | (26) |
Substitute Eq. (25) into Eq. (23). It can be inferred that
| $ \begin{aligned} p_{1 a} &=\left[R_{11}-\frac{\left(R_{13}-\mathrm{jtan} \left(k L_{6}\right) T_{14}\right) Q_{1}}{Q_{3}}\right] p_{1 a}(L)+\\ & {\left[R_{12}-\frac{\left(R_{13}-\mathrm{jtan} \left(k L_{6}\right) T_{14}\right) Q_{2}}{Q_{3}}\right] \rho_{0} c_{0} U_{1 a}(L) } \end{aligned} $ | (27a) |
| $ \begin{aligned} \rho_{0} c_{0} U_{1 a}&(0)=\left[R_{21}-\frac{\left(R_{23}-\mathrm{jtan} \left(k L_{6}\right) T_{24}\right) Q_{1}}{Q_{3}}\right] p_{1 a}(L)+ \\ &{\left[R_{22}-\frac{\left(R_{23}-\mathrm{jtan} \left(k L_{6}\right) T_{24}\right) Q_{2}}{Q_{3}}\right] \rho_{0} c_{0} U_{1 a}(L)} \end{aligned} $ | (27b) |
Therefore, it indicates that the transfer matrix of the perforated pipe is
| $ \left[\begin{array}{c} p_{1 a}(0) \\ \rho_{0} c_{0} u_{1 a}(0) \end{array}\right]=\left[\begin{array}{cc} A_{2} & B_{2} \\ C_{2} & D_{2} \end{array}\right]\left[\begin{array}{c} p_{1 a}(L) \\ \rho_{0} c_{0} u_{1 a}(L) \end{array}\right] $ | (28) |
where
| $ A_{2} =R_{11}-\frac{\left(R_{13}+\mathrm{jtan} \left(k L_{6}\right) R_{14}\right) Q_{1}}{Q_{3}} $ | (29a) |
| $ B_{2} =R_{12}-\frac{\left(R_{13}+\mathrm{jtan} \left(k L_{6}\right) R_{14}\right) Q_{2}}{Q_{3}} $ | (29b) |
| $ C_{2} =R_{21}-\frac{\left(R_{23}+\mathrm{jtan} \left(k L_{6}\right) R_{24}\right) Q_{1}}{Q_{3}} $ | (29c) |
| $ D_{2} =R_{22}-\frac{\left(R_{23}+\mathrm{jtan} \left(k L_{6}\right) R_{24}\right) Q_{1}}{Q_{3}} $ | (29d) |
It can be concluded that the transfer matrix of Eq. (28) is T34.
The transfer matrix of the shrinking sub units is[13]
| $ \boldsymbol{T}_{4}=\left[\begin{array}{cc} \frac{1}{2}\left[\mathrm{e}^{\mathrm{j} k^{l^{\prime}}}+\mathrm{e}^{-\mathrm{j} k-l^{\prime}}\right] & \frac{\rho c_{0}}{2 S}\left[\mathrm{e}^{\mathrm{j} k^{\prime}}+\mathrm{e}^{-\mathrm{j} k^{-} l^{\prime}}\right] \\ \frac{S}{2 \rho c_{0}}\left[\mathrm{e}^{\mathrm{j} k^{l \prime}}+\mathrm{e}^{-\mathrm{j} k-l^{\prime}}\right] & \frac{1}{2}\left[\mathrm{e}^{\mathrm{j} k^{l^{\prime}}}+\mathrm{e}^{-\mathrm{j} k^{-} l^{\prime}}\right] \end{array}\right] $ | (30) |
where S and l' are the length and cross-section area of the contraction cavity.
| $ k^{+}=\frac{k}{1+M}, k^{-}=\frac{k}{1-M} $ | (31) |
The transfer matrix of the straight pipe anechoic sub units is[13]
| $ \boldsymbol{T}_{45}=\left[\begin{array}{cc} \cos \left(\frac{k L_{4}}{1-M^{2}}\right) & \mathrm{jsin} \left(\frac{k L_{4}}{1-M^{2}}\right) \\ \mathrm{jsin} \left(\frac{k L_{4}}{1-M^{2}}\right) & \cos \left(\frac{k L_{4}}{1-M^{2}}\right) \end{array}\right] $ | (32) |
The calculation formula of the transmission loss is[14]
| $ \mathrm{TL}=10 \lg \left(\frac{\left|A_{3}+m B_{3}+C_{3}+m D_{3}\right|^{2}}{4 m}\right) $ | (33) |
where A3, B3, C3, d3 are the transfer matrix elements, m is the ratio of exit area to entrance area.
According to the analysis above, the parameters influencing transmission loss are the length of both ends of perforated section L5 and L6, diameter of external chamber d2, diameter of perforated pipe d3, and the porosity ϕ The following calculates the changes of transmission loss curve when parameters change.
When the length of both ends of perforated section L5 and L6 changes, the changes of transmission loss curves are shown in Fig. 4.
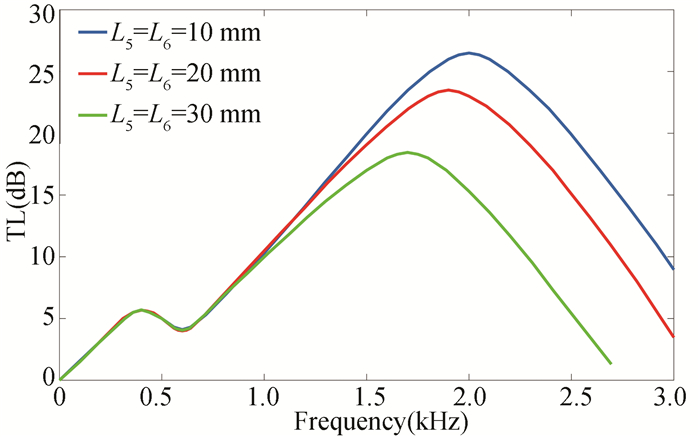
|
Fig.4 Overall transmission loss curves of divergent offset perforated pipe |
When the diameter of external chamber d3 remain the same, diameter of perforated pipe d2 changes. The changes of transmission loss curves are shown in Fig. 5.
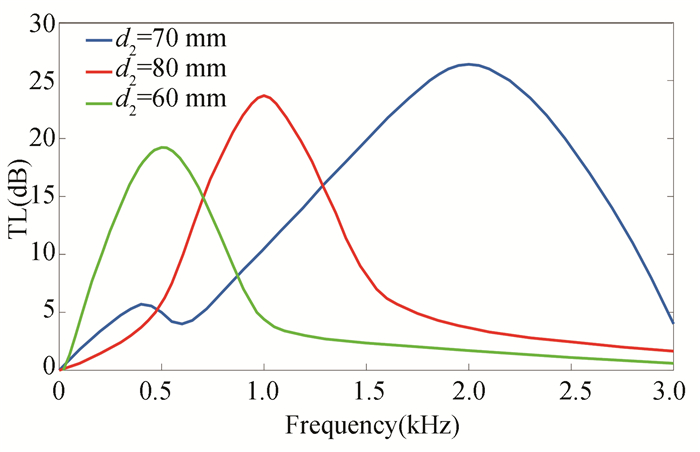
|
Fig.5 Overall transmission loss curves of divergent offset perforated pipe |
When the diameter of perforated pipe d2 remains the same, diameter of external chamber d3 changes. The changes of transmission loss curves are shown in Fig. 6.
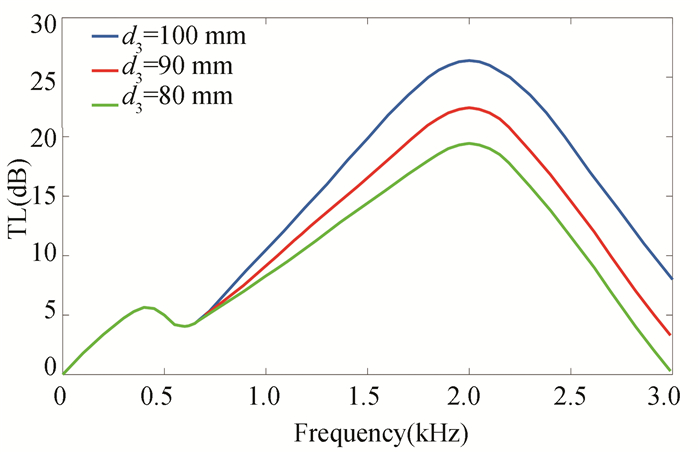
|
Fig.6 Overall transmission loss curves of divergent offset perforated pipe |
When the porosity ϕ hanges, the changes of transmission loss curves are shown in Fig. 7.
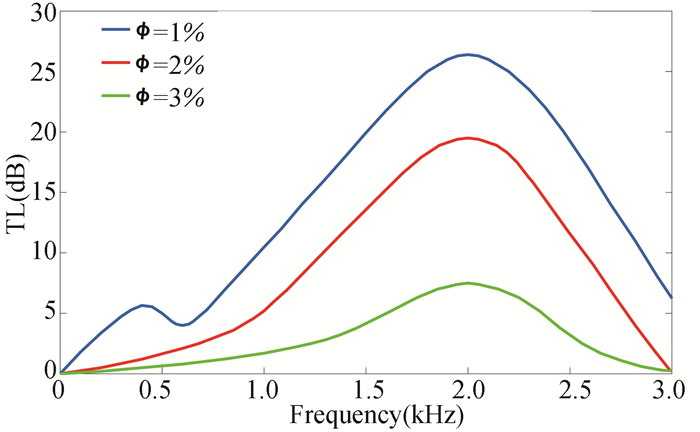
|
Fig.7 Overall transmission loss curves of divergent offset perforated pipe |
3 Experimental Verification 3.1 Experimental Methods
In the experiment of transmission loss, the two-load method is adopted, the sound source position is fixed, and the quadrupole parameters are solved by establishing equations by changing the impedance condition at the outlet.
As shown in Fig. 8, pi is the incident sound wave at the inlet, pr is the reflected sound wave at the inlet, pi1 is the incident sound wave at the outlet, and pr1 is the reflected sound wave at the outlet. The sound wave transmission relationship at both ends of the element to be tested is as follows:
| $ \left[\begin{array}{c} p_{i} \\ p_{r} \end{array}\right]=\left[\begin{array}{c} T_{11} & T_{12} \\ T_{21} & T_{22} \end{array}\right]\left[\begin{array}{c} p_{i 1} \\ p_{r 1} \end{array}\right] $ | (34) |
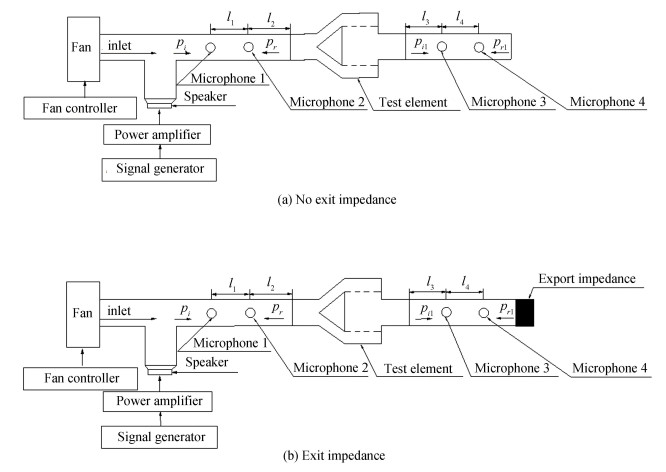
|
Fig.8 Perforated plate structure |
When the constraint condition is that the outlet is a non-reflective end and pr1=0, the calculation formula of transmission loss TL is as follows[19]:
| $ \mathrm{TL}=20 \lg \left(\frac{\left|p_{i}\right|}{\left|p_{i 1}\right|} \mid p_{r 1}=0\right)=20 \lg \left|T_{11}\right| $ | (35) |
In general, it is difficult to obtain the experimental condition that the outlet is a non-reflective end, so the outlet has two different impedance conditions, and two equations are obtained, and the simultaneous equations solve T11.When there is no impedance at the outlet, the sound wave transmission relationship at both ends of the element to be tested is as follows:
| $ \left[\begin{array}{c} p_{i a} \\ p_{r a} \end{array}\right]=\left[\begin{array}{c} T_{11} & T_{12} \\ T_{21} & T_{22} \end{array}\right]\left[\begin{array}{c} p_{i 1 a} \\ p_{r 1 a} \end{array}\right] $ | (36) |
When there is an impedance at the outlet, the sound wave transmission relationship at both ends of the element to be tested is as follows:
| $ \left[\begin{array}{c} p_{i b} \\ p_{r b} \end{array}\right]=\left[\begin{array}{c} T_{11} & T_{12} \\ T_{21} & T_{22} \end{array}\right]\left[\begin{array}{c} p_{i 1 b} \\ p_{r 1 b} \end{array}\right] $ | (37) |
With Eqs. (36) and (37), it can be figured out that
| $ T_{11}=\frac{p_{i a} p_{r 1 a}-p_{i b} p_{r 1 a}}{p_{i 1 a} p_{r 1 b}-p_{i 1 b} p_{r 1 a}} $ | (38) |
By changing the acoustic impedance at the outlet, the sound pressure values of two groups of incident waves and reflected waves are measured by four microphones, and the transmission loss TL can be solved by substituting the sound pressure values into the above formula.
3.2 Experimental ResultsWhen the length L5 and L6 at both ends of the perforation segment changes, the comparison of the transmission loss obtained in the experiment and the result calculated by the formula are shown in Fig. 9.
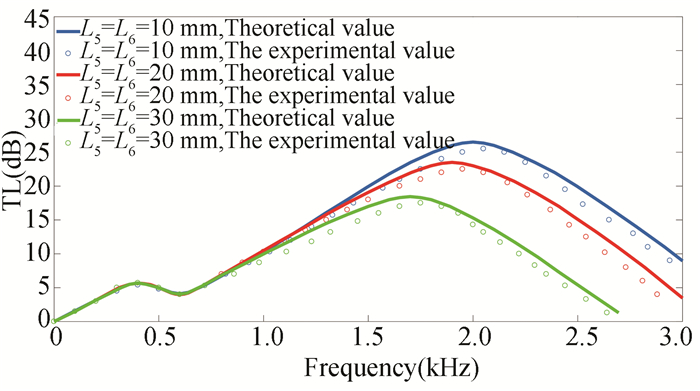
|
Fig.9 Comparison of transmission loss |
When diameter of the external cavity d3 remains the same, the diameter of the perforated pipe d2 changes. The comparison of the transmission loss obtained in the experiment and the result calculated by the formula are shown in Fig. 10.
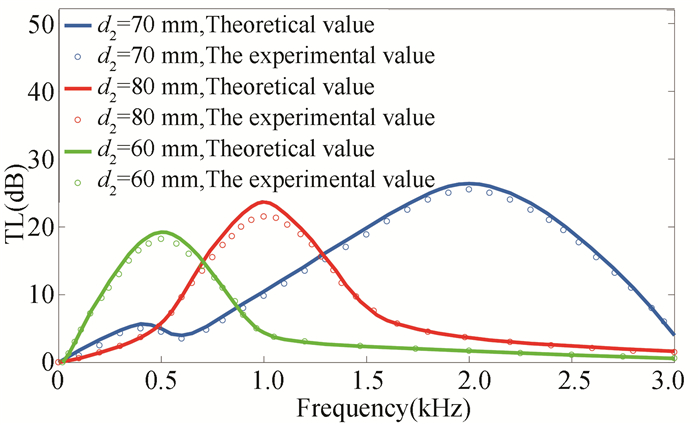
|
Fig.10 Comparison of transmission loss |
When diameter of the perforated pipe d2 remains the same, diameter of the external cavity d3 changes. The comparison of the transmission loss obtained in the experiment and the result calculated by the formula are shown in Fig. 11.
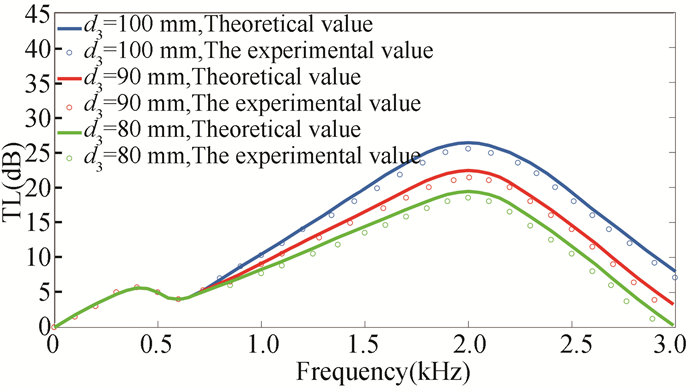
|
Fig.11 Comparison of transmission loss |
When the porosity ϕ hanges, the comparison of the transmission loss obtained in the experiment and the result calculated by the formula are shown in Fig. 12.
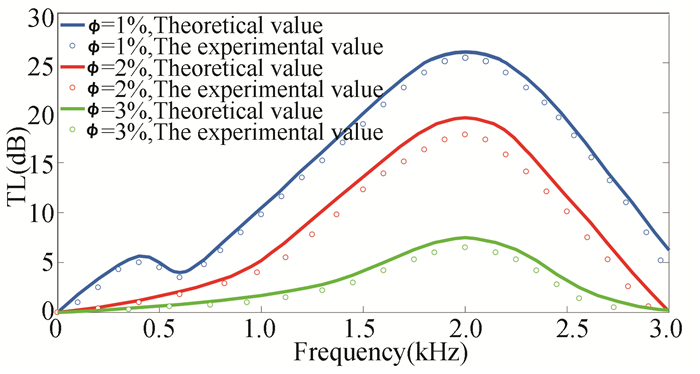
|
Fig.12 Comparison of transmission loss |
When the airflow velocity of the entrance changes, the comparison of the transmission loss obtained in the experiment and the result calculated by the formula are shown in Fig. 13.
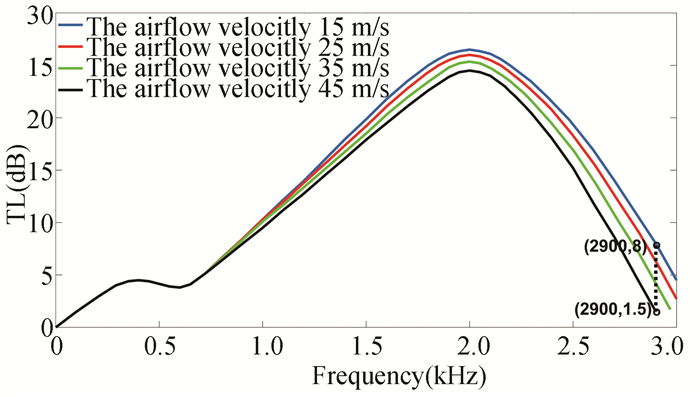
|
Fig.13 Comparison of transmission loss |
3.3 Analysis on Experimental Results
According to Fig. 8, when the length of both ends of perforated section L5 and L6 decreases, transmission loss increases. It is because with the increase of length of perforated pipe, the number of holes increases, and the effect of air flow hedge strengthens, which causes the transmission loss to increase.
According to Fig. 10, when the diameter of external chamber d3 remains the same, the diameter of perforated pipe d2 decreases gradually, the peak value of transmission loss moves to low frequency range, which results from the fact that as the diameter of perforated pipe decreases after the air flow hedge of the holes, the air flow speed increases, re-created noise gets higher, and it has little influence on low frequency range while has bigger influence on high frequency range.
According to Fig. 11, when the diameter d2 of the perforated pipe is constant and the diameter d3 of the external cavity decreases, the transmission loss decreases, which results from the fact that with the decrease of the diameter of the external cavity, the gap between the external cavity and the perforated pipe decreases, and the shunted airflow cannot be fully decelerated in the external cavity, and with the decrease of the diameter of the external cavity, the flow velocity of the shunted airflow will gradually increase, resulting in a decrease in transmission loss.
According to Fig. 12, when the porosity ϕ ncreases, the transmission loss decreases, which results from the fact that as the porosity ϕ increases, the acoustical mass in the small pore decreases, which makes the sound impedance of the perforated pipe decrease, thus reducing the sound absorption effect.
According to Fig. 13, when the airflow velocity of the entrance increases, the transmission loss decreases, which results from the fact that the diameter of the perforated tube, the length of the perforated section and porosity are fixed values. The hedging effect does not increase when it reaches the peak. When the the airflow velocity of the entrance increases, the regeneration noise increases, the transmission loss decreases, but the reduction range is smaller. However, it can still guarantee good functions of absorbent.
3.4 Analysis on Experimental ErrorThe overall trend of the experimental results is basically consistent with the theoretical calculation results, but there is some error. This is because during the experiment, due to the rough inner wall of the muffler sample, the transition area at the connection of each muffler unit failed to achieve smooth transition, resulting in resistance in these areas, affecting the muffler effect. Besides, due to the interference of the external environment, there will be a certain amount of background noise. The existence of background noise will reduce the measurement accuracy.
4 ConclusionsThe air in external chamber slows down fully after the conical shunt units split it. After this, it goes hedging through perforated pipe. The flow speed after hedging decreases greatly, which decreases the generation of re-created noise. During the hedging process of the air, the acoustic energy attenuated mutually, thus strengthens the sound absorption effect.
The variables that affect the transmission loss are verified by experiments. The experimental results show that the length of both ends of the perforated section L5 and L6, the diameter d2 of the perforated pipe, the diameter d3 of the external cavity, and porosity ϕ have significant effects on the transmission loss. Only by reasonably selecting the above structural parameters can better sound absorption effect be guaranteed.
When the inlet air velocity increases from 15 m/s to 45 m/s, the maximum reduction of transmission loss is 6.5 dB in the range of 0-3000 Hz, which proves that the structure has a good effect of sound absorption.
| [1] |
Munjal M L. Recent advances in muffler acoustics. International Journal of Acoustics and Vibration, 2013, 18(2): 71-85. DOI:10.20855/ijav.2013.18.2321 (  0) 0) |
| [2] |
Isshiki Y, Shimamoto Y, Wakisaka T. Analysis of acoustic characteristics and pressure losses in intake silencers by numerical simulation. Transactions of the Japan Society of Mechanical Engineers Part B, 1993, 59(559): 332-337. (  0) 0) |
| [3] |
Wu X, Wu P, Shao Y L. Study on new exhaust silencer of single cylinder diesel engine. Journal of Inner Mongolia Agricultural University (Science and Technology), 2009, 30(2): 188-190. (  0) 0) |
| [4] |
Shao Y L. A study on exhaust muffler using a mixture of counter-phase counteract and split-gas rushing. Procedia Engineering, 2011, 15: 4409-4413. DOI:10.1016/j.proeng.2011.08.828 (  0) 0) |
| [5] |
Song B G, Wu P, Ma Y H, et al. Resistame and acoustic performance study on performance of a new split-Stream exhaust muffler. China Sciencepaper, 2016, 11(23): 2735-2739. (  0) 0) |
| [6] |
Zhang Y A, Ma Y H, Wu P, et al. Prediction and analysis on acoustic properties of a specially-structured muffler unit. Transactions of CSICE, 2017, 35(4): 362-368. DOI:10.16236/j.cnki.nrjxb.201704051 (  0) 0) |
| [7] |
Yasuda T, Wu C Q, Nakagawa N, et al. Predictions and experimental studies of the tail pipe noise of an automotive muffler using a one dimensional CFD model. Applied Acoustics, 2010, 71(8): 701-707. DOI:10.1016/j.apacoust.2010.03.001 (  0) 0) |
| [8] |
Fan Y L, Ji Z L. Three-pass mufflers with perforated inlet/outlet tubes. Applied Acoustics, 2019, 156: 217-228. DOI:10.1016/j.apacoust.2019.07.018, (  0) 0) |
| [9] |
Bucciarelli F, Fierro G P M, Meo M. A multi-layer micro-perforated panel prototype for broadband sound absorption at low frequencies. Applied Acoustics, 2019, 146: 134-144. DOI:10.1016/j.apacoust.2018.11.014 (  0) 0) |
| [10] |
Carbajo J, Mosanenzadeh G S, Kim S, et al. Multi-layer perforated panel absorbers with oblique perforations. Applied Acoustics, 2020, 169: 107496. DOI:10.1016/j.apacoust.2020.107496 (  0) 0) |
| [11] |
Zhou H, Meng S, Tao C F, et al. Low-frequency sound absorptive properties of dual perforated plates under bias flow. Applied Acoustics, 2019, 146: 420-428. DOI:10.1016/j.apacoust.2018.11.027 (  0) 0) |
| [12] |
Bravo T. Enhancing sound absorption and transmission through flexible multi-layer micro-perforated structures. The Journal of the Acoustical Society of America, 2013, 134(5): 3663. DOI:10.1121/1.4821215 (  0) 0) |
| [13] |
Ji Z L. Acoustic Theories and Design about Silencer. Beijing: Science Press, 2015.
(  0) 0) |
| [14] |
Liu C L. Structure Design and Optimization of a Multi-layer Micro-perforated Tube Muffler Based on the Sound Transfer Matrix Method. Liuzhou: Guangxi University of Science and Technology, 2018.
(  0) 0) |
| [15] |
Zhang M H, Zuo S G, Xiang L Y, et al. Transmission loss theoretical calculation and analysis of double-layer micro-perforated muffler. Journal of Central South University (Science and Technology), 2015, 46(2): 505-511. DOI:10.11817/j.issn.1672-7207.2015.02.019 (  0) 0) |
| [16] |
Lee S H, Ih J G. Empirical model of the acoustic impedance of a circular orifice in grazing mean flow. The Journal of the Acoustical Society of America, 2003, 114(1): 98-113. DOI:10.1121/1.1581280 (  0) 0) |
| [17] |
Munjal M L. Acoustics of Ducts and Mufflers, Second Edition. Chichester, UK: John Wiley, 2014.
(  0) 0) |
| [18] |
Guo R, Tang W B, Zhu W W. Comparison of 1D transfer matrix method and finite element method with test for acoustic performance of multi-chamber perforated resonator. Applied Acoustics, 2016, 112: 140-146. DOI:10.1016/j.apacoust.2016.05.018 (  0) 0) |
| [19] |
Shi X F, Mak C M. Sound attenuation of a periodic array of micro-perforated tube mufflers. Applied Acoustics, 2017, 115: 15-22. DOI:10.1016/j.apacoust.2016.08.017 (  0) 0) |
 2022, Vol. 29
2022, Vol. 29


