Metallic glasses have drawn wide attention for more than fifty years since originally synthesized in 1960. Much effort has been devoted to the study of amorphous alloys because they own excellent properties[1-3]. However, there are still many longstanding issues to be solved, such as the influence of composition on the structure of amorphous alloys, and the nature of glass formation[4-8]. For the sake of understanding the nature of the amorphous alloys, research has been conducted on the influence of composition on the structure and properties. There were many simulation and experiment results in the last few decades. Zhang et al.[9] found that Zr64Cu26Al10 alloy exhibited better compression performance compared with Zr45Cu45Al10 by conducting molecular dynamics (MD) simulations. Wong[10] revealed that the ZrCuAl ternary alloys had more icosahedra when the composition of Zr was less than that of Cu by conducting the simulations on the ZrxCu90-xAl10 system. Yu[11] used a novel approach to forecast the glass forming ability of different alloys and they successfully applied it to the (Zr0.5Cu0.5)100-xAlx ternary system. Deb Nath[12] found that Zr composition had great influence on the mechanical properties of the ZrCuAl ternary alloys. Huang et al.[13] investigated the local atomic structures of Cu50Zr45Al5 and Zr50Cu45Al5 amorphous alloys by conducting ab initio MD simulations. The difference in the atomic chemical property between Zr and Cu atom contributed to the difference in the GFA. MD simulations have been conducted on the Zr50Cu50-xAlx alloys and Celtek et al.[14] found that Al played a great important role in glass transition and icosahedral ordering. Zhao[15-16] reported that ZrCuAl amorphous alloys with high Zr composition packed more loosely by analyzing the structure and energy distribution by conducting the MD simulations. Sha et al.[17] provided the straightforward evidence that the electronic stability of the basic cluster in CuZrAl amorphous alloys is the origin of GFA. Wang[18] found that as Cu content increases, the number of the ordered coordination polyhedron increases monotonically. Thus it is highly necessary to study the influence of the composition on the final structures and properties of the amorphous alloys.
In this paper, investigations were carried out in the ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) ternary alloys. For the sake of clarifying the influence of the composition on these amorphous alloys, the coordination polyhedron structure, the coordination polyhedron connection structure and the energy distribution of these structures in the ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) ternary alloys were described.
1 Simulation and Analysis 1.1 Simulation MethodThe study was conducted using classic MD simulations[19]. In our work, there were 16000 atoms in the unit cell, which were randomly arranged. The initial configuration of the ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys was heated up to 2000 K firstly, and then held for 10 ns. The liquid was cooled to 300 K with the speed of 5×1010 K/s.
1.2 Energy AnalysisEp, C represents the cluster formation energy of the cluster with N atoms and the calculation method is as follows[20-21]:
| $E_{\mathrm{p}, \mathrm{C}}=(1 / N) \sum\limits_{i=1}^N\left(E_{\mathrm{p}, i}-E_{\mathrm{ref}, \alpha}\right)$ | (1) |
Ep, i represents the potential energy of the i-th atom and Eref, α represents the reference energy of the type α atom. The reference energy is the crystal chemical PE (hcp Zr, fcc Cu and fcc Al).
2 Results and Discussion 2.1 Glass TransitionFig. 1 represents variation in potential energy(PE) of ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys with the temperature during cooling. The PE basically changes linearly with the temperature, and there is a turning point between the temperature of 700 K and 800 K, indicating the occurrence of glass transition. The glass transition temperatures (Tg) of these alloys were obtained by linear fitting PE curves from 1200 K to 800 K and from 700 K to 300 K. Tg of Zr50Cu40Al10 alloy obtained in the experiment is 670 K-679 K[22], which is roughly consistent with the simulation result. As the cooling rate of simulation is much faster than that of the experiment (the cooling rate of experiment is generally lower than 106K/s), Tg obtained in the simulations is generally higher than the value obtained in the experiments. Fig. 2 shows variation of Tg with the Zr composition. It can be seen that Tg tends to increase first and then decrease with Zr composition increasing. When the Zr composition is 30%, Tg reaches the maximum value and the alloy owns high GFA, which is consistent with the results of relevant literature[10-12].
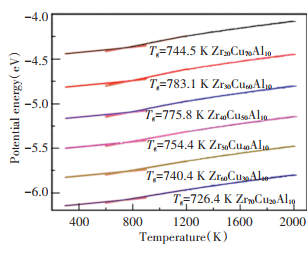
|
Fig.1 Variation of the average potential energy (PE) of ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys |

|
Fig.2 Variation of Tg in ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys |
2.2 Pair Distribution Functions
Fig. 3 shows the pair distribution functions of ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) amorphous alloys at 300 K. The curves are shifted vertically for better comparison. It can be seen that the first peak of each curve exhibits short-range order. When the Cu composition is higher than the Zr composition, the first peak splits. With the increase of Zr composition, the splitting phenomenon becomes less and less obvious. When the composition of Zr is higher than the composition of Cu, the splitting phenomenon disappears. In addition, with the increase of Zr composition, the first shell peak position shifts to the right.

|
Fig.3 Pair distribution functions (PDFs) for ZrxCu90-xAl10(x=20, 30, 40, 50, 60, 70) alloys |
Fig. 4 shows the partial PDFs of like atomic pairs and unlike atomic pairs. For better comparison, the curves are shifted vertically. As shown in the figure, the PDF curves of like atomic pairs are sensitive to the composition of the alloy. For the Zr-Zr partial PDF, with Zr composition increasing, the first peak strength increases. Partial PDFs for Cu-Cu atom pairs present strong short-range order when Cu composition is large. For the partial PDFs of unlike atomic pairs, the composition has little effect on the strength of their peaks.

|
Fig.4 Partial pair distribution functions (PPDFs) for ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys |
The first shell peak position and cut-off distance are extracted from the partial PDFs and are listed in Tables 1, 2, 3 and 4, which is basically consistent with the data obtained in the simulations conducted by Deb Nath[12].
| Table 1 The first shell peak position of the partial PDFs for the Zr-Zr, Cu-Cu, Al-Al atom pairs in ZrxCu90-xAl10(x=20, 30, 40, 50, 60, 70) alloys at 300 K |
| Table 2 The first shell peak position of the partial PDFs in ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys at 300 K |
| Table 3 The first shell cut-off distance of the partial PDFs in ZrxCu90-xAl10(x=20, 30, 40, 50, 60, 70) alloys at 300 K |
| Table 4 The first shell cut-off distance of the partial PDFs in ZrxCu90-xAl10(x=20, 30, 40, 50, 60, 70) alloys at 300 K |
2.3 Short-Range Structure and Energy Analysis
Fig. 5 shows the fractions and energy of the Al, Cu and Zr-centered nearest shell coordination polyhedron. It is found that CN = 16 occupies the most fraction in the Zr20Cu70Al10, Zr30Cu60Al10 alloys, CN = 15 occupies the most fraction in the Zr40Cu50Al10, Zr50Cu40Al10 alloys and the coordination polyhedron number with CN=14 is the largest in the Zr60Cu30Al10 and Zr70Cu20Al10 alloys. As the Zr composition increases, the corresponding CN of the coordination polyhedron with the highest fraction in the alloy decreases. The energy distribution of the Zr-centered coordination polyhedron shows that as the CN increases, the energy of the coordination polyhedron declines and then climbs up. When CN reaches 16, the energy of the coordination polyhedron is the lowest. Dowling et al.[23-26] proposed that the decrease of the polyhedron with high coordination number stands for the increase of the atomic structures packing loosely. It is found that the proportion of the Cu-centered 12 coordination polyhedron increases and then decreases with the increase of Zr composition. As the Zr composition increases from 20% to 30%, the proportion of the Cu-centered 12 coordination polyhedron increases from 55% to 56%, while the fraction decreases to 12% as the Zr composition increases to 70%. By calculating the energy of the coordination polyhedron, the variation trend in the energy of Cu-centered coordination polyhedron is the same as that of the Zr-centered polyhedron. When CN reaches 12, the energy of the coordination polyhedron is the lowest. Different from the Zr-centered coordination polyhedron, the energy distribution of the Cu-centered polyhedron with CN=12 differs greatly from that of other polyhedron, which should be caused by the formation of stable icosahedral structure. Among the Al-centered coordination polyhedron, the proportion of the 12 coordination polyhedron is the highest. The energy distribution is similar with the Cu-centered polyhedron. The 12 coordination polyhedron have the minimum energy and are the most stable. In the Zr30Cu60Al10 alloy, there are more Zr-centered high-coordination polyhedron (especially the coordination polyhedron with CN=16), Al and Cu-centered 12 coordination polyhedron, forming more tightly packed and low-energy short-range structure. As a result, Zr30Cu60Al10 alloy owns higher GFA.

|
Fig.5 Fraction and energy distribution of the Zr-, Cu- and Al-centered coordination polyhedron with different coordination numbers of ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloys |
2.4 Medium-Range Structure and Energy Analysis
Researchers introduced three classical connected clusters to explain the splitting of sub-nearest neighbor peaks, i.e., the cluster connection sharing one atom, two atoms and three atoms[27-30]. The main peak of the sub-nearest neighbor is mainly derived from the connection with three sharing atoms, the splitting peak is principally derived from the connection sharing one atom, and the peak valley between the two peaks is mainly derived from the connection sharing two atoms[27-30]. It can be seen from the PDFs of Zr30Cu60Al10 and Zr60Cu30Al10 amorphous alloys that the second peak of PDF for Zr30Cu60Al10 amorphous alloy shifts to left in Fig. 6. The main peak of the second peak mainly results from the coordination polyhedron connection with three sharing atoms, revealing that the structure is closely concerned with the cluster connection sharing three atoms. The splitting of the second peak is mainly caused by the coordination polyhedron connection sharing one atom. For crystals, coordination polyhedrons are generally connected with one, two and four sharing atoms, and the cluster connection with two and four sharing atoms are the main connection mode in crystals. In the rapid cooling process, the formation of the cluster connection with two and four sharing atoms are inhibited. Therefore, the amount of the polyhedron connections with three sharing atoms can represent the GFA to a certain extent.

|
Fig.6 PDFs for Zr30Cu60Al10 and Zr60Cu30Al10 metallic glasses at 300 K and the decomposed second nearest neighbor atoms via 1-atom, 2-atom, 3-atom and 4-atom coordination polyhedron connections |
It can be seen from Fig. 7 that in the studied ZrxCu90-xAl10 (x=20, 30, 40, 50, 60, 70) alloy system, the number of connection sharing an atom is the largest, followed by the connection with three sharing atoms, and then the connection with two sharing atoms, and the number of the connection with four sharing atoms is the lowest. With Zr composition increasing, the amount of the connections sharing one atom and three atoms increases first and then decreases, while the amount of the connections with two and four sharing atoms decreases first and then increases. For the Zr30Cu60Al10 ternary alloy, the amount of connection with three sharing atoms is the highest and the amount of connection sharing two atoms is the lowest. Further calculating the different polyhedron connections with three sharing atoms with different central atoms, as shown in Fig. 8, as the Zr composition increases (Cu composition decrease), the amount of Zr-Al and Zr-Zr polyhedron connections sharing three atoms increases continuously, while the amount of the Cu-Al and Cu-Cu polyhedron sharing three atoms decreases continuously. The amount of Zr-Cu polyhedron connection with three sharing atoms increases first and then decreases, reaching the high value when the Zr composition is 30% and 40%. This change mainly contributes to that Zr30Cu60Al10 having the maximum amount of the connection with three sharing atoms.
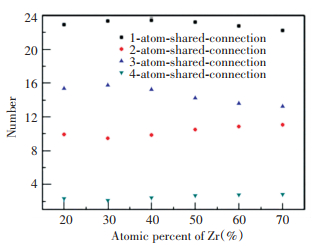
|
Fig.7 Average number of the coordination polyhedron connections with the number of shared atoms from one to four |
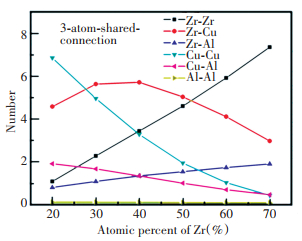
|
Fig.8 Change of the average number of the Zr-Zr, Zr-Cu, Zr-Al, Cu-Cu, Cu-Al, Al-Al coordination polyhedron connection with three shared atoms |
Fig. 9 shows energy distribution of the alloys with different compositions and connection modes. It can be seen that, regardless of the composition, the connection with three sharing atoms presents the lowest energy, followed by the connection sharing one atom, and then the connection with four sharing atoms, and the connection with two sharing atoms presents the highest energy. The energy landscape indicates that the amorphous alloy tends to form the coordination polyhedron connection with three sharing atoms. When Zr composition is 30% and 40%, the energy of different connections is the lowest. The amount of the connection with three sharing atoms is the most for the Zr30Cu60Al10 alloy. Therefore, the Zr30Cu60Al10 has more tightly connected, lower energy and more stable medium-range structure, owning higher GFA.
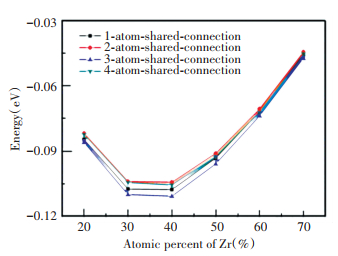
|
Fig.9 Energy of the coordination polyhedron connections with the number of shared atoms from one to four |
3 Conclusions
The results of the classical molecular dynamics simulations show that when the Zr composition is 30%, the glass transition temperature reaches the maximum value and the Zr30Cu60Al10 owns high glass forming ability. There are more Zr-centered polyhedron with high CN, Al and Cu-centered 12 coordination polyhedron and coordination polyhedron connections sharing three atoms in Zr30Cu60Al10 alloy. The existence of the stable structures has great impact on the high GFA of the Zr30Cu60Al10 alloy.
| [1] |
Hays C C, Kim C P, Johnson W L. Microstructure controlled shear band pattern formation and enhanced plasticity of bulk metallic glasses containing in situ formed ductile phase dendrite dispersions. Physical Review Letters, 2000, 84(13): 2901. DOI:10.1103/PhysRevLett.84.2901 (  0) 0) |
| [2] |
Bian Z, He G, Chen G L. Microstructure and mechanical properties of as-cast Zr52.5Cu17.9Ni14.6Al10Ti5 bulky glass alloy. Scripta Materialia, 2000, 43(11): 1003-1008. DOI:10.1016/S1359-6462(00)00524-8 (  0) 0) |
| [3] |
Vaidyanathan R, Dao M, Ravichandran G, et al. Study of mechanical deformation in bulk metallic glass through instrumented indentation. Acta Materialia, 2001, 49(18): 3781-3789. DOI:10.1016/S1359-6454(01)00263-4 (  0) 0) |
| [4] |
Ma E. Tuning order in disorder. Nature Materials, 2015, 14(6): 547-552. DOI:10.1038/nmat4300 (  0) 0) |
| [5] |
Hufnagel T C, Schuh C A, Falk M L. Deformation of metallic glasses: Recent developments in theory, simulations, and experiments. Acta Materialia, 2016, 109: 375-393. DOI:10.1016/j.actamat.2016.01.049 (  0) 0) |
| [6] |
Cheng Y Q, Ma E. Atomic-level structure and structure-property relationship in metallic glasses. Progress in Materials Science, 2011, 56(4): 379-473. DOI:10.1016/j.pmatsci.2010.12.002 (  0) 0) |
| [7] |
Liu Y H, Wang G, Wang R J, et al. Super plastic bulk metallic glasses at room temperature. Science, 2007, 315(5817): 1385-1388. DOI:10.1126/science.1136726 (  0) 0) |
| [8] |
Yu P, Chan K C, Chen W, et al. Elastic moduli and mechanical properties of bulk metallic glasses after quasi-static compression. Journal of Alloys and Compounds, 2011, 509(34): 8518-8521. DOI:10.1016/j.jallcom.2011.05.113 (  0) 0) |
| [9] |
Zhang L, Cheng Y Q, Cao A J, et al. Bulk metallic glasses with large plasticity: composition design from the structural perspective. Acta Materialia, 2009, 57(4): 1154-1164. DOI:10.1016/j.actamat.2008.11.002 (  0) 0) |
| [10] |
Wang C C, Wong C H. Structural properties of ZrxCu90-xAl10 metallic glasses investigated by molecular dynamics simulations. Journal of Alloys and Compounds, 2012, 510(1): 107-113. DOI:10.1016/j.jallcom.2011.07.110 (  0) 0) |
| [11] |
Yu C Y, Liu X J, Zheng G P, et al. Atomistic approach to predict the glass-forming ability in Zr-Cu-Al ternary metallic glasses. Journal of Alloys and Compounds, 2015, 627: 48-53. DOI:10.1016/j.jallcom.2014.12.023 (  0) 0) |
| [12] |
Deb Nath S K. Formation, microstructure and mechanical properties of ternary ZrxCu90-xAl10 metallic glasses. Journal of Non-Crystalline Solids, 2015, 409: 95-105. DOI:10.1016/j.jnoncrysol.2014.11.004 (  0) 0) |
| [13] |
Huang Y X, Li H, Wang C Z, et al. Ab initio molecular dynamics simulations of short-range order in Zr50Cu45Al5 and Cu50Zr45Al5 metallic glasses. Journal of Physics Condensed Matter An Institute of Physics Journal, 2016, 28(8): 085102. DOI:10.1088/0953-8984/28/8/085102 (  0) 0) |
| [14] |
Celtek M, Sengul S, Domekeli U. Glass formation and structural properties of Zr50Cu50-xAlx bulk metallic glasses investigated by molecular dynamics simulations. Intermetallics, 2017, 84: 62-73. DOI:10.1016/j.intermet.2017.01.001 (  0) 0) |
| [15] |
Zhao J F, Tang Z, Kelton K F, et al. Evolution of the atomic structure of a supercooled Zr55Cu35Al10 liquid. Intermetallics, 2017, 82: 53-58. DOI:10.1016/j.intermet.2016.11.010 (  0) 0) |
| [16] |
Zhao J F, Liu C T, Inoue A, et al. Structural properties and energy analysis of ZrxCu92-xAl8 ternary metallic glasses. Computational Materials Science, 2017, 139: 260-265. DOI:10.1016/j.commatsci.2017.07.045 (  0) 0) |
| [17] |
Sha Z D, Pei Q X. Ab initio study on the electronic origin of glass-forming ability in the binary Cu-Zr and the ternary Cu-Zr-Al(Ag) metallic glasses. Journal of Alloys and Compounds: An Interdisciplinary Journal of Materials Science and Solid-state Chemistry and Physics, 2015, 619: 16-19. DOI:10.1016/j.jallcom.2014.09.010 (  0) 0) |
| [18] |
Wang P, Yang X H. Atomistic investigation of aging and rejuvenation in CuZr metallic glass under cyclic loading. Computational Materials Science, 2020, 185: 109965. DOI:10.1016/j.commatsci.2020.109965 (  0) 0) |
| [19] |
Cheng Y Q, Ma E, Sheng H W. Atomiclevel structure in multicomponent bulk metallic glass. Physical Review Letters, 2009, 102(24): 245501. DOI:10.1103/PhysRevLett.102.245501 (  0) 0) |
| [20] |
Wu S Q, Wang C Z, Hao S G, et al. Energetics of local clusters in Cu64.5Zr35.5 metallic liquid and glass. Applied Physics Letters, 2010, 97(2): 021901. DOI:10.1063/1.3464164 (  0) 0) |
| [21] |
Zhao J F, Inoue A, Liu C T, et al. Structural evolution and energy landscape of the clusters in Zr55Cu35Al10 metallic liquid and glass. Scripta Materialia, 2016, 117: 64-67. DOI:10.1016/j.scriptamat.2016.02.023 (  0) 0) |
| [22] |
Nagai H, Mamiya M, Okutani T. Thermophysical properties of Zr-Cu-Al metallic glasses during crystallization. Journal of Non-Crystalline Solids, 2011, 357(1): 126-131. DOI:10.1016/j.jnoncrysol.2010.09.078 (  0) 0) |
| [23] |
Dowling J E, Wald G. The biological function of vitamin-a acid. PNAS, 1960, 46(5): 587-608. DOI:10.1073/pnas.46.5.587 (  0) 0) |
| [24] |
Ma D, Stoica A D, Wang X L. Power-law scaling and fractal nature of medium-range order in metallic glasses. Nature Materials, 2009, 8(1): 30-34. DOI:10.1038/nmat2340 (  0) 0) |
| [25] |
Hirata A, Guan P, Fujita T, et al. Direct observation of local atomic order in a metallic glass. Nature Materials, 2011, 10(1): 28-33. DOI:10.1038/nmat2897 (  0) 0) |
| [26] |
Wang X H, Inoue A, Kong F L, et al. Influence of ejection temperature on structure and glass transition behavior for Zr-based rapidly quenched disordered alloys. Acta Materialia, 2016, 116: 370-381. DOI:10.1016/j.actamat.2016.06.049 (  0) 0) |
| [27] |
Sheng H W, Luo W K, Alamgir F M, et al. Atomic packing and short-to-medium-range order in metallic glasses. Nature, 2006, 439(7075): 419-425. DOI:10.1038/nature04421 (  0) 0) |
| [28] |
Miracle D B. A structural model for metallic glasses. Nature Materials, 2004, 3(10): 697-702. DOI:10.1038/nmat1219 (  0) 0) |
| [29] |
Bennett C H. Serially deposited amorphous aggregates of hard spheres. Journal of Applied Physics, 1972, 43(6): 2727-2734. DOI:10.1063/1.1661585 (  0) 0) |
| [30] |
Liu X J, X uY, Hui X, et al. Metallic liquids and glasses: atomic order and global packing. Physical Review Letters, 2010, 105(15): 155501. DOI:10.1103/PhysRevLett.105.155501 (  0) 0) |
 2023, Vol. 30
2023, Vol. 30


