High efficiency is strongly demanded by gas turbines to lower emissions of CO2. To enhance its efficiency, the temperature at the entrance of the turbine is elevated. Hence, the thermal loading at the turbine vane is higher, and the secondary losses of the flow happen to be the pertinent part of aerodynamic losses caused by the association between the horseshoe vortex (HV) and the powerful cross-flow at the endwall.
Slot injection to the vane pressure side showed enhanced endwall cooling effectiveness than with an axial injection[1]. The endwall cooling effectiveness revealed higher homogenous spreading when the swirling angles are positive in the coolant jet[2]. The effectiveness of film cooling (FCE) on the endwall with a flat shape revealed lower deviations in the case of contoured passage than that of the flat passage[3-4]. As the flow rates of film cooling are lower, the FCE became abruptly lower in the passage of the contracting contoured endwall. When the leakage flow enhances, heat transfer also enhances adjacent to the leading edge suction side.
A local heat transfer rise to the tune of 20% adjacent to the passage junction on the pressure side and a 3% fall in heat transfer on an overall basis is reported for contoured endwalls[5]. The turbomachinery blading efficiency is enhanced for 2D airfoils by tangential endwall contouring, though the efficiency of the first stage interfaces suppresses the positive effects onto the downstream blade row, the endwall contouring is still beneficial for the overall performance of the engine[6]. The contouring could lower the losses due to secondary flow productively with the passage location getting the most benefit and with upstream airfoil region, getting the least[7]. Further, the contoured endwall affects the profile losses as well. The unfavorable pressure gradient has been lowered principally through the configuration of the groove adjacent to the suction surface leading edge concerning the suppression of corner separation[8]. The results of both the numerical as well as experimental work are utilized to designate the maneuvering of the metric as a substitute for the performance and as a quantity of design for the endwall design[9].
The axisymmetric endwall of the contoured geometry of a nozzle guide vane (NGV) showed the bottom-level heat transfer[4, 10] and the thermal exchange enhancement lowered with an elevation in the leakage flow because of the concentration of the HV. The Endwall of the first NGV is a critical portion due to the terrible operation conditions, complicated three-dimensional flow filed, and highly exposed area[11]. To protect the endwall from thermal ablation and limit the depletion of compressor bleed air, highly efficient endwall cooling systems are inevitable.
To meet low NOx emission requirements, diffusive combustion is changed to premixed combustion which makes the turbine inlet temperature flatter[12]. Since the pressure as well as vortex gradients are cumbersome, the area adjacent to the pressure side, leading and trailing edges was hard to cool[13]. The regions of enhanced static pressure with film injections having an elevated momentum flux ratio had the prospect to lower the crossflow intensity at the endwall and provided coolant to these zones[14-15].
A fall in local hot spot regions adjacent to the suction side and a fall in the heat transfer coefficient (HTC) obtained on an area averaging for the endwall are found utilizing for coolant blowing especially for the contoured cases than the flat ones[16]. The film cooling and thermal exchange studies were mostly reported in a linear cascade[17]. The difference in cooling effectiveness was found more than 10% between annular and linear cascades[18]. Nusselt number (Nu) measurement was carried out on the contoured and flat hub of the endwall passages[19]. Ice formation method was used and obtained heat transfer reduction on the designed endwall[20].
Inlet temperature enhancement in a gas turbine is a productive approach to augmenting its output power and thermal efficiency[21]. The structures of the secondary flow adjacent to the end wall affect huge complexities of the thermal ambiance and its interaction with the flow and protection of endwall cooling[22]. The endwalls of the turbine cascade are cooled by purge flow by sealing the interface gaps between the turbine and combustor or the stator and rotor[23]. The impact of the purge flow is restricted to the bottom half of the channel height and the optimized design can lower the vorticity adjacent to the casing[24]. Endwall contouring lower the gross pressure loss coefficient of the cascade and outstandingly elevate the productive purge flow distribution region near the suction side[25].
Endwall contouring modifies the spreading of static pressure on it by modifying the endwall surface curvature and leads to aerodynamic benefits[26-27]. The impact of optimization of endwall contouring in outlet transonic conditions was studied and found that the area-averaged HTC calculated on an area-averaging basis is lowered by 10%[28]. The HTC of an endwall contoured by the ice formation method was investigated and observed to alter the vortex movements as well as flow fields[29].
The FCE got reduced by the endwall contouring[30]. In the case of endwalls having enclosed holes for film cooling, the endwall contouring could enhance the cooling effectiveness at an elevated mass flux ratio (MFR)[31]. The endwall of a contoured transonic blade could lower its thermal load, by the cooling action of leakage flows upstream[32]. When gross losses for a vane with a narrow aspect ratio are considered, the secondary losses contribute nearly 30%-50%. HV enhances the thermal exchange in the leading-edge location highly and results in a hot spot whenever film cooling cannot cool sufficiently[33].
All the optimized endwall designs of contoured geometry identified are having recessed areas at the passage central region and an upward area at the passage aft region adjacent to the suction side. A superior thermal exchange performance case attributed to an enhanced HTC calculated on a mean area basis with a smaller total endwall area[34]. A double coolant temperature model could precisely forecast endwall heat load and film cooling spread, and phantom cooling spread of the vane surface[35]. A suitable contouring amplitude could remarkably lower the endwall heat load with no significant impact aerodynamically[36]. The FCE of holes placed discretely resulted in enhanced coverage of coolant at the location of stagnation having a high angle of contouring[37]. The top important areas concerning the aerodynamic efficiency of turbine passage are at its mid-pitch from front to aft part subjected to acceleration characteristics of the stream[38]. The endwall design of a rotor cascade is investigated by limiting the disparity adjacent to the hub and shroud separately and simultaneously in an axisymmetric manner. The control locations of the Bezier curve are used as parameters on the surface of an endwall[39].
In this study, shape changes are determined at which endwall regions have the highest pertinent influence on thermal exchange characteristics of endwall or aerodynamic performance of passage. Multi-objective optimization approach design of passages of endwall in a non-axisymmetric manner of ideal characteristics developed by the outcomes of this study is further investigated to comprehend in-depth, why transposition of shape in these most prominent locations transforms significantly the aero-thermal characteristics. The contouring in a non-axisymmetric endwall with the existence of purge flow becomes the pivot of this paper, with significance on the implementation of numerical methods to the configuration of unique endwalls. The present study reduces the endwall heat load of the vane and improves the overall efficiency of the turbine. The work involved the design of a Reynolds number, with coolant blowing, with an upstream slot, and without coolant blowing conditions. Optimum contouring shape and perturbation heights to span concerning non-axisymmetry are adjudged with stage efficiency, output torque, and pressure loss comparisons. The net reduction in heat flux, Nu, the effectiveness of Phantom cooling, and aerodynamic performance through total pressure loss coefficients are reported and discussed.
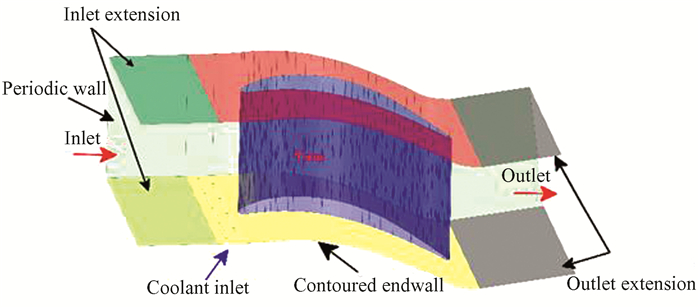
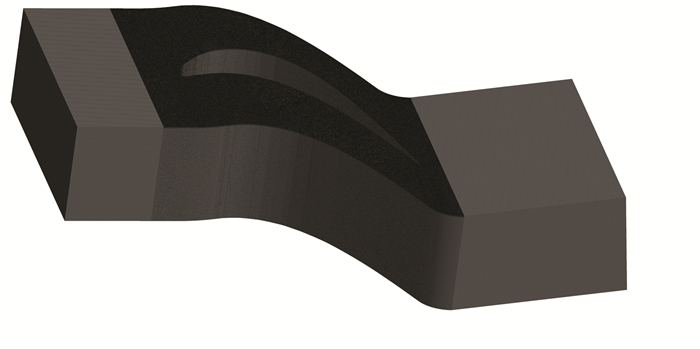
1 Computational Setup 1.1 Computational Model and MeshTwo different endwall shapes viz. flat and contoured (axisymmetric convergent) designed for the first NGV were investigated in this study. The computational domain is the transonic vane cascade (16% inlet turbulence, 1.7×106 outlet Re and 0.85 outlet Mach number). Cax is the length of axial separation of the leading and trailing edges of the vane. The upstream purge flow is considered by the 42 cylindrical cooling double-row holes with a diameter of 2.4 mm which are located at 0.4 Cax upstream of the center passage vane leading edge. Figs. 1 and 2 give the computational domain as well as the mesh in respect of the axisymmetric convergent contoured endwall. Periodic conditions were assigned over the boundary towards the pitch-ward direction. Meshes of structured nature were created through Integrated Computer-aided Engineering and Manufacturing (ICEM) CFD with low Re meshes with nodes of 5.5×106 for the axisymmetric convergent contoured endwall vane passage, and 9×106 for the coolant holes as well as the plenum. For the locations of the boundary layer of the wall, meshes were concentrated using a height of 1.5×10-3 mm for the first cell and a 1.15 expansion factor to reproduce the flow evolution. The y+ value was maintained lower than 0.8 on the endwall. FCE spread around the vane leading edge on the contoured endwall with 6×106, 9×106, 12×106, and 15×106 grid points were compared at 2.5% blowing ratio (BR). The FCE value of 0.48 was unchanged when the grid number increased from 12×106 to 15×106. The mesh size of 12× 106 provides a grid-independent endwall FCE.
|
Fig.1 Schematic diagram of the linear vane cascade |
|
Fig.2 Mesh details of the contoured vane end-wall |
1.2 Solver and Turbulence Models
The numerical predictions were carried out for the steady-state RANS, using ANSYS FLUENT. Pressure-velocity coupling, a realizable k-ε turbulence model with compressibility, viscous heating effects, curvature correction, and production limiter was used. The k-ε turbulence model was employed by several authors[40-41] and recommended as an excellent option. Instead, Du[42] used the SST turbulence model considering the identical Nozzle Guide Vane concerning that of in Ref.[41], with no upgradation of the outcome. The Realizable k-ε turbulence model alongside the augmented wall function has been hence used being demonstrated as excellent in comparison with the standard wall function as well as similar ones. Endwall thermal exchange enhancement along the vane passage for the realizable k-ε turbulence model in respect of contouring in a non-axisymmetric manner registered concurrence with the measured values[30]. Further, the experimental and simulated film cooling values employing the realizable k-ε turbulence model stipulated that the contouring in a non-axisymmetric manner regulates the flow distribution of film-cooling over the endwall relying on the association of the contour geometry as well as the film. The realizable k-ε turbulence model alongside the augmented wall function has been used and compressibility effects, curvature correction, viscous heating effects, and production limiter were accounted for in this model[35]. The realizable k-ε model may be more appropriate for the endwall contouring of a turbine with a no-slip wall, as it is well-suited for predicting turbulent flows near walls[30]. Air was used with a constant turbulent Prandtl number of 0.95.
1.3 Boundary ConditionsThe Mass Flux Ratio is:
| $ \operatorname{MFR}=\frac{\rho_c v_c}{\rho_m v_m} $ | (1) |
where the ρc and ρm are the density of coolant and mainstream vc and vm are the velocity of coolant and mainstream. The passage entrance of the vane in the computational domain was detected at 1.2 Cax upstream of its leading edge. Different coolant flow rates were set at the coolant inlet depending on MFR = 0.5%-2.5%. At the computational model's inlet; total pressure, total temperature, and mass flow; and at the exit, constant mean pressure was provided. Conditions of adiabatic and no-slip wall boundary had been assigned in respect of the holes as well as the plenum of coolant. The coolant temperatures were set as 298 and 295 K.
1.4 Optimum Contoured Endwall ProfilingThe axisymmetric deviation adjacent to the shroud, hub, and endwall surfaces is confined and parameterized adopting the Latin Hypercube sampling statistics with BP ANN (NSGA-Ⅱ) algorithm for optimization. The perturbation height percentage to the span of 9, 12, and 15 are obtained concerning conditions of contouring of endwalls based on the shroud and hub separately as well as both hub and shroud simultaneously using a 7th-order Bezier curve function[43] to restrict radial deviation of the control spots of endwall. The perturbation height limit to span is expressed as the proportion of fluctuation height to blade chord in percent. The convexity endwall possesses positive height and the concavity represents negative height. H shape refers to the hub and has a convex area (enhancing radius) at the surface of the endwall in respect of the hub in proximity to the blade pressure surface and an area of concavity (diminishing radius) adjacent to the vane suction surface. S shape refers to the shroud endwall and has an area of concavity (enhancing radius) adjacent to the vane suction surface with a convex area adjacent to the mid-passage (diminishing radius) adjacent to the suction surface. In the case of HS shape, the contouring is enforced on both hub and shroud surfaces at the same time. Its curvature is smaller than the H and S shapes and its shroud area is adjacent to the suction surface downstream of the passage.
1.5 Heat Transfer and Film Cooling Prediction MethodologyThe forecast of the effectiveness of film cooling was carried out using the model of walls of the adiabatic and isothermal vane and endwall concepts and two coolant temperatures. Different numerical simulations were performed at a range of MFR cases using various boundary conditions in respect of endwall and vane surfaces. The near endwall film flow temperatures are equal to the adiabatic wall temperatures. A uniform wall temperature of 300 K was applied and the adiabatic wall temperatures, Taw,c1 and Taw,c2 at the first and second coolant temperatures, respectively along with the wall heat flux are obtained. The thermal exchange attributes for the generated endwall contours were analyzed for the Reynolds number (Re) of making viz., 200000. The HTC hc, and the effectiveness of adiabatic film cooling η in respect of the endwall are found utilizing the following equations:
| $ \mathrm{Nu}=\frac{h_c \cdot C}{k} $ | (2) |
| $ \eta=\frac{T_{a w, c 1}-T_{a w, c 2}}{T_{c 1}-T_{c 2}} $ | (3) |
The present method reduces the numerical prediction error of cooling effectiveness caused by the approximation of the local mainstream recovery temperature and avoids its cumbersome determination.
1.6 Numerical Method and ValidationA comparison with the experiment measurements[35] was provided at MFR 2.5% along the middle pitch for axisymmetric convergent contoured endwall Nu distributions (Table 1). An enhanced thermal exchange is not well forecasted due to the impact of the HV suction side leg. A deterministic unsteadiness might also contribute to the measured turbulent kinetic energy since the HV has a bimodal nature. This concentrated turbulent kinetic energy might have resulted in the gradients of heat transfer. The numerical predictions in general show a good agreement with measurements in trend, the magnitude has differences concerning upstream locations of the vane leading edge (x < 0). The maximum prediction error is less than 19% in the whole vane passage (0 < x/Cax < 0.65 Cax) and less than 1.24% downstream of the vane passage throat (x > 0.65 Cax). This prediction error might be caused by the surface roughness in the original measurements, (but treated as a smooth one in simulations) as well as the insufficient prediction of endwall secondary flows. In general, the present numerical method is reliable and can be used to investigate the impacts of axisymmetric convergent contouring and BR on film cooling as well as the associated phantom cooling distributions on endwall, turbine stage efficiency, heat transfer and aerodynamic performances of the vane pressure side. Several authors reported the existence of maximum prediction error in a contoured endwall to the tune of 20% and an extreme deviation, especially in locations having elevated skew (> 70 degrees) as well as adverse gradients of pressure downstream of HV separation and reattaching flow due to adequately capturing the physics of complex flow[35].
| Table 1 Comparison of Nu distribution along middle pitch of axisymmetric convergent contoured endwall at MFR 2.5% with that of Bai et al., 2022[35] |
2 Results and Discussion 2.1 Optimum Endwall Profiles
The spread of variation in height of axisymmetric endwalls, after optimization in a non-axisymmetric way, yielded different optimal profiles of endwall. A height of positive value denotes a convex contoured endwall, accelerating the flow and a height of negative value denotes a concave contoured endwall, decelerating the flow. The deviations in height of different sign values result in a lower deviation in the flow area. A geometrical difference exists concerning the rise and fall of the surfaces of the hub and shroud linked to the channel of the flow. Radial height on hub and shroud surfaces are higher and lower respectively than its radius. Similar changes in the radius are found at the concavity as well.
When non-axisymmetric contouring is attempted on the endwall of a gas turbine, a specific shape of "S" is resulted, as convex and concave areas are assigned adjacent to the pressure and suction surfaces respectively. The results of the optimization of a hydraulic turbine are different from the above. The original axisymmetric endwall has a zero-perturbation height percent to span (F0) and the changes with 3% and 6% perturbation heights to span are too small. A fresh Hub 9% (H9) possesses a convexity area (radius enhancement) from the entrance to the exit on the surface of the endwall at the hub (9% perturbation height to span) adjacent to the vane pressure side, and possesses a concave area (diminishing radius) adjacent to the vane suction side, stretching till its trailing edge. A fresh Shroud 9% (S9) possesses a concave area (radius enhancement) on it adjacent to the vane suction side. In contrast, an apparent convex area (diminishing radius) develops adjacent to the passage center of the cascade, elongating towards the stream direction along the suction surface till the trailing edge. A fresh Hub-Shroud 9% (HS9) possesses contouring in a non-axisymmetric manner with both faces together. The endwall curvature of HS9 is smaller than that of H9 and S9. The concave and convex spread of the HS9 are almost opposite to H9 and S9 except for the shroud area of HS9 which is adjacent to the downstream of passage at its suction surface. However, comparing H9 and H12, and S9 and S12, the perturbation height spread is not equal, but the location is uniformly inverted like the height spread at the upstream passage flow adjacent to the pressure surface of S9 and S12. Such events commence on H12 and H15, and S12 and S15. As the radial fluctuation limits of the endwall control variables of the endwall increase, their concavity and convexity heights do not rise consistently. This might be due to the balancing process of optimization for enhancement accomplishment of all the entities earmarked, rather than the linear functional association among the variables as well as the single objective function. An optimal fluctuation height range exists every time.
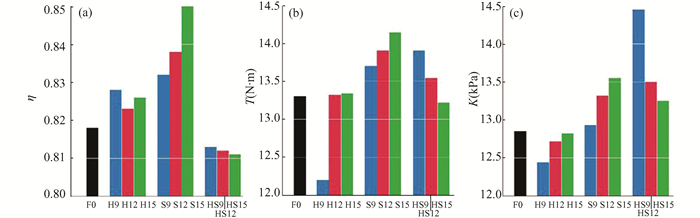
The performance attributes of all endwall profiles are depicted in Fig. 3(a), 3(b), and 3(c), where ηts denotes the efficiency of a turbine stage, T denotes the output torque of the single stage and K denotes gross loss in pressure. The optimum non-axisymmetric endwalls were developed to an enhancement in stage efficiency of the turbine. The optimization of endwall in respect of non-axisymmetric shroud leads to marked enhanced stage efficiency and output torque when the perturbation height varies from 9% to 15% with the outstanding performance enhancement of 3.2% in efficiency and 0.84 N·m in torque for S15 than F0, but with a total pressure loss enhancement by 0.7 also (Fig. 3). As the perturbation height rises from 9% to 15%, the endwall optimization in respect of the non-axisymmetric shroud and hub together leads to a sustained fall in efficiency and a non-optimistic fall in total pressure loss. The endwall optimization in respect of the non-axisymmetric hub though leads to little improvements in efficiency, a fall in output torque also is evident. The endwall optimization in respect of the non-axisymmetric shroud is efficient to enhance the extensive performance attainment of the turbine as evident for S15 in Fig. 4, but great attention is necessary to contour the hub and shroud endwalls simultaneously.
|
Fig.3 Turbine stage efficiency (a), output torque (b), pressure loss(c) for different endwall shapes and perturbation heights to span |

|
Fig.4 Non-axisymmetric Shroud optimized contoured endwall with 15% high perturbation |
2.2 Phantom Cooling Effectiveness
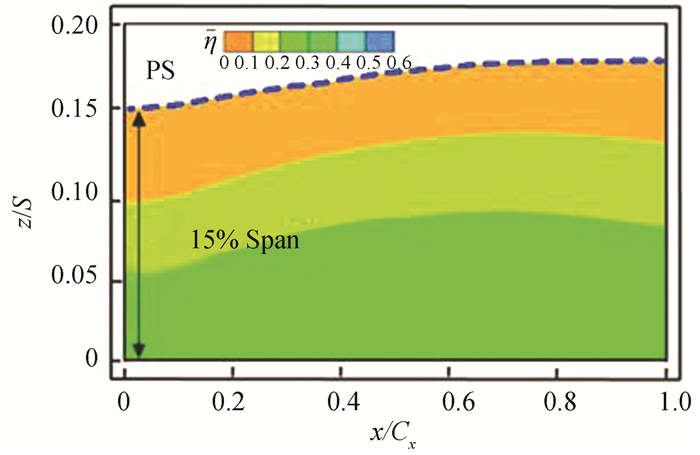
For perfect film cooling performance, the phantom cooling effectiveness has a maximum value of one, (same as coolant hole outlet temperature) while a value of zero indicates that the film cooling has not reduced the wall temperature. z is the spanwise coordinate (mm) and S is the spanwise height of the vane (m) or longitudinal coordinate beside the curve. Figs. 5 and 6 show contoured endwalls for 15% perturbation height to span (S15) and flat endwalls respectively at a BR of 3.5% and illustrates the effectiveness of the local phantom cooling at the pressure side surface of the vane. For BR = 1.0 case, there is nearly no phantom cooling at the pressure side surface of the vane, because of the insufficient momentum of the coolant flow. For BR = 2.5 and BR=3.5 cases, the pressure side surface of the vane shows a good phantom cooling effectiveness level of 0.3-0.6 for axisymmetric convergent contoured endwall, especially for the location adjacent to the leading edge of the vane. The phantom cooling effectiveness level is low (less than 0.3) in the pressure side surface of the vane for the flat endwall, and the effectiveness of phantom cooling is more homogenous from the leading to the trailing edge of the vane. In addition, the location of phantom cooling of the pressure side surface of the vane increases by nearly 100% (up to 15% span) at BR = 3.5, due to the suppression impacts of the HV for contoured convergent axisymmetric endwall and high momentum of the coolant. For axisymmetric convergent contoured endwall, the region of phantom cooling increases approximately 100% (up to 15% span) at BR = 3.5, and the level of phantom cooling effectiveness is 0.3-0.6 on the pressure side surface of the vane. This suggests that the depletion of coolant protecting the vane surface from thermal ablation can be decreased by introducing an axisymmetric convergent contoured endwall. The optimum endwall contouring shapes can simplify the vane cooling structure and decrease the number of film holes located on the root of the vane pressure side surface. At the aft location, the movement of coolant is much more delicate to lift off from the suction surface of the vane, but unable to recombine to the surface of the platform due to the secondary flow migration from the pressure surface to the suction surface. This leads to either the absence or weaker phantom cooling due to injection from the suction side on the surface of the platform.

|
Fig.5 Phantom cooling effectiveness for vane pressure side surface of S15 contoured endwall at BR 3.5 |
|
Fig.6 Phantom cooling effectiveness for vane pressure side surface of flat endwall at BR 3.5 |
2.3 Pitch-Averaged Endwall Nusselt Number
To quantify the endwall heat transfer, the pitch-averaged values of endwall Nu for flat and contoured endwall shapes at BR 1.0-3.5 is shown in Fig. 7. The axisymmetric convergent contouring slightly enhances endwall thermal load in the whole vane passage(0 < x < 0.65Cx), and the maximum enhancement (about 20%) is observed at high BR=3.5. Here, Cx represents the axial chord length of the blade. In addition, the axisymmetric convergent contouring significantly decreases endwall thermal load in the location downstream of the vane passage throat (x > 0.65Cx), and the maximum decrease (up to 50%) is observed at a low blowing ratio of BR=0.5. As the BR increases from 1.0 to 2.5 (Fig. 7), the endwall heat transfer increases for both two endwall contouring shapes in the location upstream of the vane passage(x < 0.4Cx), but it decreases in the region downstream of the vane passage throat (x > 0.65Cx). As the BR increases from 2.5 to 3.5, only a slight enhancement is observed in the location upstream of the vane passage (x < 0.4Cx).

|
Fig.7 Pitch-averaged endwall Nu for flat and contoured endwall shapes at BR 1.0-3.5 |
The contouring of convergent axisymmetric endwall generates a low elevation (about 20%) of thermal exchange in the whole passage of the vane (0 < x < 0.65Cx) and a significant decrease (up to 50%) in the region downstream of the vane passage throat (x > 0.65Cx). The flow conditions of blowing ratio have significant effects on endwall thermal load distributions, particularly in locations upstream of the passage of the vane (x < 0.4Cx), vane pressure surface corner, and downstream of the vane passage throat (x > 0.65Cx).
2.4 Effect of Endwall Contouring 2.4.1 Effect of endwall contouring with upstream slot and without coolant blowingThe entering boundary layer bisect at the vane leading edge stagnation point upstream and develops an HV in a flat endwall. The contoured endwall redirects the pressure side leg of the HV in the direction of the stream.
The HV pressure side leg progress far interior of the passage in a flat endwall and develops in solidity upon interaction with the mainstream. The pressure differential of the cross-passage developed among the vane pressure side with the nearby vane suction side operates the passage vortex leading to the airfoil suction side, and there it joins with the HV suction side leg and is taken away from the endwall in a flat endwall. The protruded contoured endwall region of the vane leading edge proceeding towards the suction side prevents the passage vortex from meeting over the passage when the pressure gradient is large. Hence the passage vortex keeps weaker and unable to develop from the mainstream in solidity and the pressure differential impact of the cross passage is minimal in the contoured endwall.
The corner vortex attaches and proceeds to get larger through the vane suction side till the trailing edge in a flat endwall and finally attaches with it. A peak field controls the passage vortex through the airfoil pressure side and presses the union spot with the HV suction side leg farther in a contoured endwall (Fig. 8). The trackway and solidity of the passage vortex decide the entrainment of hot gas towards the endwall and have a high impact on heat transfer. Local Nu are sustained on flow characteristics adjacent to the endwall and enhanced Nu is evident in regions of powerful vortices.

|
Fig.8 Vortex structure without coolant blowing |
2.4.2 Effect of endwall contouring with coolant injection
Figs. 9 and 10 show endwall Nu spread for the cases of film-cooling with a coolant to mainstream MFR=1.5% for flat (Fig. 9) and contoured (Fig. 10) endwall geometries. The interaction among the coolant and secondary flow structures is noticed as significant for the geometries of flat and contoured endwalls evident on Nu spread. The upstream slot of leakage flow abides by the trackway completed among the HV pressure side leg and the suction side of the nearby airfoil and is subsequently moved out to the mainstream direction. The penetration of the coolant jet as well as the diffusion into the passage flow of the mainstream enhanced with enhancement in coolant MFRs from 0.5% to 1.5%. A local zone of enhanced heat transfer (Fig. 9) is evident at the airfoil upstream adjacent to the chamfer region for flat endwall with MFR 1.5%. This zone is greatly influenced by turbulent dissipation as well as the merging of the coolant jet with the mainstream. As the MFR and momentum flux of the coolant rises, the dissipation rate also gets elevated and the powerful interaction among mainstream and coolant flow becomes powerful and the zone adjacent to the coolant outlet becomes intense and the zone enlarges with elevated heat transfer. Bolstering of the slot-prompted coolant vortex generates still higher momentum flux to the coolant is also resulted from the elevated HTC of this zone. The coolant vortex is a powerful type of cavity vortex described before because of the inclusion of coolant. Elevation of the endwall-specific thermal load because of the coolant jet merging is evident in contoured geometry with the MFR 0.5% and 1.0%. But the zone is migrated further downstream than in the flat endwall. An elevated flow rate of coolant markedly lowers the thermal load adjacent to the upstream location and the leading edge for the contoured endwall with an MFR of 1.5%.

|
Fig.9 Contour plot of endwall Nu distribution for film-cooling with coolant to mainstream MFR = 1.5% for flat endwall |

|
Fig.10 Contour plot of endwall Nu distribution for film-cooling with coolant to mainstream MFR = 1.5% for contoured endwall |
At elevated MFR, superior cooling is achieved adjacent to the region of stagnation of the leading edge. The coolant spread in the direction of the mainstream is not elevated much for MFR 1.5% in comparison with 1.0% because of the coolant lift-off from the endwall. The Nu contours for MFR 1.5% depicted in Fig. 10 with extremely elevated zones of heat transfer corroborates this behavior and might be because of higher turbulence amalgamation of coolant and mainstream. In the case of the contoured endwall, the spread of coolant film is much more elevated over the airfoil suction side in the streamwise direction than the flat endwall for elevated coolant MFR. This phenomenon might be because of the adherence of the coolant with the endwall by the impact of contouring at the passage downstream with not much influence of the secondary flow. At 1.5% MFR, the flat endwall has slightly elevated effectiveness at the leading-edge forepart as shown in Figs. 9 and 10. But the contoured endwall has markedly elevated effectiveness at the leading-edge forepart at 0.5% and 1.0% MFR.
2.5 Net Heat Flux ReductionThe net heat flux reduction (NHFR) denotes an absolute appraisal of the endwall thermal load and is estimated using the FCE and HTC as follows:
| $ \text { NHFR }=1-h / h_0(1-\eta \phi) $ | (4) |
| $ \phi=T_{\infty}-T_c / T_{\infty}-T_w $ | (5) |
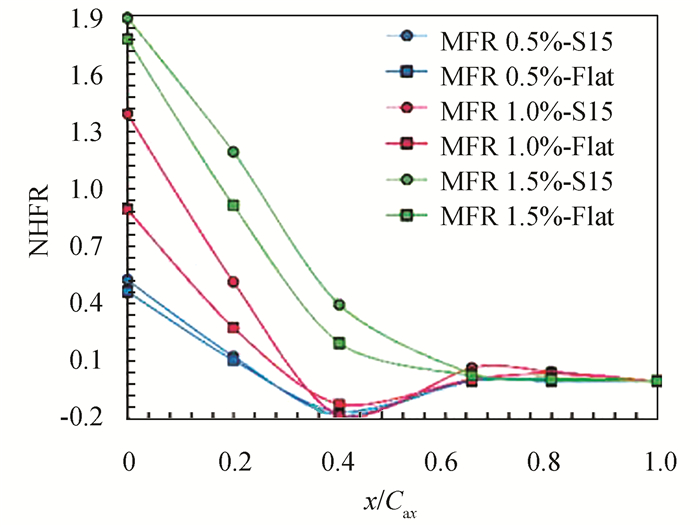
where ϕ represents the effectiveness of cooling on an overall basis denoted by non-dimensional metal temperature. For a turbine vane with film cooling, its value is set as 1.6[44]. An elevated NHFR explains a lower heat load on the endwall. A contoured endwall significantly augments the endwall NHFR with MFR > 1.0% leading to a lower endwall heat load than a flat endwall. At MFR 0.5% the NHFR has the main gain from the lowering of heat transfer than the augmentation in film cooling and hence the NHFR enhancement is not much beneficial. At MFR 1.0%, an endwall with a contoured geometry considerably enhances the NHFR at x/Cax < 0.65 with a still elevated value upstream. A 1.5% contouring amplitude achieves the highest NHFR throughout the endwall and might be due to augmenting the FCE and lowering the rates of heat transfer. At MFR 1.5% the contoured endwall with a contoured geometry continuously augments the NHFR at x/Cax < 0.65. A 1.0% contouring amplitude achieves the highest NHFR at most locations of the endwall. An escalated contouring amplitude alternatively lowers the NHFR on the front of the endwall.
Adiabatic cooling effectiveness is more on endwall with contoured geometry than that with flat geometry at similar MFR. Since the contoured endwall has a wide area for cooling, the enhanced cooling performance benefit in the passage vanishes. But the impact of effectiveness enhancement in adiabatic cooling is higher, compared to the area change, leading to a lower endwall net heat flux in comparison to that in the passage, when MFR > 1.625%.
The marked augmentation in NHFR at the endwall of contoured geometry (Fig. 11) is the result of lowering the vigor of the passage vortex (by effectively controlling the secondary flow) and flow gradients of the cross-passage; as well as the coolant film capacity in piercing the passage farther till the trailing edge at elevated MFR. The pressure side NHFR is mainly the result of local HTC fall. The impact of the coolant film spread is commanding towards the suction side of the platform and to the stagnation zone of the slot downstream. An enhanced overall Nu is found at MFR 0.5%-1.0% with the contoured endwall, marked augmentation in NHFR can be acquired since the FCE is elevated than the flat endwall. At 1.5% MFR, the performance of a contoured geometry is higher as coolant film adheres with the surface by contouring and in the case of flat geometry, jet lift-off takes place. A contoured geometry considerably augments the endwall NHFR (averaged crosswise at the direction of length) at MFR >1.0% (Fig. 11). Hence, a contoured geometry can lower the endwall heat load than a flat geometry.
|
Fig.11 Comparison of NHFR in a flat and S15 endwalls with MFR 0.5%-1.5% |
2.6 Aerodynamic Performance
Total pressure loss coefficients (TPLC) with coolant blowing are:
| $ \text { TPLC }=\frac{\frac{\dot{m}_{\mathrm{c}}}{\dot{m}_{\mathrm{c}}+\dot{m}_{\mathrm{m}}} P_{\mathrm{t}, \mathrm{cin}}+\frac{\dot{m}_{\mathrm{c}}}{\dot{m}+\dot{m}_{\mathrm{m}}} P_{\mathrm{t}, \mathrm{m}}-P_{\mathrm{t}, \mathrm{f}}}{P_{\mathrm{t}, \mathrm{m}}-P_{\mathrm{f}}} $ | (6) |
Coolant mass flow rate is denoted

|
Fig.12 TPLC with MFR 0-2.5% at x/Cax = 1.25 in the passage of flat and contoured endwalls |
3 Conclusions
Turbine vane endwall with an axisymmetric contoured geometry were subjected to computational simulations and the results were analyzed concerning the endwall outlet flow field, thermal exchange, and FCE. The optimization of contouring profiles of the endwall revealed a lower coolant distribution throughout. Endwall contouring shifted the HV farther from the passage vortex, delayed the passage vortex evolution, and increased thermal exchange all over the saddle point. The endwall boundary layer is not much affected by this tiny passage vortex emanated, that reduced thermal exchange in the passage. The passage aft location, entrance boundary layer segregation downstream, the flow field around the upstream cavity in the HV development, and the location of the vortices in respect of the secondary flow away from the endwall need to be explored in designing endwall contours. Revamping the heat transfer forecast of the endwall seems to be the upcoming prime obstacle in enhancing durability and axial turbine efficiency by way of ideal thermal and aerodynamic designs.
| [1] |
Du K, Song L, Li J, et al. Effects of the mainstream turbulence intensity and slot injection angle on the endwall cooling and phantom cooling of the vane suction side surface. International Journal of Heat and Mass Transfer, 2017, 112: 427-440. DOI:10.1016/j.ijheatmasstransfer.2017.05.010 (  0) 0) |
| [2] |
Zhang L J, Moon H K. Comparison of two axisymmetric profiles on blade platform film cooling. Heat Transfer, Parts A and B, 2011, 5: 11-20. DOI:10.1115/GT2011-45102 (  0) 0) |
| [3] |
Thrift A A, Thole K A, Hada S. Effects of an axisymmetric contoured endwall on a nozzle vane: Adiabatic effectiveness measurements. Proceedings of the ASME Turbo Expo 2010: Power for Land, Sea, and Air. Volume 4: Heat Transfer, Parts A and B. Glasgow, UK. 2010, 43994: 1617-1627. DOI: 10.1115/GT2010-22968
(  0) 0) |
| [4] |
Thrift A A, Thole K A, Hada S. Effects of an axisymmetric contoured endwall on a nozzle guide vane: Convective heat transfer measurements. Journal of Turbomachinery, 2011, 133(4): 041007. DOI:10.1115/1.40029665 (  0) 0) |
| [5] |
Lynch S P, Thole K A, Kohli A, et al. Computational predictions of heat transfer and film-cooling for a turbine blade with nonaxisymmetric endwall contouring. Journal of Turbomachinery, 2011, 133(4): 041003. DOI:10.1115/1.4002951 (  0) 0) |
| [6] |
Zimmermann T W, Curkovic O, Wirsum M, et al. Comparison of two-dimensional and three-dimensional turbine airfoils in combination with nonaxisymmetric endwall contouring. Journal of Turbomachinery, 2017, 139(6): 061007. DOI:10.1115/1.4035274 (  0) 0) |
| [7] |
Duan W, Qiao W, Wei Z, et al. The influence of different endwall contouring locations on the secondary flow losses in a highly loaded low pressure turbine. Proceedings of ASME Turbo Expo - Turbomachinery Technical Conference and Exposition. New York: ASME, 2018. GT2018-76159. DOI: 10.1115/GT2018-76159.
(  0) 0) |
| [8] |
Li X, Jin D, Gui X, et al. Effect of solidity on non-axisymmetric endwall contouring performance in compressor linear cascades. Turbomachinery, 2018, 2A: 50992. DOI:10.1115/GT2018-76247 (  0) 0) |
| [9] |
Bergh J, Snedden G. Investigation of a novel flow metric for the design and evaluation of non-axisymmetric turbine endwall contours preliminary computational and experimental investigation. Proceedings of the 25th International Society of Air-Breathing Engines Conference (ISABE 2022). Ottawa, Canada. 2022.296. https://www.researchgate.net/publication/359209384
(  0) 0) |
| [10] |
Thrift A A, Thole K A, Hada S. Effects of an axisymmetric contoured endwall on a nozzle guide vane: Adiabatic effectiveness measurements. Journal of Turbomachinery, 2011, 133(4): 041007. DOI:10.1115/1.4002965 (  0) 0) |
| [11] |
Han J C. Fundamental gas turbine heat transfer. Journal of Thermal Science and Engineering Applications, 2016, 5(2): 021007. DOI:10.1115/1.4023826 (  0) 0) |
| [12] |
Bahr D W. Gas Turbine Combustion: Alternative Fuels and Emissions. 3rd Ed. New York: CRC Press, Taylor and Francis Group, 2010.1-557. DOI: 10.1201/9781420086058.
(  0) 0) |
| [13] |
Thomas M, Kirollos B, Jackson D, et al. Experimental and CFD studies of NGV endwall cooling. Proceedings of the ASME Turbo Expo 2013: Turbine Technical Conference and Exposition. Volume 3B: Heat Transfer. San Antonio, Texas: ASME, 2013. GT2013-95639, V03BT13A056. DOI: 10.1115/GT2013-95639.
(  0) 0) |
| [14] |
Li S J, Lee J, Han J C. Turbine platform cooling and blade suction surface phantom cooling from simulated swirl purge flow. Journal of Turbomachinery, 2016, 138(8): 081004. DOI:10.1115/1.4032676 (  0) 0) |
| [15] |
Wang L, Li X, Ren J, et al. The interaction between upstream and downstream film cooling rows in flow field and heat transfer. International Journal of Thermal Sciences, 2020, 149: 106176. DOI:10.1016/j.ijthermalsci.2019.106176 (  0) 0) |
| [16] |
Roy A, Jain S, Ekkad S V, et al. Heat transfer performance of a transonic turbine blade passage in the presence of leakage flow through upstream slot and mateface gap with endwall contouring. Journal of Turbomachinery, 2017, 139(12): 121006. DOI:10.1115/1.4037909 (  0) 0) |
| [17] |
El-Batsh H M. Effect of the radial pressure gradient on the secondary flow generated in an annular turbine cascade. International Journal of Rotating Machinery, 2012, 2012: 1-14. DOI:10.1155/2012/509209 (  0) 0) |
| [18] |
Yao R, Wang J, Yang W, et al. A numerical investigation on the differences between annular and flat film cooled endwalls. Proceedings of the ASME Turbo Expo 2017: Turbomachinery Technical Conference and Exposition, American Society of Mechanical Engineers. Volume 5A: Heat Transfer. Charlotte, North Carolina: ASME, 2017. V05AT12A006-V05AT12A006. DOI: 10.1115/GT2017-63337.
(  0) 0) |
| [19] |
Lynch S P, Sundaram N, Thole K A, et al. Heat transfer for a turbine blade with nonaxisymmetric endwall contouring. Journal of Turbomachinery, 2011, 133(1): 011019. DOI:10.1115/1.4000542 (  0) 0) |
| [20] |
Winkler S. Endwall Contouring Using Numerical Optimization in Combination with the Ice Formation Method. Stuttgart: University of Stuttgart, 2016. DOI: 10.18419/opus-8819.
(  0) 0) |
| [21] |
Han J C. Advanced cooling in gas turbines 2016 max Jakob memorial award paper. ASME Journal of Heat Transfer, 2018, 140(11): 113001. DOI:10.1115/1.4039644 (  0) 0) |
| [22] |
Papa M, Srinivasan V, Goldstein R J. Film cooling effect of rotor-stator purge flow on endwall heat/mass transfer. Journal of Turbomachinery, 2012, 134(4): 041014. DOI:10.1115/1.4003725 (  0) 0) |
| [23] |
Schreiner B, Wilson M, Li Y S. Effect of purge on the secondary flow-field of a gas turbine blade-row. Journal of Turbomachinery, 2020, 142: 101006. DOI:10.1115/1.4047185 (  0) 0) |
| [24] |
Schaflein L, Janssen J, Brandies H, et al. Influence of purge flow injection on the performance of an axial turbine with three-dimensional airfoils and non-axisymmetric endwall contouring. Journal of Turbomachinery, 2023, 145(6): 061004. DOI:10.1115/1.4056238 (  0) 0) |
| [25] |
Du K, Jia Y, Song H, et al. Effect of slot jet flow on non-axisymmetric endwall cooling performance of high-load turbines. Machines, 2023, 11(2): 134. DOI:10.3390/machines11020134 (  0) 0) |
| [26] |
Guo Z, Bu H, Song L, et al. Experimental test of a 3D parameterized vane cascade with non-axisymmetric endwall. Aerospace Science and Technology, 2019, 85: 429-442. DOI:10.1016/j.ast.2018.12.021 (  0) 0) |
| [27] |
Bergh J, Snedden G, Dunn D. Optimization of non-axisymmetric endwall contours for the rotor of a low speed, 1 1/2-stage research turbine with unshrouded blades-optimization and experimental validation. Journal of Turbomachinery, 2020, 142(4): 041006. DOI:10.1115/1.4045988 (  0) 0) |
| [28] |
Panchal K V, Abraham S, Roy A, et al. Effect of endwall contouring on a transonic turbine blade passage: Heat transfer performance. Journal of Turbomachinery, 2017, 139: 011009. DOI:10.1115/1.4034411 (  0) 0) |
| [29] |
Winkler S, Weigand B, Ligrani P. Flow structure and surface heat transfer from a turbine component endwall contoured using the ice formation method. International Journal of Heat and Mass Transfer, 2018, 120: 895-908. DOI:10.1016/j.ijheatmasstransfer.2017.12.028 (  0) 0) |
| [30] |
Lynch S P. The effect of endwall contouring on boundary layer development in a turbine blade passage. Virginia: Virginia Polytechnic Institute and State University, 2011. http://hdl.handle.net/10919/77202.
(  0) 0) |
| [31] |
Chen P, Wang L, Li X, et al. Effect of axial turbine non-axisymmetric endwall contouring on film cooling at different locations. International Journal of Heat and Mass Transfer, 2020, 147: 118995. DOI:10.1016/j.ijheatmasstransfer.2019.118995 (  0) 0) |
| [32] |
Roy A, Blot D M, Ekkad S V, et al. Thermal management of a transonic turbine: Leakage flow and endwall contouring effects. Journal of Thermophysics and Heat Transfer, 2018, 32: 1031-1044. DOI:10.2514/1.T5340 (  0) 0) |
| [33] |
Satta F, Tanda G. Measurement of local heat transfer coefficient on the endwall of a turbine blade cascade by liquid crystal thermography. Experimental Thermal and Fluid Science, 2014, 58: 209-215. DOI:10.1016/j.expthermflusci.2014.07.005 (  0) 0) |
| [34] |
Chen P, Zhao K, Li X, et al. Effects of varying non-axisymmetric contours of the turbine endwall on aerodynamics and heat transfer Aspects: A sensitivity analysis study. International Journal of Thermal Sciences, 2021, 161: 106689. DOI:10.1016/j.ijthermalsci.2020.106689 (  0) 0) |
| [35] |
Bai B, Li Z, Li J, et al. The effects of axisymmetric convergent contouring and blowing ratio on endwall film cooling and vane pressure side surface phantom cooling performance. Journal of Engineering for Gas Turbines and Power, 2022, 144: 021020. DOI:10.1115/1.4052500 (  0) 0) |
| [36] |
Tao Z, Yu B, Li Y, et al. Effects of non-axisymmetric endwall contouring on aerothermal performance of a gas turbine blade endwall with a purge flow. International Journal of Thermal Sciences, 2021, 164: 106921. DOI:10.1016/j.ijthermalsci.2021.106921 (  0) 0) |
| [37] |
Hussain S, Liu J, Wang L, et al. Suppression of endwall heat transfer in the junction region with a symmetric airfoil by a vortex generator pair. International Journal of Thermal Sciences, 2019, 136: 135-147. DOI:10.1016/j.ijthermalsci.2018.10.019 (  0) 0) |
| [38] |
Chen P, Li X, Ren J, et al. Multi-objective optimization of non-axisymmetric contoured endwall for axial turbines. International Journal of Gas Turbine, Propulsion and Power Systems, 2021, 12(1): 1-9. DOI:10.38036/jgpp.12.1_1 (  0) 0) |
| [39] |
Meng L, Zhang X D, Yan G, et al. Investigation of improving the hydraulic turbine cascade performance using non-axisymmetric endwall contouring. Advances in Mechanical Engineering, 2020, 12(4): 1-18. DOI:10.1177/1687814020915410 (  0) 0) |
| [40] |
Knost D G. Prediction and measurement of film cooling on the endwall of a first stage vane. Blacksburg: Virginia Polytechnic Institute and State University, 2003. https://vtechworks.lib.vt.edu/bitstream/handle/10919/35186/dknost_Thesis.pdf?sequence=1.
(  0) 0) |
| [41] |
Hada S, Thole K A. Computational study of a midpassage gap and upstream slot on vane endwall film-cooling. Journal of Turbomachinery, 2011, 133: 011024. DOI:10.1115/1.4001135 (  0) 0) |
| [42] |
Du K, Li J. Numerical study on the effects of slot injection configuration and endwall alignment mode on the film cooling performance of vane endwall. International Journal of Heat and Mass Transfer, 2016, 98: 768-777. DOI:10.1016/j.ijheatmasstransfer.2016.02.014 (  0) 0) |
| [43] |
Zhao G J. Improving the performance of turbine based on a new optimization design method. Journal of Propulsion Technology, 2014, 35: 597-602. (  0) 0) |
| [44] |
Lynch S P, Thole K A. Heat transfer and film cooling on a contoured blade endwall with platform gap leakage. Journal of Turbomachinery, 2017, 139: 051002. DOI:10.1115/1.4035202 (  0) 0) |
 2024, Vol. 31
2024, Vol. 31


